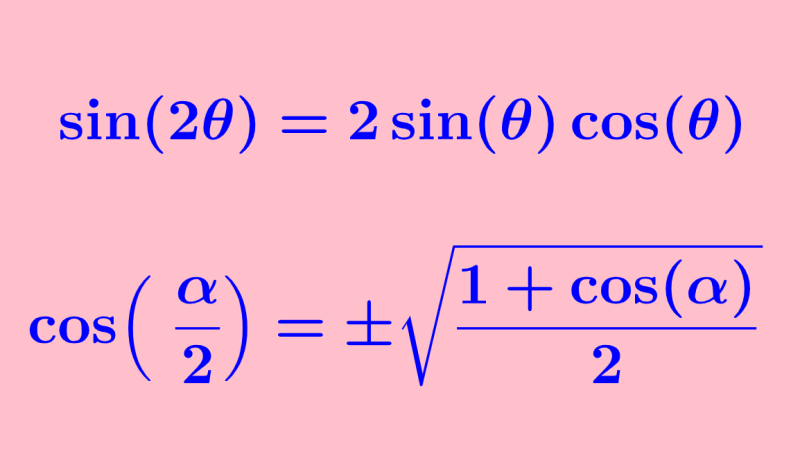Formulas for the Trigonometric Identities

Formulas of reciprocal identities

A reciprocal of a fraction is defined as a fraction that has the numerator and denominator with switched positions. The reciprocal identities are defined with respect to the fundamental trigonometric functions, sine, cosine, and tangent.

Each fundamental trigonometric function produces a reciprocal identity. For example, the sine function is defined as the opposite side divided by the hypotenuse, that is, $latex \sin(\theta)=\frac{O}{H}$.

The cosecant function is the reciprocal of the sine, so we have $latex \csc(\theta) = \frac{H}{O}$. If we have $latex \sin (\theta) = \frac{1}{2}$, then we also have $latex \csc(\theta) = 2$.

Similarly, the secant function is the reciprocal of the cosine and the cotangent function is the reciprocal of the tangent. Therefore, we have the formulas:

$latex \csc(\theta)=\frac{1}{\sin(\theta)}$ $latex \sec(\theta)=\frac{1}{\cos(\theta)}$ $latex \cot(\theta)=\frac{1}{\tan(\theta)}$

---

Formulas of Pythagorean identities

Pythagorean identities are derived from the Pythagorean theorem. In a unit circle, the x-coordinates correspond to cosine and the y-coordinates correspond to sine. In addition, the unit radius corresponds to the hypotenuse, so we have:

$latex {{\sin}^2} (\theta)+{{\cos}^2} (\theta)=1$

This is the main Pythagorean identity. From this identity, we can divide it by sine or cosine to obtain the following variations:

$latex {{\tan}^2} (\theta)+1={{\sec}^2} (\theta)$ $latex 1+{{\cot}^2} (\theta)={{\csc}^2} (\theta)$

In these identities, “tan” represents the tangent, “sec” represents the secant, “cot” represents the cotangent, and “csc” represents the cosecant.

---

Formulas of quotient identities

Quotient identities are formulas that relate the tangent and the cotangent in terms of sine and cosine. The sine function is defined as the opposite side divided by the hypotenuse and the cosine function is defined as the adjacent side divided by the hypotenuse.

These definitions can be used to check the following formulas for the quotient identities:

$latex \tan(\theta)=\frac{\sin(\theta)}{\cos(\theta)}$ $latex \cot(\theta)=\frac{\cos(\theta)}{\sin(\theta)}$

---

Formulas of negative angle identities

Negative angle identities relate the trigonometric functions of a negative angle to trigonometric expressions of a positive angle. These identities use the definitions of the even and odd functions. Therefore, we have:

$latex \sin(-\theta)=-\sin(\theta)$ $latex \cos(-\theta)=\sin(\theta)$ $latex \tan(-\theta)=-\tan(\theta)$ $latex \cot(-\theta)=-\cot(\theta)$ $latex \sec(-\theta)=\sec(\theta)$ $latex \csc(-\theta)=-\csc(\theta)$

---

Formulas of complementary angle identities

Complementary angle identities relate two trigonometric functions through complementary angles. Two angles are complementary when they add up to 90°. Therefore, to find a complementary angle, we subtract 90° from the original angle. The formulas for these identities are:

$latex \sin(90^{\circ} -\theta)=\cos(\theta)$ $latex \cos(90^{\circ} -\theta)=\sin(\theta)$ $latex \tan(90^{\circ} -\theta)=\cot(\theta)$ $latex \cot(90^{\circ} -\theta)=\tan(\theta)$ $latex \sec(90^{\circ} -\theta)=\csc(\theta)$ $latex \csc(90^{\circ} -\theta)=\sec(\theta)$

---

Formulas of sum and difference identities

The identities of sum and difference of angles are used mainly to find the exact values of an angle, which can be written as a sum or subtraction of common angles such as 30°, 45°, 60°, 90°, and their multiples. This is because the values of these angles are generally known.

The formulas for the identities of the sum of angles are:

The formulas for the identities of the difference of angles are:

---

Formulas of double angle identities

The double angle identities are derived from the angle sum identities. These identities are used when we know the value of θ and we have to find the value of sine, cosine, or tangent of 2θ.

The formula for the double angle identity for the sine is:

$latex \sin(2x)=2\sin(x)\cos(x)$

The double angle identity for the cosine has three variations, two of which are obtained by using the Pythagorean identity:

$latex \cos(2x)={{\cos}^2}(x)-{{\sin}^2}(x)$ $latex =2{{\cos}^2}(x)-1$$latex =1-2{{\sin}^2}(x)$

The formula for the double angle identity for the tangent is:

$latex \tan(2x)=\frac{2\tan(x)}{1-{{\tan}^2}(x)}$

---

Formulas of half-angle identities

The half-angle identities are derived using the double angle identities and substituting for $latex \frac{\theta}{2}$. These trigonometric identities are used when we know the value of sine, cosine, or tangent of θ and we want to find the value of $latex \frac{\theta}{2}$.

These identities can also help us transform trigonometric expressions that have exponents to one without exponents.

The formula for the identity of the half-angle of the sine is:

$latex \sin(\frac{\theta}{2})=\pm \sqrt{\frac{1-\cos(\theta)}{2}}$

The formula for the identity of the half-angle of the cosine is:

$latex \cos(\frac{\theta}{2})=\pm \sqrt{\frac{1+\cos(\theta)}{2}}$

The formula for the tangent half-angle identity is:

$latex \tan(\frac{\theta}{2})=\frac{\sin(\theta)}{1+\cos(\theta)}$ $latex =\frac{1-\cos(\theta)}{\sin(\theta)}$

---

See also

Interested in learning more about trigonometric identities? Take a look at these pages:

• Reciprocal Identities – Formulas and examples
• Quotient Identities – Formulas and Examples
• Pythagorean Identities – Formulas, Proof and Examples
• Fundamental Trigonometric Identities