Trigonometric identities are equations, which are true for any angle used. Trigonometric identities are used to rewrite trigonometric expressions and simplify or solve them. These identities are derived from the fundamental trigonometric functions, sine, cosine, and tangent. Also, the unit circle and the Pythagorean theorem are used to obtain more identities.
Here, we will learn about the formulas of the fundamental trigonometric identities. Then, we will use these identities to solve some practice problems.
TRIGONOMETRY
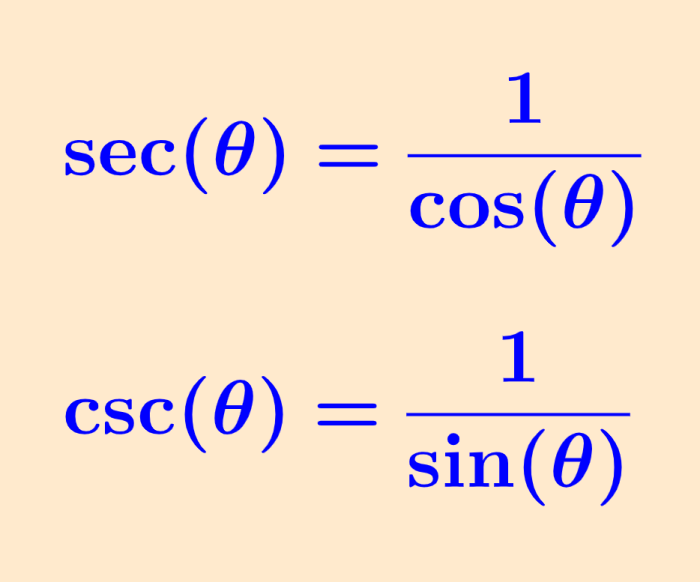
Relevant for…
Learning about the fundamental trigonometric identities with examples.
TRIGONOMETRY
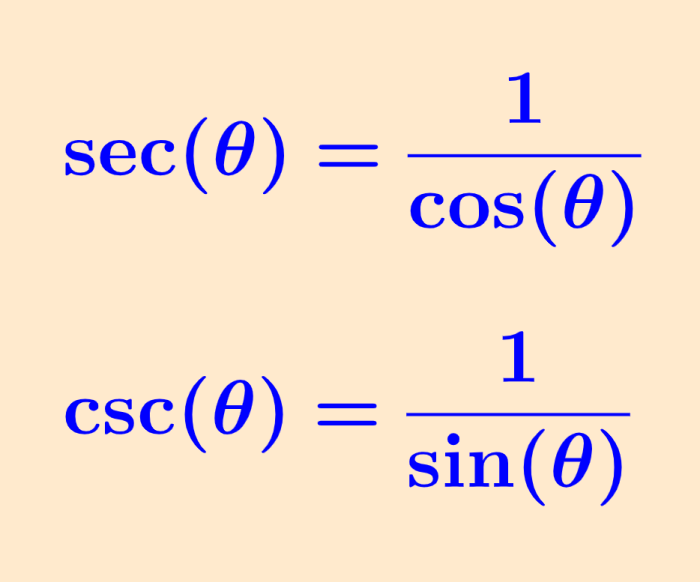
Relevant for…
Learning about the fundamental trigonometric identities with examples.
What are the fundamental trigonometric identities?
There are several trigonometric identities that can be derived from the definitions of trigonometric functions. Also, the unit circle and the Pythagorean theorem are used to obtain more identities.
Finally, the first trigonometric identities are used to derive and obtain variations of trigonometric identities that can be applied in other situations.
This means that there are several formulas for trigonometric identities, however, the most important are reciprocal identities, quotient identities, complementary angle identities, negative angle identities, and Pythagorean identities.
Reciprocal identities
These identities are defined from the trigonometric functions sine, cosine and tangent. A reciprocal of a fraction is equal to the numerator and denominator swapped in position. We can form a reciprocal by writing one over the original quantity. Therefore, we define the cosecant, secant and cotangent functions:
$latex \csc(\theta)=\frac{1}{\sin(\theta)}$
$latex \sec(\theta)=\frac{1}{\cos(\theta)}$
$latex \cot(\theta)=\frac{1}{\tan(\theta)}$
Quotient identities
These identities are found by writing the tangent and the cotangent in terms of the sine and the cosine. Therefore, we have:
$latex \tan(\theta)=\frac{\sin(\theta)}{\cos(\theta)}$
$latex \cot(\theta)=\frac{\cos(\theta)}{\sin(\theta)}$
Complementary angle identities
Complementary angles are angles that add up to 90°. We can use these angles to define trigonometric identities that relate to trigonometric functions:
$latex \sin(\theta)=\cos(90^{\circ} -\theta)$
$latex \cos(\theta)=\sin(90^{\circ} -\theta)$
$latex \tan(\theta)=\cot(90^{\circ} -\theta)$
$latex \cot(\theta)=\tan(90^{\circ} -\theta)$
Negative angle identities
Using the definitions of even and odd functions, we can find identities for negative angles and write them as positive angles.
$latex \sin(-\theta)=-\sin(\theta)$
$latex \cos(-\theta)=\cos(\theta)$
$latex \tan(-\theta)=-\tan(\theta)$
Pythagorean identities
The first Pythagorean identity is derived using the Pythagorean theorem on a unit circle. The other two variations are found by dividing the main Pythagorean identity by sine or cosine and simplifying.
$latex {{\sin}^2}(\theta)+{{\cos}^2}(\theta)=1$
$latex {{\tan}^2}(\theta)+1={{\sec}^2}(\theta)$
$latex {{\cot}^2}(\theta)+1={{\csc}^2}(\theta)$
Fundamental trigonometric identities – Examples with answers
The following examples are solved by applying the fundamental trigonometric identities seen above. Each example has its respective solution, where you can observe the process used.
EXAMPLE 1
Use the Pythagorean identities to simplify the trigonometric expression $latex \sin(x){{\cos}^2}(x)-\sin(x)$.
Solution
We observe that the expression has the sine and the cosine. This means that we can use the identity $latex {{\sin}^2}(x) + {{\cos}^2} (x) = 1$. However, we have to start by factoring the given expression:
$$\sin(x){{\cos}^2}(x)-\sin(x)=\sin(x)({{\cos}^2}(x)-1)$$
Also, we are going to rewrite the identity $latex {{\sin}^2}(x)+{{\cos}^2}(x)=1$ in the following way: $latex {{\cos}^2}(x)-1=-{{\sin}^2}(x)$ and we substitute it in the identity to get:
$$\sin(x)({{\cos}^2}(x)-1)=\sin(x)(-{{\sin}^2}(x))$$
$latex ={{\sin}^3}(x)$
EXAMPLE 2
Calculate the value of $latex \tan(\theta)$ using the values $latex \cos(\theta)=\frac{3}{11}$ and $latex \sin(\theta)=\frac{5}{11}$.
Solution
In this case, we can use the identity of the quotient of the tangent and write it in terms of sine and cosine. Therefore, the value of the tangent is:
$latex \tan(\theta)=\frac{\sin(\theta)}{\cos(\theta)}$
$latex \tan(\theta)=\frac{\frac{5}{11}}{\frac{3}{11}}$
$latex \tan(\theta)=\frac{5}{3}$
EXAMPLE 3
Determine the value of $latex \tan(\theta)$ using $latex \cot(\theta)=\frac{5}{3}$.
Solution
We can solve this exercise using the reciprocal identity of the tangent. The cotangent is the reciprocal function of the tangent, so we find the value of the tangent by “flipping” the value of the cotangent. Therefore, we have:
$latex \cot(\theta)=\frac{5}{3}$
⇒ $latex \tan(\theta)=\frac{3}{5}$
EXAMPLE 4
Use the trigonometric identities to simplify the expression: $$({{\sec}^2}(x))(1-{{\sin}^2}(x))-(\frac{\sin(x)}{\csc(x)}+\frac{\cos(x)}{\sec(x)})$$
Solution
We can start by using the reciprocal identities $latex \sec(x)=\frac{1}{\cos(x)}$ and $latex \csc(x)=\frac{1}{\sin(x)}$. Therefore, we have:
$$({{\sec}^2}(x))(1-{{\sin}^2}(x))-(\frac{\sin(x)}{\csc(x)}+\frac{\cos(x)}{\sec(x)})$$
$$=({{\sec}^2}(x))(1-{{\sin}^2}(x))-({{\sin}^2}(x)+{{\cos}^2}(x))$$
Now, we are going to use the main Pythagorean identity to completely solve:
$latex =({{\sec}^2}(x))({{\cos}^2}(x))-(1)$
$latex =1-1$
$latex =0$
Fundamental trigonometric identities – Practice problems
Use the formulas for the fundamental trigonometric identities seen above to solve the following problems. Select an answer and click “Check” to check that you got the correct answer.
See also
Interested in learning more about trigonometric identities? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
