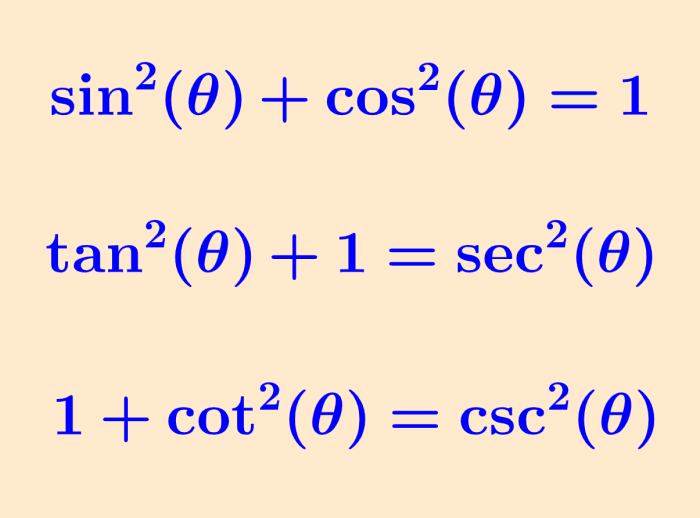Trigonometric identities are equations that are true for any angle used. These identities are used to rewrite and simplify complex trigonometric expressions. Furthermore, we can also use trigonometric identities to solve trigonometric expressions by rewriting them as more manageable expressions or as additions and subtractions of known angles.
Here, we will learn to solve trigonometric identities problems. We will use various techniques to get the answer.
How to solve trigonometric identities problems?
To solve trigonometric identities exercises, we have to start by carefully observing the type of exercise we have. Some exercises will ask us directly to apply an identity type to calculate the angle values.
For example, the identities of sum and difference of angles, the identities of half-angles or double angles are used to calculate the values of an angle that can be written as the addition, subtraction, double angle, or half-angle of “easy” angles such as 30°, 45°, 60° or 90°.
However, many of the exercises will require us to verify that other more complex trigonometric identities are true.
There is no standard method for verifying identities, but there are some general rules we can follow to make the process easier:
1. Try to simplify the more complicated side of the identity until it is identical to the other side of the identity.
2. Try to transform both sides of the identity to an identical expression.
3. Try to express both sides of the identity only in terms of sine and cosine. Then, try to make both sides equal.
4. Apply Pythagorean identities whenever possible.
5. Try factoring and combining terms, multiplying on one side of the identity by an expression that equals 1, squaring both sides of the identity, and other algebraic techniques to manipulate equations.
If you need to reinforce your knowledge of trigonometric identities, see our summary with the formulas of the most important identities.
Trigonometric identities – Problems with answers
The trigonometric identities are applied to solve the following problems. Observe the solution of the exercises and analyze the process and reasoning used. Try to solve the exercises yourself if possible.
PROBLEM 1
Find the value of $latex \cot(\theta)$ if we have $latex \cos (\theta) = \frac{5}{7}$ and $latex \sin(\theta) = \frac{2}{7}$.
Solution
We can use the identity of the quotient of the cotangent. Therefore, we know that we have to divide the value of the cosine by the value of the sine to find the value of the tangent:
$latex \cot(\theta)=\frac{\cos(\theta)}{\sin(\theta)}$
$latex \cot(\theta)=\frac{\frac{5}{7}}{\frac{2}{7}}$
$latex \cot(\theta)=\frac{5}{2}$
PROBLEM 2
Determine the value of $latex \tan (\theta)$ if we have $latex \cot (\theta) = \frac{9}{4}$.
Solution
In this case, we can use the reciprocal identity of the tangent. This means that we find the value of the tangent by “flipping” the fraction of the value of the cotangent. Therefore, we have:
$latex \cot(\theta)=\frac{9}{4}$
⇒ $latex \tan(\theta)=\frac{4}{9}$
PROBLEM 3
Apply the trigonometric identities to simplify the expression $latex \sin(x){{\cos}^2}(x) – \sin(x)$.
Solution
We can apply the Pythagorean identity $latex {{\sin}^2} (x) + {{\cos}^2}(x) = 1$. For this, we have to factor the given expression:
$$\sin(x){{\cos}^2}(x)-\sin(x)=\sin(x)({{\cos}^2}(x)-1)$$
If we reorganize the identity $latex {{\sin}^2}(x)+{{\cos}^2}(x)=1$, we have $latex {{\cos}^2}(x)-1=-{{\sin}^2}(x)$. Using this variation, we have:
$$\sin(x)({{\cos}^2}(x)-1)=\sin(x)(-{{\sin}^2}(x))$$
$latex ={{\sin}^3}(x)$
PROBLEM 4
Apply the identity of the sum of angles to determine the exact value of the cosine of 75°.
Solution
To use the identity of the sum of angles, we note that 75° is equal to the sum of 30° and 45°. Therefore, we have:
$$ \cos(A+B)=\cos(A)\cos(B)-\sin(A)\sin(B)$$
$$\cos(30+45)=\cos(30)\cos(45)-\sin(30)\sin(45)$$
$latex =\frac{\sqrt{3}}{2} \cdot \frac{\sqrt{2}}{2}-\frac{1}{2}\cdot \frac{\sqrt{2}}{2}$
$latex =\frac{\sqrt{6}}{4}-\frac{\sqrt{2}}{4}$
$latex =\frac{\sqrt{6}-\sqrt{2}}{4}$
The value of the cosine of 75° is $latex \frac{\sqrt{6}-\sqrt{2}}{4}$.
PROBLEM 5
If we have $latex \sin(A) = \frac{2}{9}$, determine the value of $latex \cos (2A)$.
Solution
We can apply the double angle identity for the cosine. We use a variation of the formula for the double cosine angle, which is obtained using the Pythagorean identity. Thus, we have:
$latex \cos(2A)=1-2{{\sin}^2}(A)$
$latex \cos(2A)=1-2{{(\frac{2}{9})}^2}$
$latex \cos(2A)=1-2(\frac{4}{81})$
$latex \cos(2A)=1-\frac{8}{81}$
$latex \cos(2A)=\frac{73}{81}$
PROBLEM 6
Check that the identity $latex \cos(A) + \sin(A) \tan (A) = \sec (A)$ is true.
Solution
We can verify this identity by rewriting it until we get the same expression on both sides. We can start by using the identity of the quotient of the tangent:
$latex \cos(A)+\sin(A)\tan(A)=\sec(A)$
$latex \cos(A)+\sin(A)(\frac{\sin(A)}{\cos(A)})=\sec(A)$
Now, we can add the expressions on the left side to get a single expression:
$latex \cos(A)+\sin(A)(\frac{\sin(A)}{\cos(A)})=\sec(A)$
$latex (\frac{{{\cos}^2}(A)+{{\sin}^2}(A)}{\cos(A)})=\sec(A)$
Now, we use the Pythagorean identity to simplify the numerator:
$latex (\frac{{{\cos}^2}(A)+{{\sin}^2}(A)}{\cos(A)})=\sec(A)$
$latex (\frac{1}{\cos(A)})=\sec(A)$
We know that the secant is the reciprocal identity of the cosine, so we have:
$latex (\frac{1}{\cos(A)})=\sec(A)$
$latex \sec(A)=\sec(A)$
We have verified that the identity is true.
PROBLEM 7
Determine the cosine and tangent values if we have $latex \sin(A) = \frac{7}{8}$ and if $latex \cos(A)<0$.
Solution
We can find the value of the cosine using the Pythagorean identity:
$latex {{\cos}^2}(A)+{{\sin}^2}(A)=1$
$latex {{\cos}^2}(A)+{{(\frac{7}{8})}^2}=1$
$latex {{\cos}^2}(A)+\frac{49}{64}=1$
$latex {{\cos}^2}(A)=1-\frac{49}{64}$
$latex {{\cos}^2}(A)=\frac{15}{64}$
$latex \cos(A)=\pm \sqrt{\frac{15}{64}}$
$latex \cos(A)=- \frac{\sqrt{15}}{8}$
We take the negative value since we have $latex \cos (A) <0$. Now, we can use the identity of the quotient of the tangent to find its value using the sine and cosine values:
$latex \tan(A)= \frac{\sin(A)}{\cos(A)}$
$latex \tan(A)= \frac{\frac{7}{8}}{-\frac{\sqrt{15}}{8}}$
$latex \tan(A)= -\frac{7}{\sqrt{15}}$
$latex \tan(A)= -\frac{7\sqrt{15}}{15}$
Trigonometric identities – Practice problems
Apply the different trigonometric identities to solve the following problems. Select an answer and check it to see if you got the correct answer.
See also
Interested in learning more about trigonometric identities? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
