The transformation of functions is the changes that we can apply to a function to modify its graph. One of the important transformations is the reflection of functions. A function can be reflected over the x-axis when we have –f(x) and it can be reflected over the y-axis when we have f(-x).
Here, we will learn how to obtain a reflection of a function, both over the x-axis and over the y-axis. We will use examples to illustrate important ideas.
ALGEBRA
Relevant for…
Learning about the reflection of functions over the x-axis and y-axis.
ALGEBRA
Relevant for…
Learning about the reflection of functions over the x-axis and y-axis.
Reflecting a function over the x-axis and y-axis
The reflections of a function are transformations that make the graph of a function reflected over one of the axes. A reflection is equivalent to “flipping” the graph of the function using the axes as references.
We can understand this concept using the function $latex f(x)=x+1$. When we graph this function, we get the line shown in the following graph:
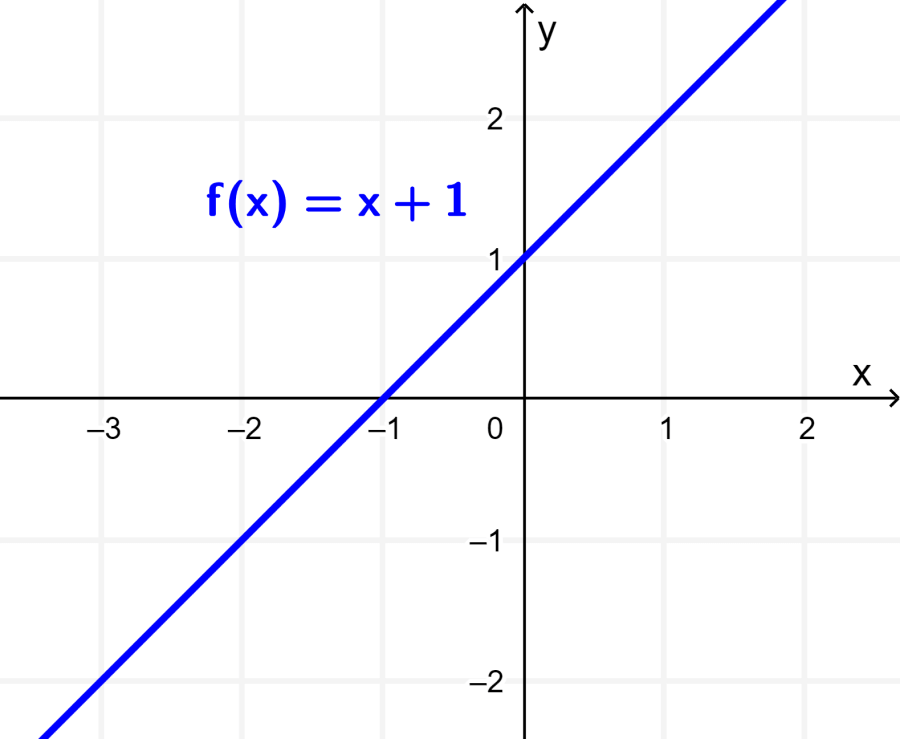
Now, we can perform two different transformations on the function $latex f(x)$ to obtain the following functions:
(i) $latex -f(x)=-(x+1)=-x-1$
(ii) $latex f(-x)=(-x)+1=-x+1$
If we plot functions (i) and (ii) together with the original function $latex f(x)$, we have:
In case (i), the graph of the original function $latex f(x)$ has been reflected over the x-axis.
In case (ii), the graph of the original function $latex f(x)$ has been reflected over the y-axis.
In short, we have:
- The transformation $latex -f(x)$, results in a reflection of the graph of $latex f(x)$ over the x-axis.
- The transformation $latex f(-x)$ results in a reflection of the graph of $latex f(x)$ over the y-axis.
Examples of reflection of functions over the axes
In the following examples, we apply what we have learned about reflecting functions over the x-axis and over the y-axis. Each example has a detailed solution.
EXAMPLE 1
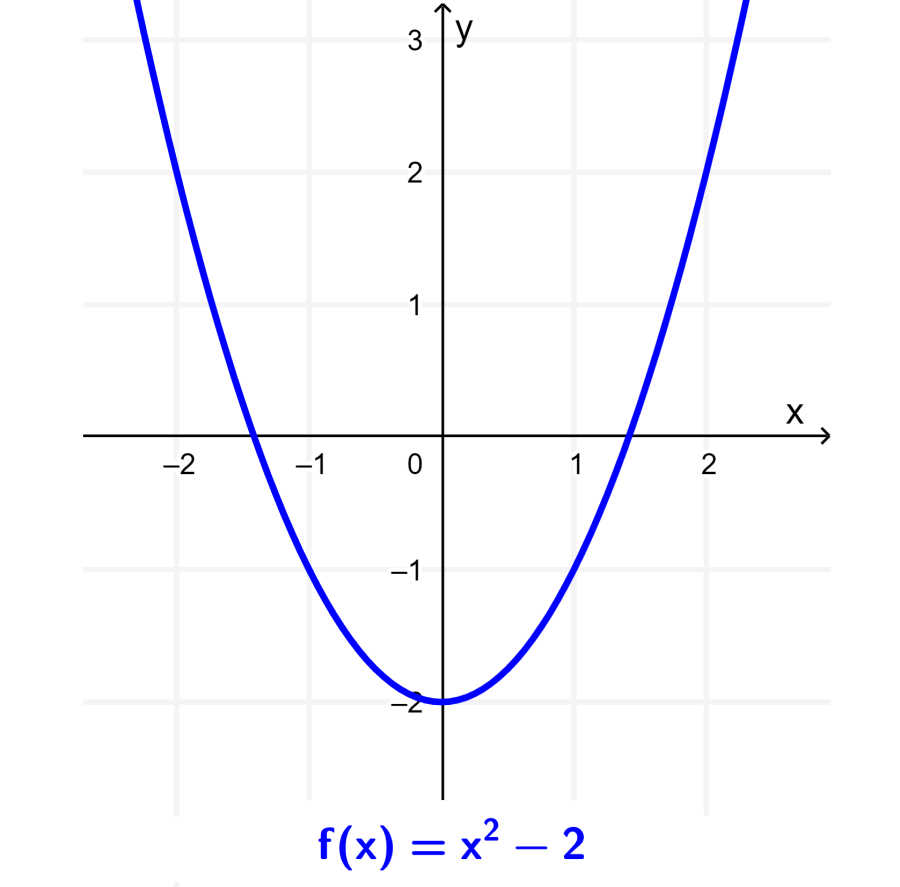
Graph the function $latex f(x)=x^2-2$, and then graph the function $latex g(x)=-f(x)$.
Solution
The graph of f is a parabola shifted 2 units down, as shown in the graph below:
Now, when we apply the transformation on the function g, we get $latex g(x)=-x^2+2$. We can get its graph by reflecting the graph of f over the x-axis:
.
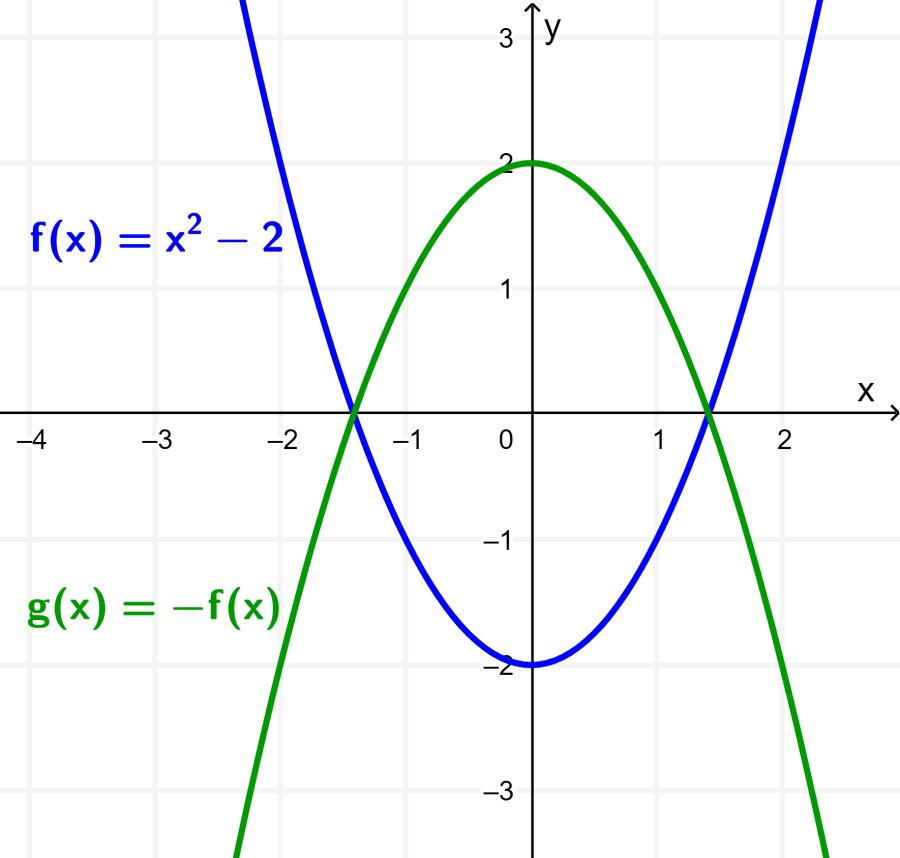
EXAMPLE 2
What is the difference between the graph of $latex f(x)=\cos(2x)$ and the graph of $latex g(x)=\cos(-2x)$?
Solution
The graph of the function $latex f(x)=\cos(2x)$ is as follows:
We can see that the function g is equivalent to $latex g(x)=f(-x)$. Then, the function g is obtained by applying a reflection over the y-axis.
Now, we can see that the graph of $latex f(x)=\cos(2x)$ has symmetry about the y-axis. This means that if we reflect it over the y-axis, we will get the same graph.
Therefore, the graphs of $latex f(x)=\cos(2x)$ and $latex g(x)=\cos(-2x)$ are the same.
EXAMPLE 3
Graph the absolute value function in base form, and then graph $latex g(x)=-|x|$.
Solution
The graph of the absolute value function in its base form, $latex f(x)=|x|$, is as follows:
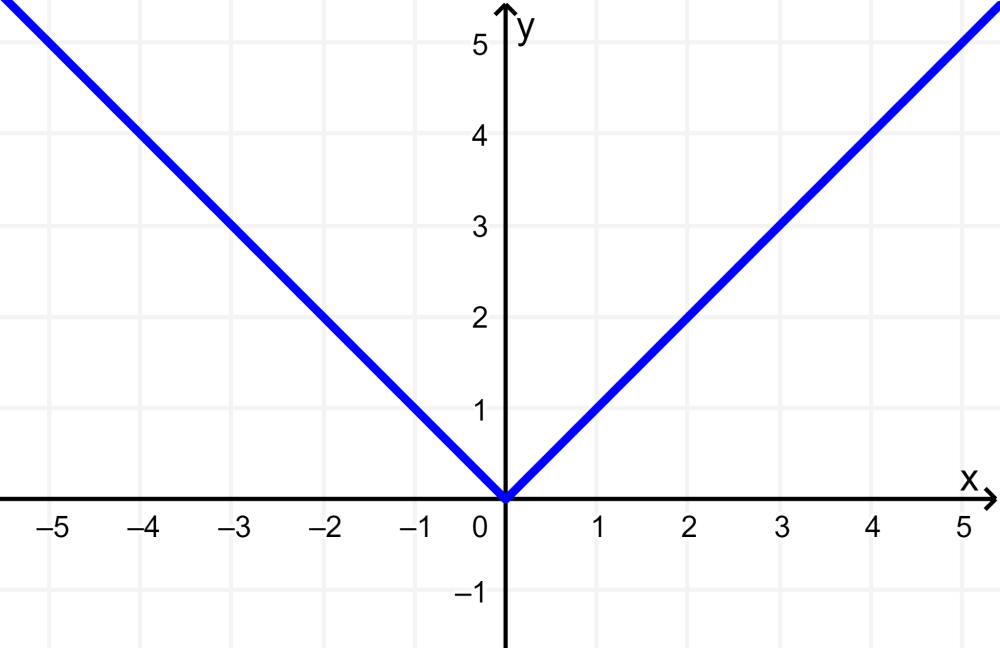
Now, we can see that the function g is equal to $latex g(x)=-f(x)$. Therefore, we get the graph of g by applying a reflection over the x-axis to the graph of f.
.
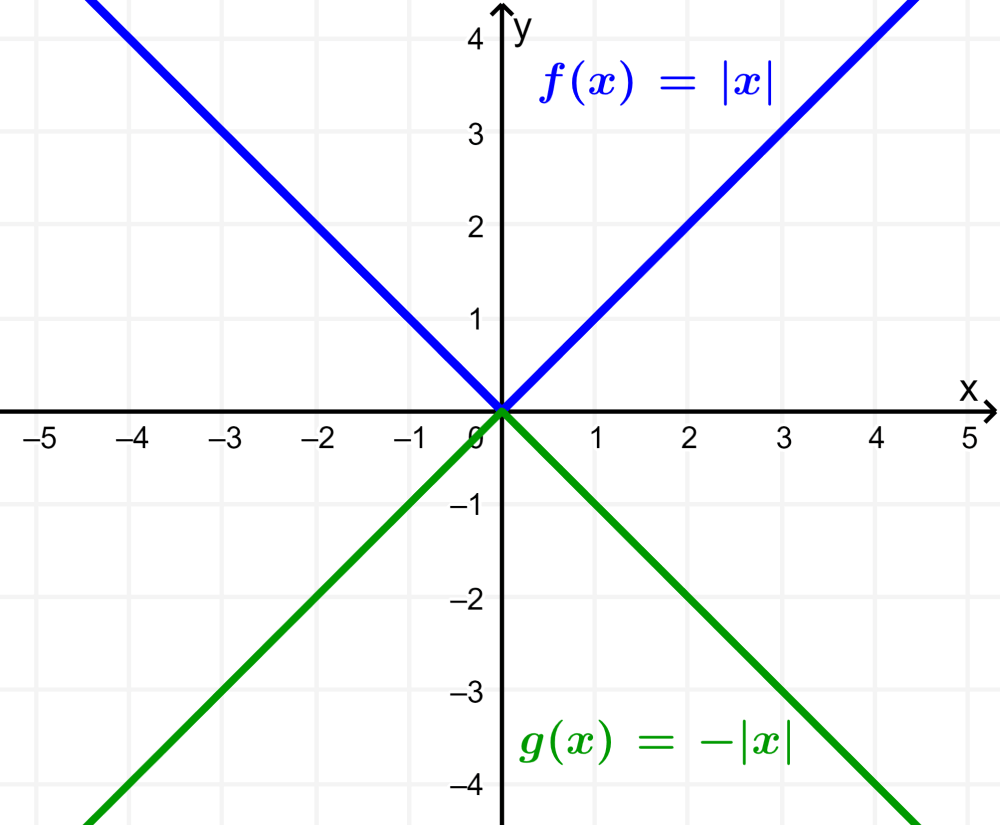
EXAMPLE 4
What is a function that has a reflection over the y-axis of the function $latex f(x)=3x^2+5x+3$?
Solution
To get a reflection over the y-axis, we have to apply the transformation $latex g(x)=f(-x)$.
Therefore, we can find the function g by substituting –x for x in the function f:
$latex g(x)=3(-x)^2+5(-x)+3$
$latex g(x)=3x^2-5-x+3$
Reflection of functions – Practice problems
Solve the following practice problems by using everything you have learned about reflection of functions.
See also
Interested in learning more about function transformations? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
