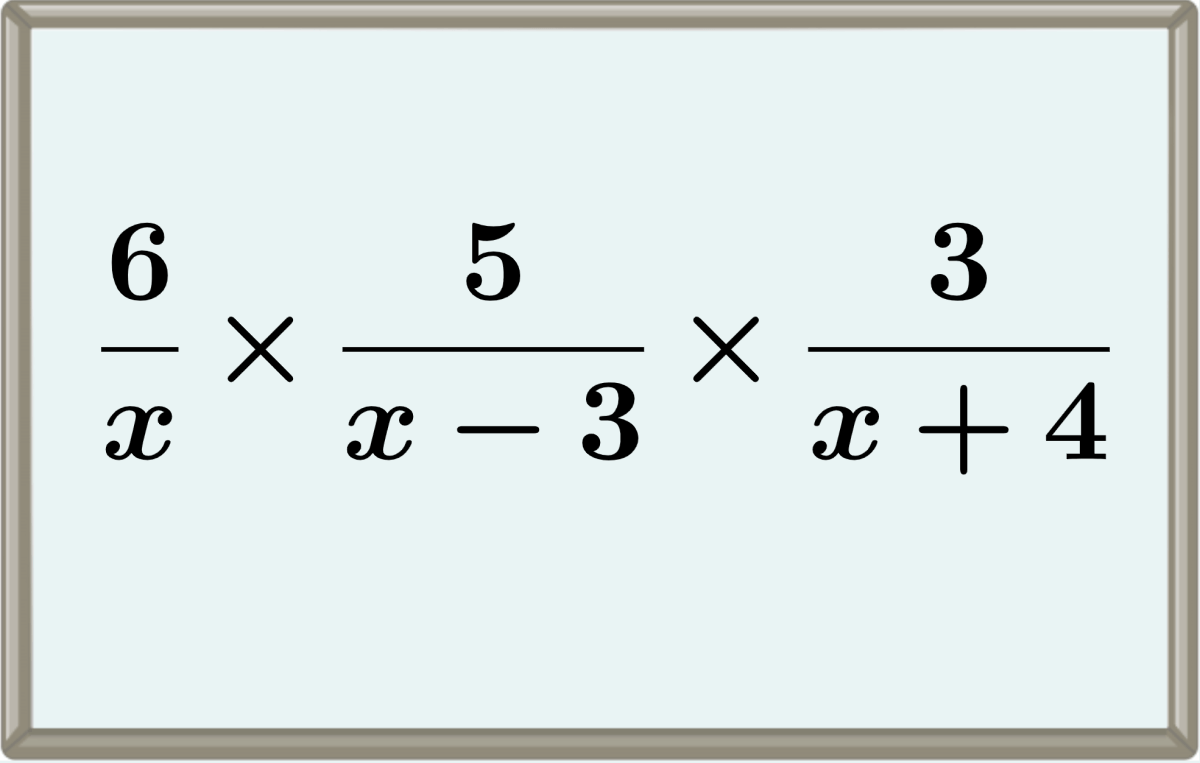Multiplying Algebraic Fractions – Examples and Practice

The fraction resulting from the product (or multiplication) of two algebraic fractions is the product of the numerators divided by the multiplication of their denominators:

$$ \frac{2a}{3b^3} \cdot \frac{3b^2}{4a} = \frac{(2a)\cdot (3b^2)}{(3b^3) \cdot (4a)}$$

Simplifying the common factors in the numerator and denominator, we are left with:

$$ =\frac{(1) \cdot (1)}{(b) \cdot (2)} $$

The end result is:

$$ \frac{1}{2b} $$

Factor the numerator and denominator of each of the fractions shown:

$$ \frac{7(x+1)}{10(x+5)} \cdot \frac{5(x+5)}{2 \cdot 7} $$

Now, multiply numerator by numerator and denominator by denominator:

$$ \frac{7(x+1) \cdot 5(x+5)}{10(x+5) \cdot 2 \cdot 7} $$

We simplify or cancel common factors between the numerator and denominator:

$$ \frac{(x+1)}{2 \cdot 2 } $$

The end result is:

$$ \frac{(x+1)}{4} $$

Multiplying the numerators by each other and the denominators by each other:

$$ \frac{10 \cdot 2a \cdot 3b}{a \cdot b^2 \cdot 20} $$

Simplifying:

$$ \frac{1 \cdot 2 \cdot 3}{1 \cdot b \cdot 2} = \frac{1 \cdot 1 \cdot 3}{1 \cdot b \cdot 1} $$

The end result is:

$$ \frac{3}{b} $$

Numerators and denominators are multiplied together:

$$ \frac{(x^3 + 2x^2 + x)(a – b)}{(a^2 – b^2)(x^2 + x)} $$

The numerator and denominator are factored as much as possible:

$$ =\frac{x(x^2 + 2x + 1)(a – b)}{(a^2 – b^2)(x + 1)x} $$

We can factor the expression $latex x^2+2x+1$ recognizing that this is equal to $latex (x+1)^2$:

$$ =\frac{x(x+1)^2(a – b)}{(a^2 – b^2)(x + 1)x} $$

Using the difference of squares, we have:

$$ =\frac{x(x+1)^2(a – b)}{(a + b)(a – b)(x + 1)x} $$

Now we simplify or cancel like factors between numerator and denominator:

$$ =\frac{1(x+1)(1)}{(a + b)(1)(1)1} $$

Finally we are left with:

$$ \frac{(x+1)}{(a + b)} $$

Multiply the numerators by each other and the denominators by each other:

$$ \frac{(a^2-4a – 5)(2a-2)}{(2a^2-50)(3a+3)} $$

Substitute the first factor of the numerator, which is a quadratic polynomial by the product of two binomials:

$$ (a^2-4a – 5) = (a-5)(a+1) $$

and we take out the common factor wherever possible:

$$ \frac{(a-5)(a+1)2(a-1)}{(2(a^2-25))3(a+1)} $$

Apply the formula for the difference of squares in $latex (a^2-25)$:

$$ \frac{(a-5)(a+1)2(a-1)}{(2(a – 5)(a+5))3(a+1)} $$

We simplify

$$ \frac{(a-1)}{(a+5)3} $$

The end result is:

$$ \frac{(a-1)}{3(a+5)} $$

The given expression is equivalent to:

$$ ( \frac{1}{1} + \frac{a}{b})( \frac{a}{1} – \frac{a^2}{a+b}) $$

First, add and subtract the fractions in each of the expressions between the parentheses:

$$ (\frac{b+a}{b})( \frac{a^2 + ab -a^2}{a+b}) $$

Simplifying, we are left with:

$$ (\frac{b+a}{b})( \frac{ ab }{a+b}) $$

Now we multiply the fractions in the usual way, numerator by numerator and denominator by denominator:

$$ \frac{(b+a)ab}{b(a+b)} $$

After simplifying, i.e. canceling equal factors in the numerator and denominator, we are left with the final answer:

$$ a $$

Considering that $latex x = \frac{x}{1} $ we subtract the fractions in the first parenthesis and add them in the second one:

$$ (\frac{x(y+1) -x}{y+1})( \frac{xy + x}{y}) $$

Expanding the first numerator and take out the common factor x in the second numerator:

$$ (\frac{xy+x -x}{y+1})( \frac{x(y + 1)}{y}) $$

Simplifying:

$$ (\frac{xy}{y+1})( \frac{x(y + 1)}{y}) $$

We multiply the fractions in parentheses:

$$ \frac{x^2y(y+1)}{(y+1)y} $$

The common factors between the numerator and denominator are cancelled, leaving as final result:

$$ x^2 $$

We multiply the numerators and the denominators separately:

$$ \frac{30xb}{10xb^2} $$

After simplifying, we get the following:

$$ \frac{3}{b} $$

We have the product of an addition by a difference, which is equal to a difference of squares:

$$ 1^2-(\frac{x}{y})^2 $$

In the above expression, we have an algebraic fraction squared, which is equivalent to multiplying the fraction by itself:

$$ \frac{1}{1}-\frac{x^2}{y^2} $$

We solve the subtraction of algebraic fractions, and we are left with:

$$ \frac{y^2-x^2}{y^2} $$

Factor the numerator using the product of the difference of squares, to get the final result:

$$ \frac{(y-x)(x+y)}{y^2} $$

Before performing the multiplication, the common factor is taken out in the numerators and denominators:

$$ \frac{15x^2(x + 1)}{10x(2x-3)} \cdot \frac{2(2x-3)}{x+1} $$

Multiplying the fractions:

$$ \frac{30x^2(x + 1)(2x-3)}{10x(2x-3)(x+1)} $$

Canceling the common factors between the numerator and denominator, we have the final result:

$$ \frac{3x}{1} = 3x $$