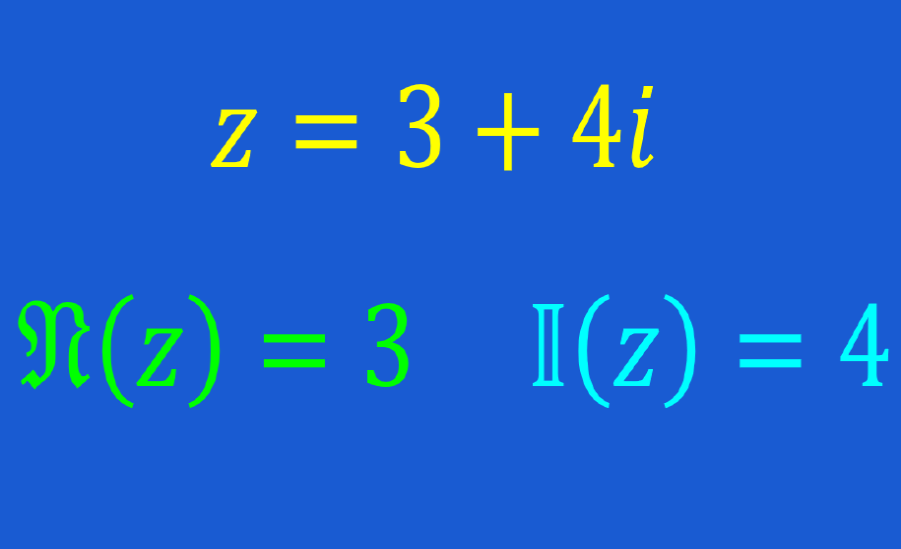Complex numbers have several very useful mathematical properties that greatly facilitate the modelling of sinusoidal systems such as electrical circuits. Basically, we work with complex numbers to avoid dealing with some calculations and to simplify mathematical problems.
What are complex numbers?
Complex numbers are numbers that take the form of a real number plus an imaginary number. Recall that real numbers are numbers like $latex 3,~ -4.5, ~\frac{3}{5}, ~\sqrt{3}$. Imaginary numbers are those numbers that when squared are equal to a negative number. The imaginary unit is i, which is equal to the square root of -1: $latex i= \sqrt{-1}$.
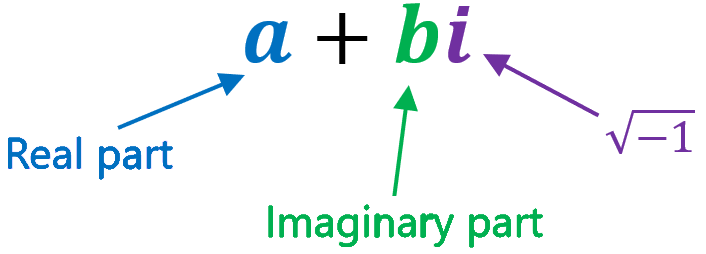
EXAMPLES
The following are examples of complex numbers:
- $latex 3+i$
- $latex 2+3i$
- $latex 10-5i$
- $latex -4-4i$
Applications of imaginary numbers
For most of our tasks in everyday life, real numbers provide an adequate description of the data. Fractions such as $latex \frac{1}{3}$ or $latex \frac{5}{3}$ do not make sense if we are counting people, but they are essential if we are quantifying parts of a group of people.
Negative numbers like -2 or -13 do not make sense when we are measuring the length of an object, but they are essential when we are talking about debts or credits.
In the same way, imaginary numbers are not useful for counting objects in everyday life, but they are essential in concrete applications in various areas of engineering and science such as signal processing, control theory, electromagnetism, vibration analysis, quantum mechanics, and others.
Applications of complex numbers in engineering
The following are some of the applications of complex numbers in engineering:
Control Theory: In control theory, systems are transformed from the time domain to the frequency domain using Laplace transformations. The poles and zeros of the system are analyzed in the complex plane.
Signal Analysis: Complex numbers are used in signal analysis and other fields to obtain a convenient description of signals that vary periodically. For given real functions that represent physical quantities, often in terms of sine and cosine, we can consider corresponding complex functions of which the real parts are the original quantities. For a sine wave of a given frequency, the absolute value |z| of the corresponding number z is the amplitude and the argument, arg(z), is the phase.
Improper integrals: For applied fields such as engineering, complex numbers are often used to calculate improper integrals with real-life values when using complex number functions.
Mechanical and Civil Engineering: The concept of complex geometry and the complex plane is very useful in the construction of buildings and cars. This concept is used in the 2D design of buildings and cars. Complex numbers can also be used in mechanics to represent rotations.
Residuals Theorem: The residuals theorem in complex analysis is a powerful tool for evaluating path integrals of meromorphic functions with respect to closed curves and can often also be used to calculate real integrals.
Applications of complex numbers in computer science
Complex numbers are not particularly fundamental in computing, but they appear in various places where their knowledge is necessary to build applications. The first place we see complex numbers in computer science is in the prototypical example of a class or type.
A complex number is an easy-to-understand example of an object in the object-oriented programming sense. We can show the data, a real part and an imaginary part, and the operations on the data implemented as methods. This is a rare case of the use of operator overload.
The second place where complex numbers appear is in computer graphics. In the 2D case, the points can be complex numbers and the rotations, translations, and reflections of those points correspond to various operations on the complex numbers. Complex numbers generalize to quaternions, which are useful for 3D graphs.
There are other places where complex numbers can appear, generally as application knowledge that needs to be understood before a program can be written. For example, we would need complex numbers for algebraic computational systems or quantum mechanical simulations.
Applications of complex numbers in electronics
Complex numbers have fundamental applications in electronics. The voltage produced by a battery is characterized by a real number called potential, for example, +10 volts or -10 volts. However, the “AC” voltage in a home requires two parameters.
One is a potential, like 120 volts and the other is an angle called the phase. Voltage has two dimensions, and a two-dimensional quantity can be represented mathematically as a vector or a complex number (known in engineering as a phasor).
In the representation of vectors, the rectangular coordinates are referred to simply as X and Y. In the representation of complex numbers, the same components are referred to as real and imaginary.
When the complex number is purely imaginary, such as a real part of 0 and an imaginary part of 120, that means that the voltage has a potential of 120 volts and a phase of 90°, which is a completely real measurement.
In electrical engineering, the Fourier transform is used to analyze varying voltages and currents. The treatment of resistors, capacitors, and inductors can be unified by introducing imaginary resistors that are frequency-dependent and combining them into a single complex number called the impedance.
This use is also extended to digital signal processing and digital image processing, which use digital versions of Fourier analysis to transmit, compress and restore image, auto, or video signals. Electrical engineers use the letter j for the imaginary unit as it is typically reserved for varying currents.
Applications of complex numbers in physics
The following are the areas of physics in which complex numbers are used:
Fluid Mechanics: Fluid mechanics and its sub-disciplines such as aerodynamics, hydrodynamics, and hydraulics have a wide variety of applications. For example, we can calculate forces and moments of inertia in an airplane, the mass flow of oil through pipelines, and predictions of weather patterns. In fluid mechanics, complex numbers are used to describe potential flow in two dimensions.
Electromagnetism: Instead of taking the magnetic and electrical parts as two different real numbers, we can represent it as a complex number.
Relativity: In general and special relativity, some formulas for measuring space-time become simpler if we take the time variable as imaginary. Although this is no longer standard in classical relativity, it is used in an essential way in quantum field theory.
Quantum mechanics: Complex numbers are relevant in the formulation of quantum mechanics, where complex Hilbert spaces provide the space for such formulations that are convenient and perhaps standard. The foundational formulas of quantum mechanics – Schrödinger’s equation and Heisenberg’s matrix mechanics – use complex numbers.
See also
Interested in learning more about applications of functions? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
