There are several applications of rational functions in everyday life. We can form rational equations and formulas to calculate speeds or distances, calculate the work rate of people or machines, and we can solve mixing problems. Rational functions even have applications in medicine and economics to model real scenarios.
Applications of rational functions in everyday life
Medicine
Rational functions have applications in medicine. Before an operation, a patient can be injected with some medication. When the concentration of the drug in the blood is at the desired level, the operation can continue.
The concentration of the drug in the blood can be modeled using a rational function. For example, the hypothetical function $latex C(t) = \frac{3t}{{{t}^2} +3}$ could help a doctor determine the concentration of the drug in the blood after a few minutes or hours.
Economics
Rational functions can be used to model average cost functions. Average cost functions help a business determine the cost of producing a certain product. For example, suppose our company produces flashlights and we want to determine the average cost to produce flashlights.
We can model the average cost to produce flashlights using the function $latex C(x)=\frac{(fixed~cost)+cx}{x}$, where the fixed cost is the cost necessary to maintain the business, c is the cost of each lantern and x is the number of lanterns produced.
Sharing things and assets
If there is a very standard application of rational functions in real life, it is to share things with others. The simplest real-life example is when you share a cookie with a friend and that cookie will be divided in half, which is already an application of rational functions by simple division.
The same can be said in more complicated matters, such as when you share your real assets and property with a loved one, or when you have shareholders in a corporation where each shareholder owns a financial asset of the company.
Proportionality
The numerator and denominator of rational functions are inversely proportional to each other when equated to a certain variable. That means that when a variable is equated to a rational expression, the numerator is said to be directly proportional, while the denominator is inversely proportional.
A quantity can be considered directly proportional when it has an equilibrium or a proper relationship to the size or quantity of the matched variable, such as when a quantity increases, the matched variable also increases. The opposite happens in an inversely proportional amount.
Time
Time is measured rationally. As the present progresses, the future gets closer, but the past becomes more distant. Apart from this, when we measure different units of time, we also use rational functions, especially when we convert seconds to minutes to hours, etc., as seconds are sixtieth of minutes, which are sixtieth of hours.
Physics
Rational functions are used in physics to model phenomena such as wave motion and propagation. For example, in mechanics, rational functions are used to model the motion of particles and rigid bodies.
In wave propagation, rational functions are used to model the propagation of electromagnetic and acoustic waves.
Robotics
Rational functions are used in robotics to model the kinematics and dynamics of robots. They are used to represent the relationships between the joints and actuators of a robot and the movement of the robot’s end effector.
They are also used to design controllers that stabilize the robot’s motion and achieve the desired performance.
Geometry
Rational functions are used in geometry to study algebraic curves and surfaces. They are used to represent the equations of these curves and surfaces and to analyze their geometric properties.
To solve problems with rational formulas
Rational functions can be used to represent real-life situations and to find solutions to real problems. Equations representing direct, inverse, or joint variations are examples of rational functions that can model everyday situations.
To solve problems involving rational formulas, it is recommended to start by solving the formula for the specified variable. For example, we may have a problem in which we have to calculate the time it takes to cover a certain distance by traveling at a given speed.
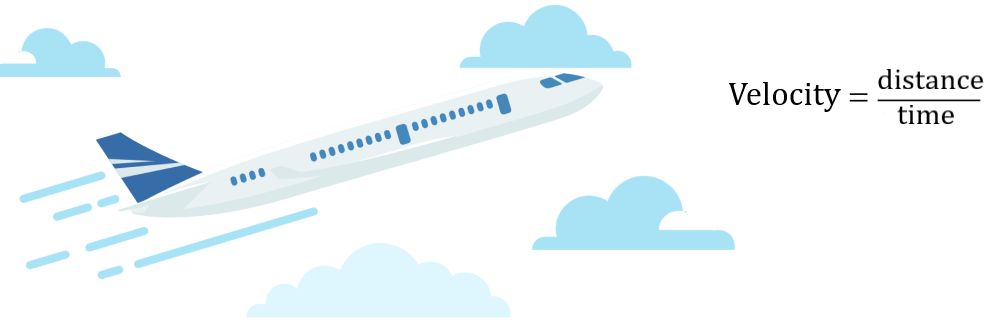
Algebraic models for such situations involve rational equations derived from the distance formula, $latex d=vt$. The distance traveled (d) is the product of the speed (v) and the elapsed time (t). Using algebra, we can write the formula in three different ways:
$latex d=vt$
Find the time: $latex t=\frac{d}{v}$
Find the velocity: $latex v =\frac{d}{t}$
EXAMPLE 1
The formula for finding the volume of a cone is $latex V=\frac{1}{3}\pi {{r}^2}h$, where V is the volume, r is the radius and h is the height of the cone. Rearrange the formula to find the height (h).
Solution: We start with the formula for the volume of a cone:
$latex V=\frac{1}{3}\pi {{r}^2}h$
We multiply both sides by 3:
$latex 3V=\pi {{r}^2}h$
We divide both sides by $latex \pi {{r}^2}$ to isolate the h:
$latex \frac{3V}{{\pi {{r}^2}}}=\frac{{\pi {{r}^2}h}}{{\pi {{r}^2}}}$
We simplify to find the height:
$latex \frac{3V}{{\pi {{r}^2}}}=h$
EXAMPLE 2
The formula for finding the density of an object is $latex D=\frac{m}{v}$, where D is the density, m is the mass of the object and v is the volume of the object. Rearrange the formula to find the volume.
Solution: We start with the formula for density:
$latex D=\frac{m}{v}$
We multiply both sides of the equation by v:
$latex vD=\frac{vm}{v}$
Now, we divide both sides by D and simplify to find the volume:
$latex \frac{vD}{D}=\frac{vm}{vD}$
$latex v=\frac{m}{D}$
To solve work problems
Rational functions and rational equations can be used in a wide variety of problems related to rates, time, and work. It is possible to determine how to combine workers or machines to complete a job using rational expressions and functions.
A work problem is an example of one of the applications of rational functions. Work problems often ask us to estimate how long it will take different people working at different rates to complete a task or job. Algebraic models for these situations often involve rational equations derived from the work formula, $latex T = rt$.
This formula is similar to the formula for distance $latex d= vt$. The amount of work (T) is equal to the rate of work (r) multiplied by the time worked (t). The work formula has three versions:
$latex T=rt$
$latex t=\frac{T}{r}$
$latex r=\frac{T}{t}$
Some problems involve multiple people or machines working at different rates. In these cases, we can add all the work rates to obtain a total work rate.
EXAMPLE
Carl takes 2 hours to water 60 plants. Manuela takes 3 hours to water 60 plants. If they work together, how long would it take them to water 200 plants?
Solution: To facilitate the resolution of the problem, we can think about how many plants each person can water in 1 hour:
Carl: $$\frac{60~\text{plants}}{2~\text{hours}}=\frac{30~\text{plants}}{1~\text{hour}}$$
Manuela: $$\frac{60~\text{plants}}{3~\text{hours}}=\frac{20~\text{plants}}{1~\text{hour}}$$
We combine their work rhythms to determine the work rhythm when they work together.
Manuela and Carl: $$\frac{30~\text{plants}}{1~\text{hour}}+\frac{20~\text{plants}}{1~\text{hour}}=\frac{50~\text{plants}}{1~\text{hour}}$$
We use one of the formulas from work to write a rational function, for example, $latex r=\frac{W}{t}$. We know the value of r, the combined pace of work, and we know W, the amount of work to be done and we have to calculate for the time. Therefore, we have
$latex \frac{50}{1}=\frac{200}{t}$
We solve the equation by multiplying both sides by t:
$latex \frac{50}{1}t=\frac{200}{t}t$
$latex 50t=200$
$latex t=\frac{200}{50}$
$latex t=4$ hours
Therefore, if Carl and Manuela work together, it would take them 4 hours to water 200 plants.
Solve mixing problems
Mixtures are made up of proportions of different substances such as gases, water, food, or chemicals. Mixtures are found in many products or even naturally around us. For example, chemical reactions and manufacturing involve mixtures.
Mathematically, mixtures can be more interesting when the components of the mixture are added at different rates and concentrations. In the following example, we will look at the mixture of water and salt.
EXAMPLE
In a container, we have 20 liters of water and we mix 1 pound of salt. We add water at a rate of 2 liters per minute and at the same time add salt at a rate of 0.2 pounds per minute. Find the concentration in the container after 10 minutes.
Solution: We can use t to represent the number of minutes since we started adding water and salt. Since water increases at 2 liters per minute and salt at 0.2 pounds per minute, these are constant rates. This tells us that the amount of water and the amount of salt are linear. We can write an equation for each one:
Water: $latex A(t)=20+2t$ in liters
Salt: $latex S(t)=1+0.2t$ in pounds
The concentration, C, will be the ratio of pounds of salt to liters of water:
$latex C(t)=\frac{1+0.2t}{20+2t}$
The concentration after 10 minutes is given by evaluating $latex C(t)$ in $latex t=10$:
$latex C(10)=\frac{1+2}{20+20}$
$latex =\frac{3}{40}$
This means that the concentration is 3 pounds of salt to 40 liters of water.
See also
Interested in learning more about applications of functions? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
