There are a wide variety of applications of linear functions. Linear functions are used to model real-life problems. These problems are converted into linear mathematical equations that are then solved using various algebraic methods.
With linear functions, we can solve strategy problems, geometry problems, percentages and money problems, and uniform motion problems.
Applications of linear functions in everyday life
The applications of linear functions in everyday life are vast. Some common applications involve solving:
- Age problems
- Speed, time and distance problems
- Geometry problems
- Percentage and money problems
- Pressure and force problems
- Salary problems
These everyday problems are converted to mathematical forms to form linear equations, which are solved using various methods. These equations should clearly explain the relationship between the data and the variables.
Mathematical translation and strategy
Linear functions help us simplify the process of solving real-life problems. It is possible to achieve this thanks to the use of letters to represent variables and unknown values, transformation of word problems to equations, and systematic techniques to solve these equations.
To solve problems using linear equations, we begin by translating word problems into mathematical formulations that describe the relationship between the given information and the unknowns. The key to this translation is to read the problem carefully and identify certain keywords and phrases. The following are examples of translated key phrases:
| Key phrases | Translation |
| The sum of a number and 5. | $latex x+5$ |
| Six more than a number. | $latex x+6$ |
| Four less than a number. | $latex x-4$ |
| Seven subtracted from a number. | $latex x-7$ |
| The product of four and a number. | $latex 4x$ |
| Three times a number. | $latex 3x$ |
| A third of a number. | $latex x/3$ |
| The quotient of a number and 4. | $latex x/4$ |
Problems with relationships between real numbers
With linear equations, we can solve a wide variety of problems with real numbers. Once we have the linear equation equivalent to the word problem, we can use algebraic techniques to find the answer to the problem.
EXAMPLE
A large integer is 4 less than 3 times a small integer. The sum of both integers is 12. Find both numbers.
Solution: We start by identifying the variables. Therefore, we assign a variable to the small number.
We are going to use $latex x$ to represent the small number
We use the first sentence to identify the large number in terms of the variable x: “A large integer is 4 less than 3 times a number”.
$latex 3x-4$ represents the large number
We form the equation that represents both numbers which is indicated by the second sentence: “The sum of both integers is 12″.
$latex x+(3x-4)=12$
Now, we solve the equation to get the small number x:
$latex x+(3x-4)=12$
$latex x+3x-4=12$
$latex x+3x=12+4$
$latex 4x=16$
$latex x=4$
We use the expression $latex 3x-4$ to find the large number:
$latex 3(4)-4=12-4=8$
The integers are 8 and 4.
Geometry problems
Several geometry problems require the use of linear equations to be solved. For example, let’s remember that the perimeter of a polygon is the sum of the lengths of its sides. Additionally, let’s remember some of the perimeter formulas:
| Geometric figure | Perimeter |
| Square | $latex P=4l$ |
| Rectangle | $latex P=2l+2a$ |
| Triangle | $latex P=a+b+c$ |
| Circle (circumference) | $latex P=2\pi r$ |
EXAMPLE
A rectangle has a perimeter that is 36 meters. The length is 2 meters more than 3 times the width. Find the dimensions of the rectangle.
Solution: The phrase “The length is 2 meters more than 3 times the width” gives us the relationship between the two variables:
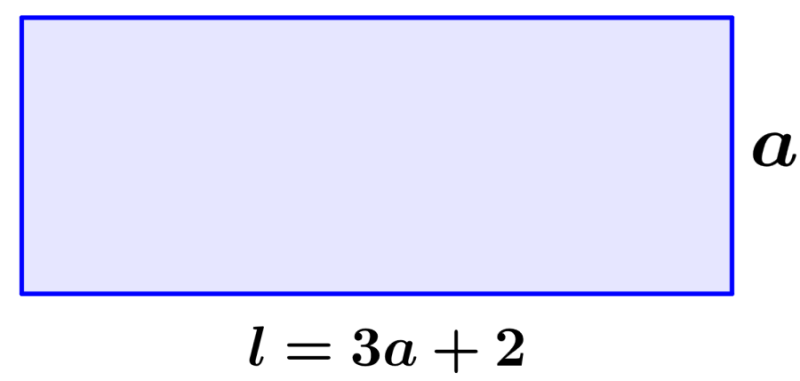
We use a to represent the width of the rectangle.
We use $latex 3a+2$ to represent the length.
The phrase “A rectangle has a perimeter that measures 36 meters” gives us an algebraic equation. Therefore, we use the formula for the perimeter of the rectangle and substitute with the given information:
$latex P=2l+2a$
$latex 36=2(3a+2)+2a$
Now that we have an equation with one variable, we solve for the width, a:
$latex 36=6a+4+2a$
$latex 36=8a+4$
$latex 32=8a$
$latex 4=a$
We use $latex 3x+2$ to find the length:
$latex 3(4)+2=12+2=14$
The rectangle measures 14 meters by 4 meters. To verify, we add all the sides:
$latex P=14+14+4+4=36$
Problems with percentages and money
To solve problems related to percentages, we have to transform percentages into decimals or fractions. If the problem asks us to find a percentage, then we must remember to transform it back to a percentage at the end.
Also, if the question involves money, we need to make sure we round to two decimal places.
EXAMPLE
If a jacket costs 50.00 dollars including an 8.5% tax, what is the original price before taxes are added?
Solution: We start by converting 8.5% to a decimal. Then, we have 0.085.
The amount of tax is equal to this rate multiplied by the original cost of the jacket. The original cost of the jacket is what we want to find.
We use c to represent cost before adding taxes.
$$value~of~tax=tax\times cost~of~object$$
$latex =0.085\times c$
$$total~cost=cost~of~object+value~of~tax$$
$latex 50.00=c+0.085c$
We use this equation to solve for c, the original cost of the object.
$latex 50.00=1c+0.085c$
$latex 50.00=1.085c$
$latex 46.08=c$
The cost of the jacket before taxes is 46.08 dollars.
Uniform motion problems
Uniform motion refers to motion with a given speed where the rate does not change, that is, there is no acceleration. We can determine the distance traveled using a linear function, we multiply the speed by the time traveled with the formula $latex d=vt$.
EXAMPLE
A car is traveling at 50 km/h. a) If it travels for 3.5 hours, what is the distance traveled? b) If you need to travel 350 kilometers, how long will it take?
Solution: a) Using the formula for distance $latex d=vt$, we simply replace the given information in this formula:
$latex d=(50)(3.5)$
$latex d=175$
Therefore, traveling at a speed of 50 km/h for 3.5 hours, the car covers a distance of 175 km.
b) We plug the data into the formula for distance and solve for time:
$latex 350=50t$
$latex 7=t$
Therefore, traveling at a speed of 50 km/h, we need 7 hours to cover a distance of 350 km.
See also
Interested in learning more about applications of functions? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
