The tangent of an angle can be defined using a right triangle. The tangent is equal to the length of the side opposite the angle divided by the length of the adjacent side. Although the tangent is defined with the angles of a right triangle, the tangent function can be used for any angle.
Here, we will learn more about the tangent of an angle. We will learn about the values of the tangent of important angles and we will solve some practice problems.
Definition of the tangent of an angle
The sine and cosine are not the only frequently used trigonometric functions. The tangent of an angle is another very important trigonometric function. The tangent is defined in terms of the unit circle.
In the diagram below, the tangent is the length of the vertical line ED that is tangent from the point of tangency, E, to the point D where the tangent line intersects the ray AD formed by the angle.
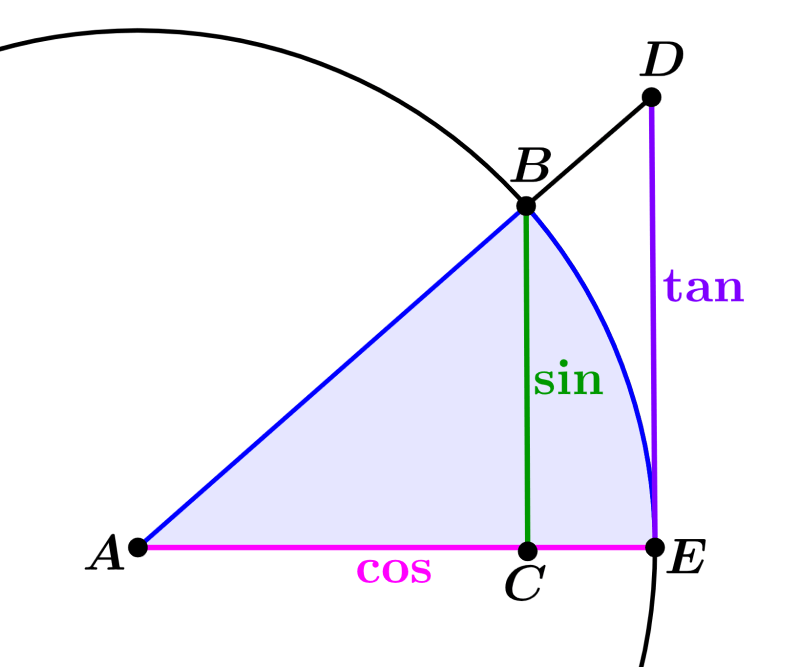
Tangents in terms of sine and cosine
The triangles ABC and ADE in the diagram above are similar, so the proportions of their sides are equal. This means that we have:
$latex \frac{ED}{AE}=\frac{CB}{AC}$
Looking at the diagram, we see that we have $latex EA=\tan(A)$. We use the unit circle, so we have $latex AE=1$. In addition, we also have the relationships $latex CB=\sin(A)$ and $latex AC=\cos(A)$. Using this, we find the fundamental identity:
| $latex \tan(A)=\frac{\sin(A)}{\cos(A)}$ |
Tangent and right triangles
Similar to how the sine and cosine can be found in terms of the sides of a right triangle, we can also do the same with the tangents. We are going to use the right triangle ABC which has a right angle at C.
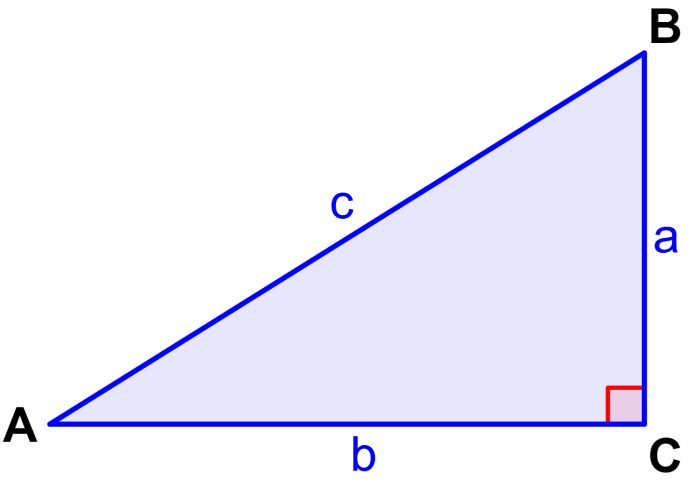
We can use the relationships that we have already seen. First we have $latex \tan(A)=\frac{\sin(A)}{\cos(A)}$. We also use the relationships$latex \sin(A)=\frac{a}{c}$ and $latex \cos(A)=\frac{b}{c}$. Using this, we can divide $latex \frac{a}{c}$ by $latex \frac{b}{c}$.
Canceling c in the numerator and denominator, we conclude that $latex \tan(A) = \frac{a}{b}$. This means that the tangent is equal to the opposite side divided by the adjacent side:
| $latex \tan(x)=\frac{\text{opposite}}{\text{adjacent}}$ |
Tangents for common special angles
The tangent of the most common angles is found using the proportions of the sides of special triangles and the fact that the tangent is equal to the sine over the cosine. For example, we are going to use a right isosceles triangle, which has the angles 45°-45°-90°.
We can use the Pythagorean theorem: $latex {{c}^2}={{a}^2}+{{b}^2}$. In this case, two sides are equal, that is, $latex a=b$. Therefore, we have $latex {{c}^2}=2{{a}^2}$. This means that we have $latex c=a\sqrt{2}$.
Therefore, both the sine and cosine of 45° are equal to $latex \frac{1}{\sqrt{2}}$ or $latex \frac{\sqrt{2}}{2}$. Since the tangent is equal to the sine over the cosine, the 45° tangent is equal to 1.
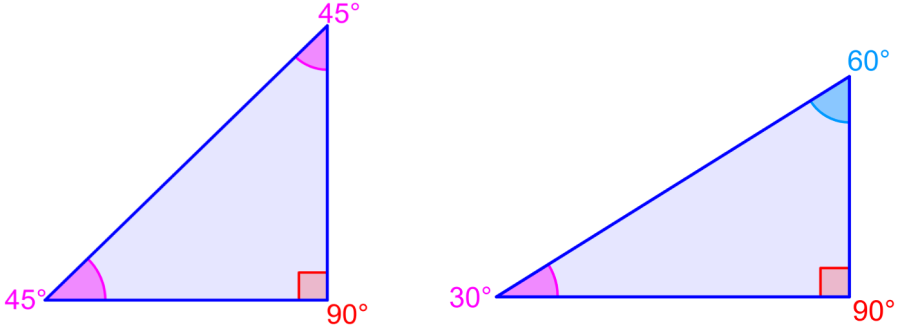
We also use the special triangle 30°-60°-90°. This triangle has sides of ratio 1:$latex \sqrt{3}$:2. Using these proportions, we have $latex \sin(30^{\circ})=\cos(60^{\circ})=\frac{1}{2}$ and we also have $latex \sin(60^{\circ})=\cos(30^{\circ})=\frac{\sqrt{3}}{2}$.
| Degrees | Radians | Sine | Cosine | Tangent |
| 90° | $latex \frac{\pi}{2}$ | 1 | 0 | Undefined |
| 60° | $latex \frac{\pi}{3}$ | $latex \frac{\sqrt{3}}{2}$ | $latex \frac{1}{2}$ | $latex \sqrt{3}$ |
| 45° | $latex \frac{\pi}{4}$ | $latex \frac{\sqrt{2}}{2}$ | $latex \frac{\sqrt{2}}{2}$ | 1 |
| 30° | $latex \frac{\pi}{6}$ | $latex \frac{1}{2}$ | $latex \frac{\sqrt{3}}{2}$ | $latex \frac{\sqrt{3}}{3}$ |
| 0° | 0 | 0 | 1 | 0 |
Tangent of an angle – Examples with answers
The following examples are solved using what has been learned about the tangents of angles. All examples refer to the right triangle seen above.
EXAMPLE 1
In a triangle, we have $latex \tan(A) = 1.2$ and $latex b = 5$, what is the value of a?
Solution
We use the triangle above as a reference. Therefore, we have the relation $latex \tan(A) = \frac{b}{a}$. Using the values given in this formula and solving for c, we have:
$latex \tan(A)=\frac{b}{a}$
$latex 1.2=\frac{5}{a}$
$latex a=\frac{5}{1.2}$
$latex a=4.17$
The length of a is 4.17.
EXAMPLE 2
Determine the value of b if we have $latex a=11$ and $latex \tan(B)=0.78$.
Solution
We use the triangle above as a reference and we can obtain the relation $latex \tan (B) = \frac{b}{a}$. We substitute the values given in this formula and when solving for c, we have:
$latex \tan(B)=\frac{b}{a}$
$latex 0.78=\frac{b}{11}$
$latex b=0.78(11)$
$latex b=8.58$
The length of b is 8.58.
EXAMPLE 3
Determine the measure of angle A if we have $latex a = 12$ and $latex b = 8$.
Solution
We form the expression $latex \tan(A)=\frac{a}{b}$. Therefore, using the given values, we have:
$latex \tan(A)=\frac{a}{b}$
$latex \tan(A)=\frac{12}{8}$
$latex \tan(A)=1.5$
Now, we have to use the $latex {{\tan}^{-1}}$ function in a calculator to get the result:
$latex {{\tan(1.5)}^{-1}}=56.3$°
Angle A measures 56.3°.
→ Tangent Calculator (Degrees and Radians)
Tangent of an angle – Practice problems
Use the tangent formula seen above to solve the following practice problems. Determine the length of a side or the measure of an angle with the given information.
See also
Interested in learning more about tangent of an angle? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
