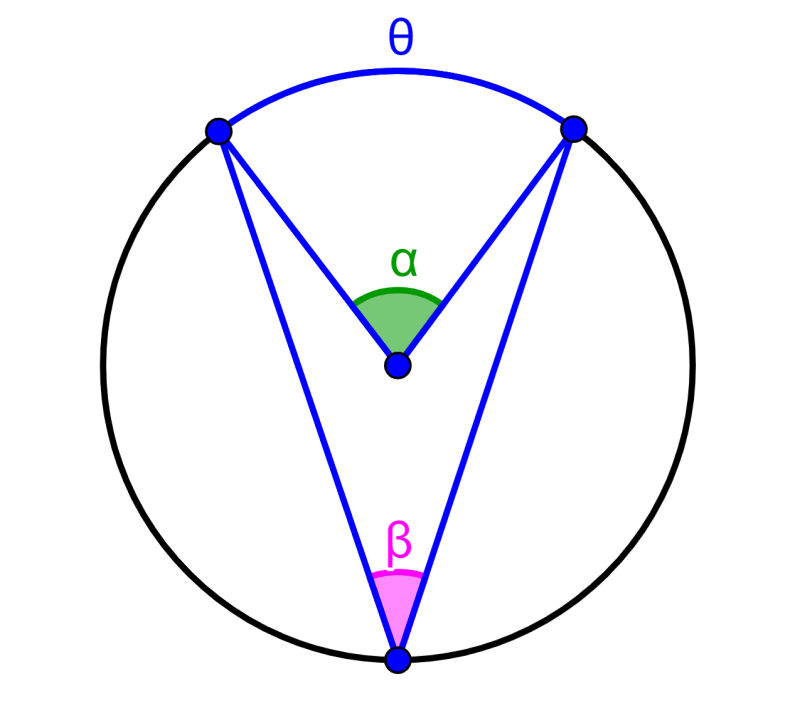
The inscribed angle theorem indicates that when we have a central angle and an inscribed angle that intersect in the same arc of the circle, the central angle is twice the size of the inscribed angle. Depending on how the angles are located, we can have three different cases of this theorem. Each of these cases needs a different method to be proved.
Here, we will learn about the different cases of the inscribed angle theorem and their respective proof.
Explanation of the inscribed angles theorem
The inscribed angle theorem states that when the central angle and the inscribed angle share endpoints on the circle, the value of the central angle is twice that of the inscribed angle.
Recall that an inscribed angle is an angle inside the circle that is formed where two chords meet.
A central angle is an angle in the interior of a circle, in which the vertex is the center of the circle and the sides are the radii.
In the diagram below, the green angle is a central angle and the pink angle is an inscribed angle.
Proof 1: Inscribed angle between a chord and the diameter of the circle
This case can be observed in the following diagram:
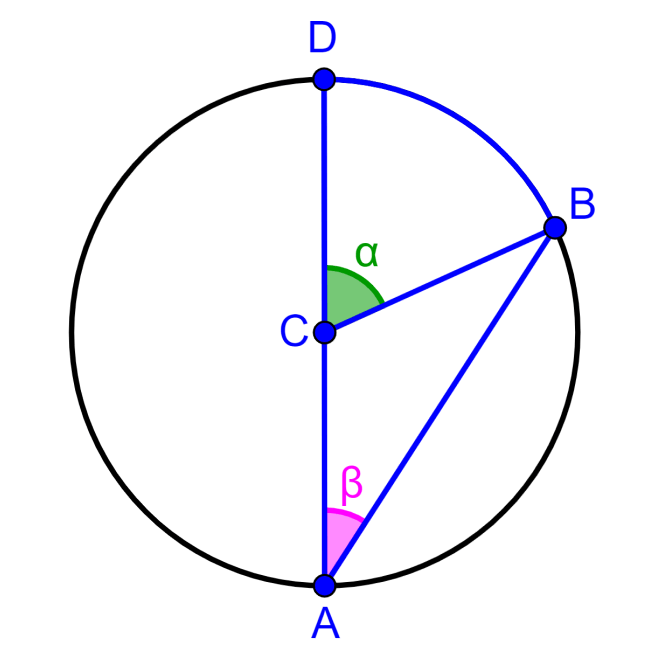
We are going to prove that α=2β.
In this diagram, triangle ABC is an isosceles triangle since segments AC and BC are radii of the circle and we have AC = BC. Therefore, the angles ∠CAB and ∠ABC are equal. That is, we have ∠CAB=∠ABC=β.
The diameter AD is a straight line, so the angle ∠BCA=(180°-α).
We know that the angles in a triangle add up to 180°, so we have:
∠CAB+∠ABC+∠BCA=180°
Now, we simplify the angles by using their known values and we have:
β+β+180°-α=180°
Simplifying and solving, we have:
β+β+180°-α=180°
2β-α=0
2β=α
We have proved the theorem.
Proof 2: Diameter between the sides of the inscribed angle
In the following diagram, we can look at the second case:
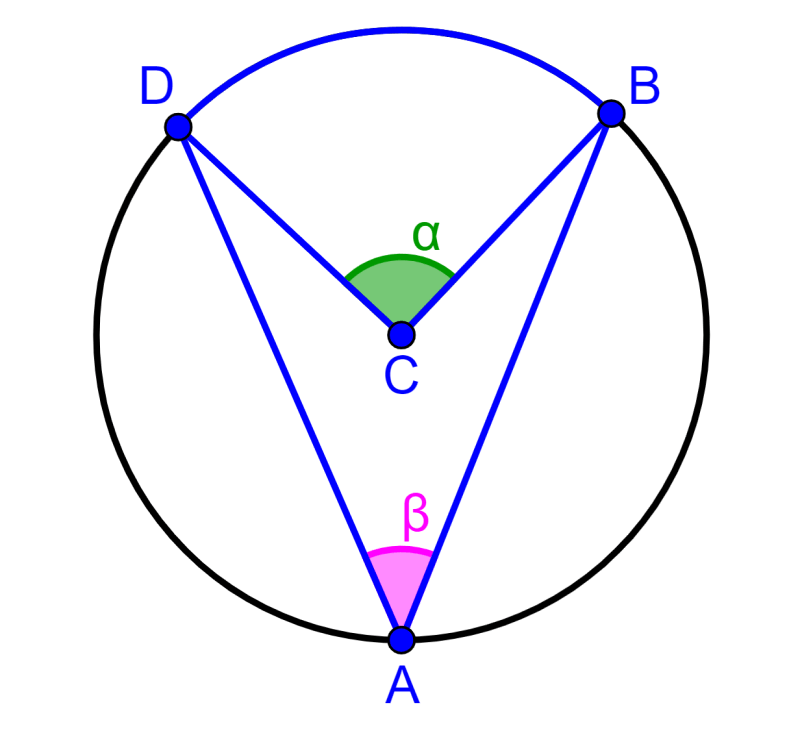
We are going to prove that 2β=α.
We can plot the diameter of the circle and represent the following important angles:
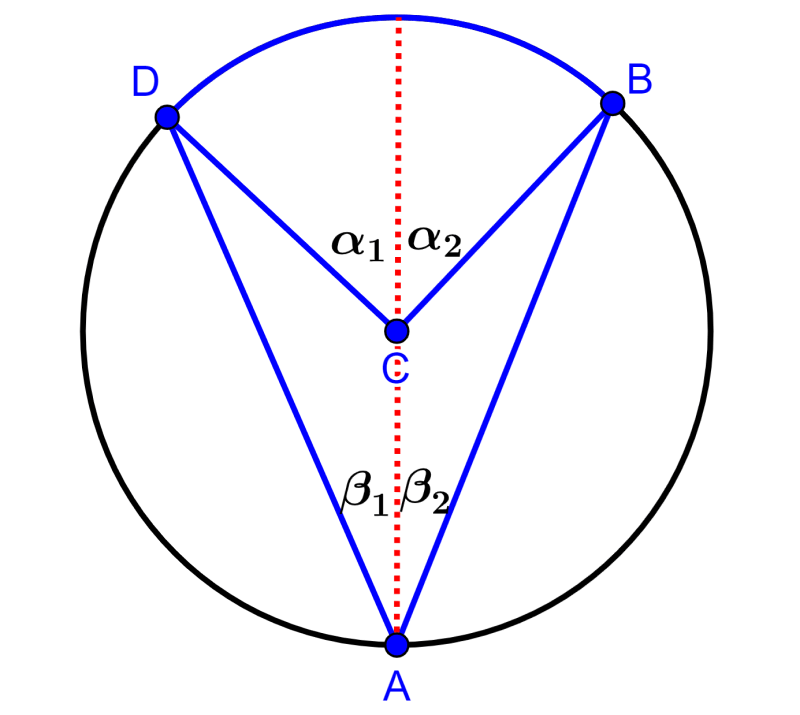
We can see that the diameter divides the angles α and β. Therefore, we have:
$latex \alpha_{1}+\alpha_{2}=\alpha$
$latex \beta_{1}+\beta_{2}=\beta$
Taking into account the first case we saw above, we can deduce the following:
$latex 2\beta_{1}=\alpha_{1}$
$latex 2\beta_{2}=\alpha_{2}$
Now, we can add the angles and simplify using $latex \alpha=\alpha_{1}+\alpha_{2}$ and $latex \beta=\beta_{1}+\beta_{2}$:
$latex 2\beta_{1}+2\beta_{2}=\alpha_{1}+\alpha_{2}$
$latex 2\beta=\alpha$
We have proved the theorem.
Proof 3: Diameter outside the sides of the inscribed angle
This case is represented in the following diagram:
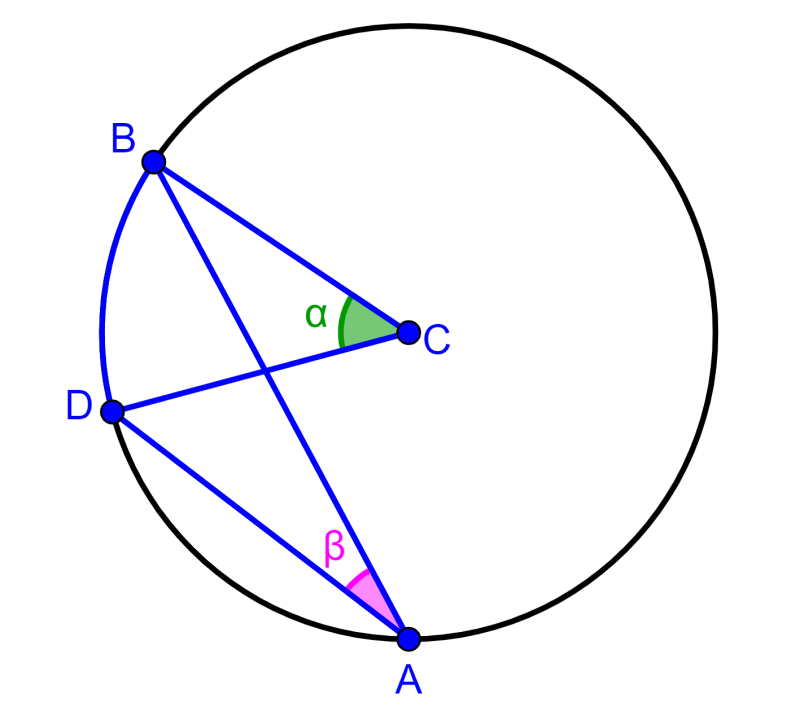
We are going to prove that 2β=α.
Similar to the previous case, we are going to plot the diameter of the circle and represent the important angles.
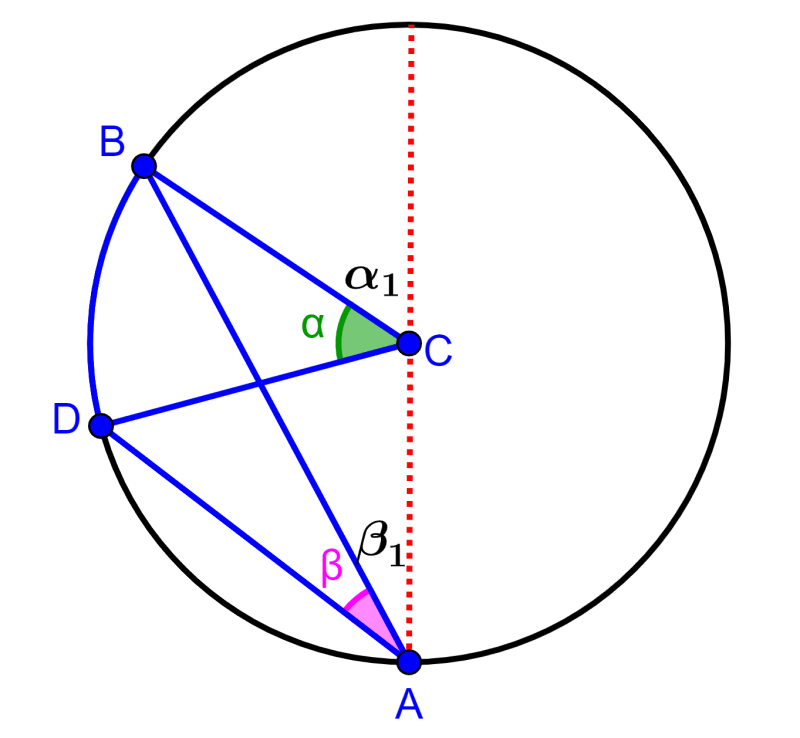
Again, we now that $latex 2\beta_{1}=\alpha_{1}$. Therefore, we have:
$latex 2(\beta_{1}+\beta)=\alpha+\alpha_{1}$
Using $latex 2\beta_{1}=\alpha_{1}$, we have:
$latex 2(\beta_{1}+\beta)=\alpha+2\beta_{1}$
$latex 2\beta_{1}+2\beta=\alpha+2\beta_{1}$
$latex 2\beta=\alpha$
We have proved the theorem
See also
Interested in learning more about the inscribed angle theorem and other angles? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
