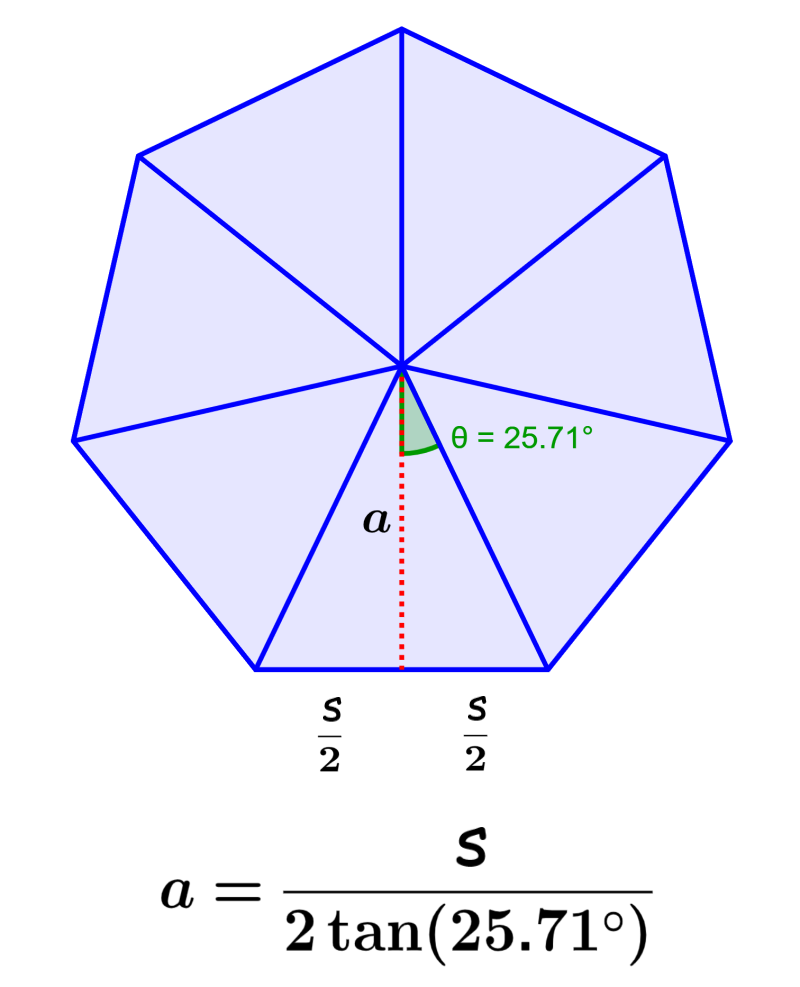
The apothem of a heptagon is the line that connects the center of the hexagon with one side of the heptagon perpendicularly. The apothem can also be considered as the length of the line that joins the center of the heptagon with the center of one of the sides. Knowing the length of the apothem, we can find the area of regular polygons using a simpler formula.
The length of the apothem can be calculated by dividing the heptagon into seven congruent triangles. Then we can use one of the triangles in conjunction with trigonometry to get a formula.
Formula to find the apothem of a heptagon
The apothem of a heptagon is the line that connects the center of the heptagon with one of its sides perpendicularly. One way to calculate the length of this line is to use trigonometry. For this, we start by dividing the heptagon into seven congruent triangles as in the following image:
We can see that the apothem is equal to the height of one of the triangles formed. This line divides the base into two equal parts. Furthermore, it also divides the triangle into two congruent right triangles.
We can determine the central angle of one of the small right triangles to use trigonometry and find the length of the apothem.
We know that the total central angle of the heptagon is 360°. Also, we know that we have 14 small right triangles, so the central angle of each is $latex 360 \div 14 = 25.71$°:
We can use the tangent function, which tells us that the tangent of an angle is equal to the opposite side on the adjacent side:
$$\tan(25.71)=\frac{\text{opposite}}{\text{adjacent}}$$
$$\tan(25.71)=\frac{\frac{s}{2}}{a}$$
$$\tan(25.71)=\frac{s}{2a}$$
| $$a=\frac{s}{2\tan(25.71)}$$ |
where s is the length of one of the sides of the heptagon.
Apothem of a heptagon – Examples with answers
The formula for the apothem of heptagons is used to solve the following examples. Each example has its respective solution, but it is advisable to try to solve the exercises yourself before looking at the solution.
EXAMPLE 1
A heptagon has sides of length 5 m. What is the length of its apothem?
Solution
We can use the apothem formula with length $latex s = 5$. Therefore, we have:
$latex a=\frac{s}{2\tan(25.71)}$
$latex a=\frac{5}{2\tan(25.71)}$
$latex a=5.19$
The length of the apothem is 5.19 m.
EXAMPLE 2
What is the length of the apothem of a heptagon that has sides of length 9 m?
Solution
We use the length $latex s=9$ in the apothem formula. Therefore, we have:
$latex a=\frac{s}{2\tan(25.71)}$
$latex a=\frac{9}{2\tan(25.71)}$
$latex a=9.35$
The length of the apothem is 9.35 m.
EXAMPLE 3
A heptagon has sides of length 12 m. What is its apothem?
Solution
We have the length $latex s = 12$. Using the apothem formula, we have:
$latex a=\frac{s}{2\tan(25.71)}$
$latex a=\frac{12}{2\tan(25.71)}$
$latex a=12.46$
The length of the apothem is 12.46 m.
EXAMPLE 4
A heptagon has an apothem of length 7.5 m. What is the length of its sides?
Solution
In this case, we start with the length of the apothem and want to find the length of the sides of the heptagon. Therefore, we use $latex a = 7.5$ and solve for s:
$latex a=\frac{s}{2\tan(25.71)}$
$latex 7.5=\frac{s}{2\tan(25.71)}$
$latex 7.5=\frac{s}{0.963}$
$latex s=7.5(0.963)$
$latex s=7.22$
The length of the sides of the heptagon is 7.22 m.
EXAMPLE 5
What is the length of the sides of a heptagon that has an apothem of length 20 m?
Solution
Again, we have the length of the apothem and we are going to find the length of the sides of the heptagon. Therefore, we use $latex a=20$ and solve for s:
$latex a=\frac{s}{2\tan(25.71)}$
$latex 20=\frac{s}{2\tan(25.71)}$
$latex 20=\frac{s}{0.963}$
$latex s=20(0.963)$
$latex s=19.26$
The length of the sides of the heptagon is 19.26 m.
Apothem of a heptagon – Practice problems
Use the following problems to practice applying the formula for the apothem of heptagons. Select an answer and click “Check” to verify that you chose the correct answer.
See also
Interested in learning more about parallelograms? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
