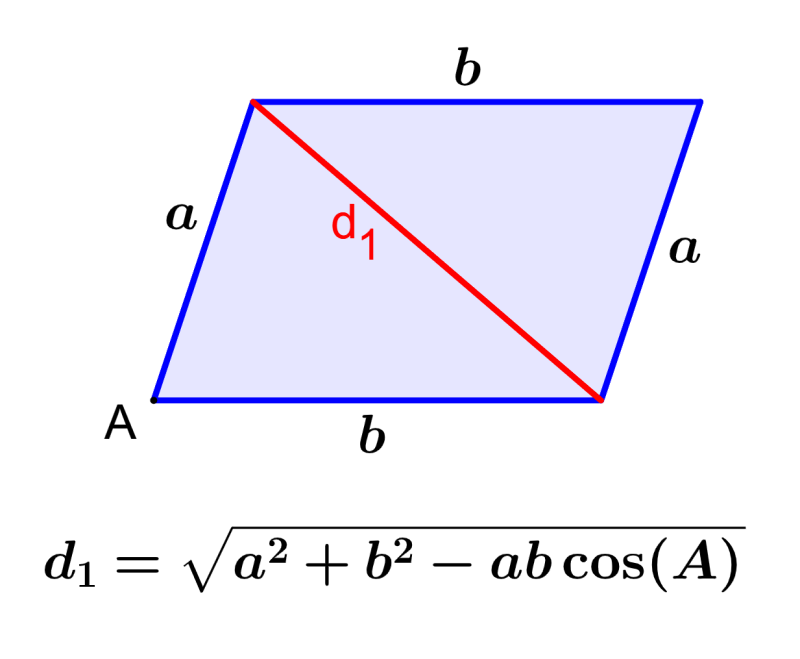
A parallelogram is a quadrilateral in which opposite sides are parallel and have the same length. Having opposite sides that are parallel and of equal lengths, it makes the angles on the opposite sides equal as well. The diagonals of a parallelogram are the segments that connect the opposite corners of the figure.
Here, we will learn about the formulas that we can use to calculate the diagonals of a parallelogram. In addition, we will look at some solved exercises in which we will apply these formulas.
Formula for the diagonal of a parallelogram
Let’s consider the following parallelogram:
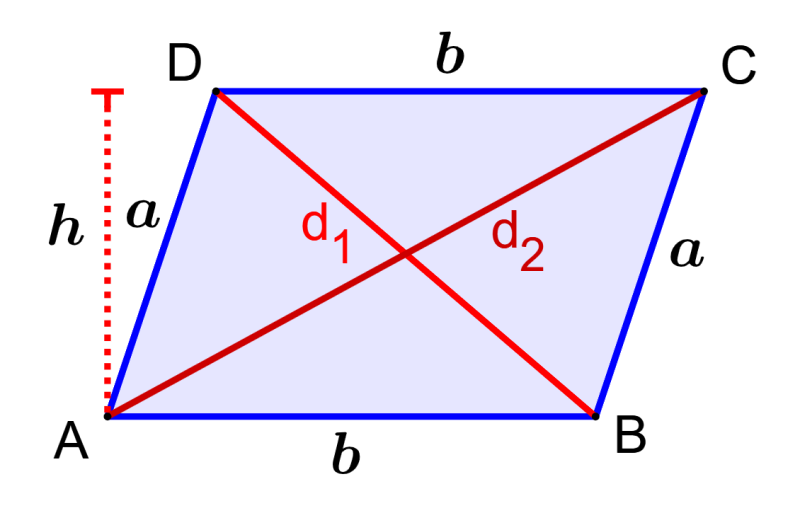
To find the diagonals of this parallelogram, we can use the following formulas:
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(A)}$
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}+2ab\cos(B)}$
$latex d_{2}=\sqrt{{{a}^2}+{{b}^2}+2ab\cos(A)}$
$latex d_{2}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(B)}$
$latex {{d_{1}}^2}+{{d_{2}}^2}=2({{a}^2}+{{b}^2})$
where,
- $latex d_{1}, ~d_{2}$ are the lengths of the diagonals
- $latex a, ~b$ are the lengths of the sides
Examples with answers of diagonal of a parallelogram
In the following examples, we use the diagonal formulas to find the lengths of the diagonals of the parallelograms. Try to solve the exercises yourself before looking at the answer.
EXAMPLE 1
Find the diagonal of a parallelogram with sides 6 m and 10 m and an angle of 30°.
Solution
We have the following values:
- Side 1, $latex a=6$ m
- Side 2, $latex b=10$ m
- Angle, $latex A=30$°
Therefore, we use the diagonal formula with these values:
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(A)}$
$$d_{1}=\sqrt{{{6}^2}+{{10}^2}-2(6)(10)\cos(30°)}$$
$latex d_{1}=\sqrt{36+100-2(6)(10)(0.5)}$
$latex d_{1}=\sqrt{136-60}$
$latex d_{1}=\sqrt{76}$
$latex d_{1}=8.72$
The diagonal has a length of 8.72 m.
EXAMPLE 2
What is the diagonal of a parallelogram with sides of length 10 m and 13 m and an angle that measures 40°?
Solution
We can identify the following:
- Side 1, $latex a=10$ m
- Side 2, $latex b=13$ m
- Angle, $latex A=40$°
Therefore, we plug these values into the formula:
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(A)}$
$$d_{1}=\sqrt{{{10}^2}+{{13}^2}-2(10)(13)\cos(40°)}$$
$$d_{1}=\sqrt{100+169-2(10)(13)(0.643)}$$
$latex d_{1}=\sqrt{269-167.18}$
$latex d_{1}=\sqrt{101.82}$
$latex d_{1}=10.09$
The diagonal has a length of 10.09 m.
EXAMPLE 3
A parallelogram has sides of length 8 m and 14 m with an angle measuring 45°. What is the length of its diagonal?
Solution
We have the following information:
- Side 1, $latex a=8$ m
- Side 2, $latex b=14$ m
- Angle, $latex A=45$°
Using these values in the formula, we have:
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(A)}$
$$d_{1}=\sqrt{{{8}^2}+{{14}^2}-2(8)(14)\cos(45°)}$$
$$d_{1}=\sqrt{64+196-2(8)(14)(0.707)}$$
$latex d_{1}=\sqrt{260-158.368}$
$latex d_{1}=\sqrt{101.632}$
$latex d_{1}=10.08$
The diagonal has a length of 10.08 m.
EXAMPLE 4
What is the length of the diagonal of a parallelogram that has sides of length 5 m and 7 m and an angle that measures 40°?
Solution
We have the following information:
- Side 1, $latex a=5$ m
- Side 2, $latex b=7$ m
- Angle, $latex A=40$°
Therefore, we use the diagonal formula with these values:
$latex d_{1}=\sqrt{{{a}^2}+{{b}^2}-2ab\cos(A)}$
$$d_{1}=\sqrt{{{5}^2}+{{7}^2}-2(5)(7)\cos(40°)}$$
$latex d_{1}=\sqrt{25+49-2(5)(7)(0.643)}$
$latex d_{1}=\sqrt{74-45.01}$
$latex d_{1}=\sqrt{76}$
$latex d_{1}=5.38$
The diagonal has a length of 5.38 m.
Diagonal of a parallelogram – Practice problems
Solve the following problems using the formula for the diagonal of a parallelogram. Select your answer and click “Check” to check your answer. Look at the solved examples above in case you need help.
See also
Interested in learning more about parallelograms? Take a look at these pages: