The cross-sections of a cube are two-dimensional figures obtained by the intersection of a plane with a cube. Depending on the orientation of the plane, we can obtain square, rectangular, triangular, and hexagonal cross-sections.
Here, we will learn about the cross-sections of a cube in detail using diagrams.
Square cross-section
When we cut a cube with a plane that is parallel to any of its six faces, the cross-section formed is square.
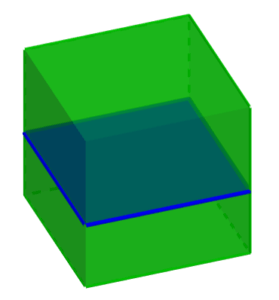
A cube has the main characteristic that all its sides have the same length. This means that all six faces of the cube have the same shape and the same area. Therefore, the area of a square cross-section of a cube is equal to the area of one of the faces.
EXAMPLE
If a cube has sides with a length of 5 m, what is the area of its square cross-section?
Solution: We know that the area of the square cross-section is equal to the area of one of the faces of the cube. Therefore, we just have to find the area of one face of the cube.
The area of a square is equal to A=s², where s is the length of one of the sides of the square. Then, we have:
A=s²
A=5²
A=25 m²
Rectangular cross-section
When we cut a cube with a plane through the diagonals of the faces, we get a rectangular cross-section.
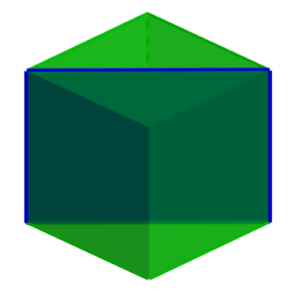
We can find the area of this rectangular cross-section by using the length of one of the sides as the height of the rectangle and the length of the diagonal of the cube’s faces as the base of the rectangle. Then, we just multiply these two lengths to get the area.
EXAMPLE
Find the area of the rectangular cross-section formed by the diagonals of the faces of a cube that has sides with a length of 10 m.
Solution: To find the cross-sectional area, we need to find the length of the diagonal of one face of the cube. For this, we use the Pythagorean theorem, where the legs are two sides of the cube and the hypotenuse is the diagonal of the face of the cube:
$latex c^2=a^2+b^2$
$latex c^2=10^2+10^2$
$latex c^2=100+100$
$latex c^2=200$
$latex c=\sqrt{200}$
$latex c=10~\sqrt{2}$
Now, we multiply that length by the length of one of the sides of the cube and we get $latex A=100~\sqrt{2}$ m².
Triangle cross-section
Square and rectangular cross-sections are formed when we intersect a cube with planes parallel to the faces of the cube. However, we can also obtain different cross-sections when we cut a cube with a plane that has an inclination with respect to its base.
For example, when the plane intersects three edges of the cube, we get a triangular cross-section. If the plane intersects three vertices of the cube, we get an equilateral triangle.
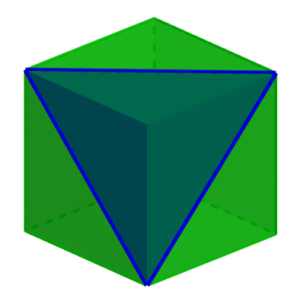
Hexagonal cross-section
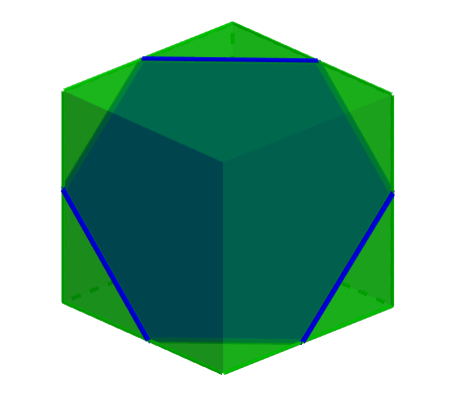
Cuando un plano interseca a un cubo en los puntos medios de seis de sus aristas, obtenemos una sección transversal hexagonal regular como se muestra en el diagrama.
También podemos obtener hexágonos irregulares cuando inclinamos al plano para que interseque en seis aristas fuera de los puntos medios.
See also
Interested in learning more about cross-sections of geometric figures? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
