The maximum point of a function is found using the derivative of the function. At the maximum point, the slope of the tangent line is equal to zero. Therefore, the maximum point is found by looking for the roots of the derivative. Then, we use the second derivative to confirm that the point is indeed a maximum.
Here, we will learn about the maximum point of a function. We will learn how to find the coordinates of this point, and we will solve some practice exercises.
What is the maximum point of a function?
The maximum point of a function is a stationary point where the value of y or the output value of the function is the maximum value that the function can reach.
In turn, let us remember that a stationary point is characterized in that the slope of the tangent line at that point is equal to zero. That is, at a maximum point we have $latex \frac{dy}{dx}=0$.
Let’s look at the following diagram, which represents a maximum point of a function:
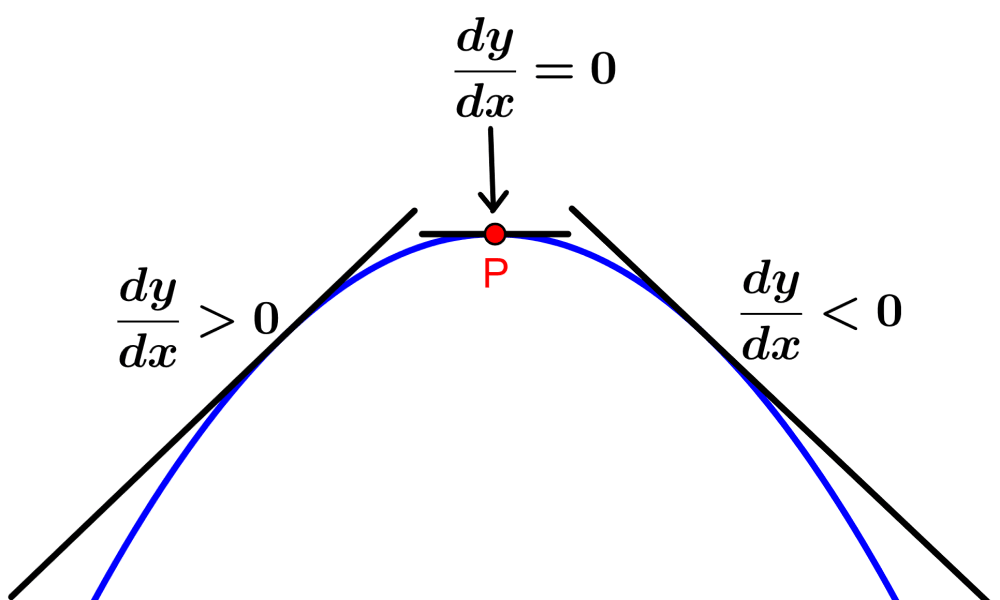
We can see that the slope of the curve is positive on the left-hand side of point P and negative on the right-hand side. That is, we have the following:
- On the left-hand side of P: $latex \frac{dy}{dx}>0$
- At point P: $latex \frac{dy}{dx}=0$
- On the right-hand side of P: $latex \frac{dy}{dx}<0$
This means that the second derivative of the function at a maximum point is negative because the derivative goes from positive to negative near the maximum point. That is, the derivative decreases from left to right there.
How to find the maximum point of a function?
The coordinates of the maximum point of a function can be found using the derivative of the function. Also, the second derivative can be used to confirm that the point is indeed a maximum point.
As we mentioned before, the slope of the tangent line at a stationary point is equal to zero. This means that we can find the stationary points by taking the roots of the derivative of the function.
Then, we can take the following steps to find the maximum point of a function:
Step 1: Get the derivative of the function.
Step 2: Form an equation with the derivative and solve for x. That is, we have $latex \frac{dy}{dx}=0$.
Step 3: The points found in step 2 are the stationary points. We use the second derivative to determine the nature of the points.
For the point to be a maximum, we must have $latex \frac{d^2y}{dx^2}<0$.
Step 4: Use the x-coordinate of the maximum point to find the y-coordinate of the point.
Maximum point of a function – Examples with answers
EXAMPLE 1
Find the maximum point of the function $latex f(x)=-x^2+2x$.
Solution
Step 1: Finding the derivative of the function, we have:
$latex f(x)=-x^2+2x$
$latex f'(x)=-2x+2$
Step 2: By forming an equation with the derivative, we can find the value of x:
$latex -2x+2=0$
$latex -2x=-2$
$latex x=1$
Step 3: In this case, we only have one value of x, so we only have one stationary point. We check if the point is a maximum using the second derivative:
$latex f^{\prime \prime}(x)=-2$
We have $latex f^{\prime \prime}(x)<0$, so we confirm that the point is a maximum
Step 4: The y-coordinate of the maximum point is:
$latex y=-x^2+2x$
$latex y=-(1)^2+2(1)$
$latex y=1$
The maximum point has the coordinates (1, 1).
EXAMPLE 2
What are the coordinates of the maximum point of the function $latex f(x)=-2x^2-8x$?
Solution
Step 1: The derivative of the function is:
$latex f(x)=-2x^2-8x$
$latex f'(x)=-4x-8$
Step 2: Finding the value of x, we have:
$latex -4x-8=0$
$latex -4x=8$
$latex x=-2$
Step 3: We use the second derivative to check if the point is a maximum point:
$latex f^{\prime \prime}(x)=-4$
We have $latex f^{\prime \prime}(x)<0$, so we confirm it is a maximum point.
Step 4: We use the function with the value $latex x=-2$ to find the y-coordinate:
$latex y=-2x^2-8x$
$latex y=-2(-2)^2-8(-2)$
$latex y=-8+16$
$latex y=8$
The maximum point has the coordinates (-2, 8).
EXAMPLE 3
Find the coordinates of the maximum point of the function $latex f(x)=-x^3+3x$.
Solution
Step 1: The derivative of the function is:
$latex f(x)=-x^3+3x$
$latex f'(x)=-3x^2+3$
Step 2: Finding the values of x, we have:
$latex -3x^2+3=0$
$latex -3x^2=-3$
$latex x=\sqrt{3}$
$latex x=1~~$ or $latex ~~x=-1$
Step 3: In this case, we have two values of x, so we use the second derivative to determine which is the maximum point:
$latex f^{\prime \prime}(x)=-6x$
When $latex x=1$, we have $latex f^{\prime \prime}(x)<0$, and when $latex x=-1$, we have $latex f^{\prime \prime}(x) >0$. So $latex x=1$ is the maximum point.
Step 4: The y-coordinate of the maximum point is:
$latex y=-x^3+3x$
$latex y=-(1)^3+3(1)$
$latex y=2$
The maximum point has the coordinates (1, 2).
EXAMPLE 4
If we have the function $latex f(x)=2x^3-6x$, what is its maximum point?
Solution
Step 1: Differentiating the function, we have:
$latex f(x)=2x^3-6x$
$latex f'(x)=6x^2-6$
Step 2: Finding the values of x, we have:
$latex 6x^2-6=0$
$latex 6x^2=6$
$latex x^2=\sqrt{1}$
$latex x=1~~$ or $latex ~~x=-1$
Step 3: We have two stationary points, so we use the second derivative to determine which is the maximum point:
$latex f^{\prime \prime}(x)=12x$
For $latex x=1$, we have $latex f^{\prime \prime}(x)>0$ and for $latex x=-1$, we have $latex f^{\prime \prime}(x)< 0$, so $latex x=-1$ is the maximum point.
Step 4: The y-coordinate of the maximum point is:
$latex y=2x^3-6x$
$latex y=2(-1)^3-6(-1)$
$latex y=4$
The maximum point has the coordinates (-1, 4).
EXAMPLE 5
What is the maximum point of $latex f(x)=\frac{1}{3}x^3+x^2-3x$?
Solution
Step 1: The derivative of the function is:
$latex f(x)=\frac{1}{3}x^3+x^2-3x$
$latex f'(x)=x^2+2x-3$
Step 2: We can form an equation with the derivative and solve using factorization:
$latex x^2+2x-3=0$
$latex (x+3)(x-1)=0$
$latex x=-3~~$ or $latex ~~x=1$
Step 3: We use the second derivative to determine the maximum point:
$latex f^{\prime \prime}(x)=2x+2$
When $latex x=-3$, we have $latex f^{\prime \prime}(x)<0$ and when $latex x=1$, we have $latex f^{\prime \prime}(x)> 0$. So the maximum point is at $latex x=-3$.
Step 4: The y-coordinate of the maximum point is:
$latex f(x)=\frac{1}{3}x^3+x^2-3x$
$latex f(x)=\frac{1}{3}(-3)^3+(-3)^2-3(-3)$
$latex y=9$
The maximum point has the coordinates (-3, 9).
EXAMPLE 6
In the following right triangle ABC, the lengths of the sides AB and BC vary in such a way that their sum is always equal to 6 cm. Find the maximum area of triangle ABC.
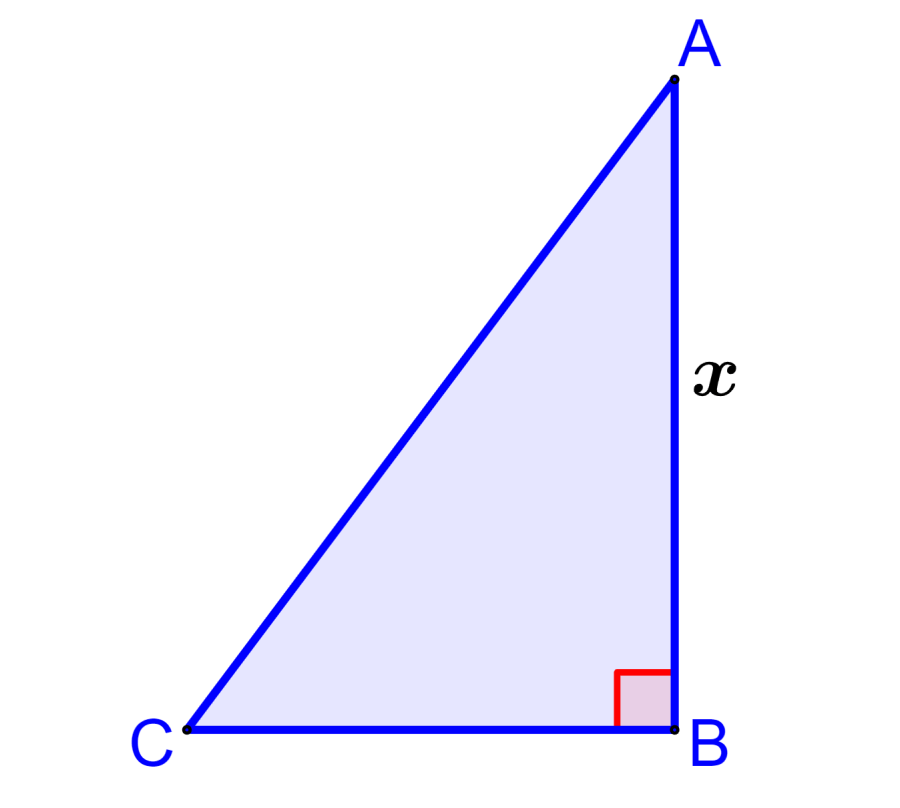
Solution
To solve this problem, we need to start by finding an equation for the area of the triangle in terms of the side x.
We know that
$latex AB+BC=6$
$latex x+BC=6$
$latex BC=6-x$
Now the area of the triangle is:
$latex A=\frac{1}{2}\times BC \times AB$
$latex A=\frac{1}{2}(6-x)x$
$latex A=3x-\frac{x^2}{2}$
Now, we use the steps to find the maximum point of the found function.
Step 1: The derivative is:
$latex A(x)=3x-\frac{x^2}{2}$
$latex A'(x)=3-x$
Step 2: The value of x is:
$latex 3-x=0$
$latex x=3$
Step 3: We use the second derivative to verify that the point is a maximum:
$latex A^{\prime \prime}(x)=-1$
We have $latex f^{\prime \prime}(x)<0$, so we confirm that the point is a maximum. This means that the area is maximum with this value of x.
Step 4: We use $latex x=3$ to find the maximum area:
$latex A=3x-\frac{x^2}{2}$
$latex A=3(3)-\frac{(3)^2}{2}$
$latex A=\frac{9}{2}=4.5$
The maximum area of the triangle is 4.5 cm².
Maximum point of a function – Practice problems
Find the maximum point of the function $latex f(x)=2x^3-24x-10$.
Write the coordinates in the input box.
See also
Interested in learning more about derivatives and stationary points? You can look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
