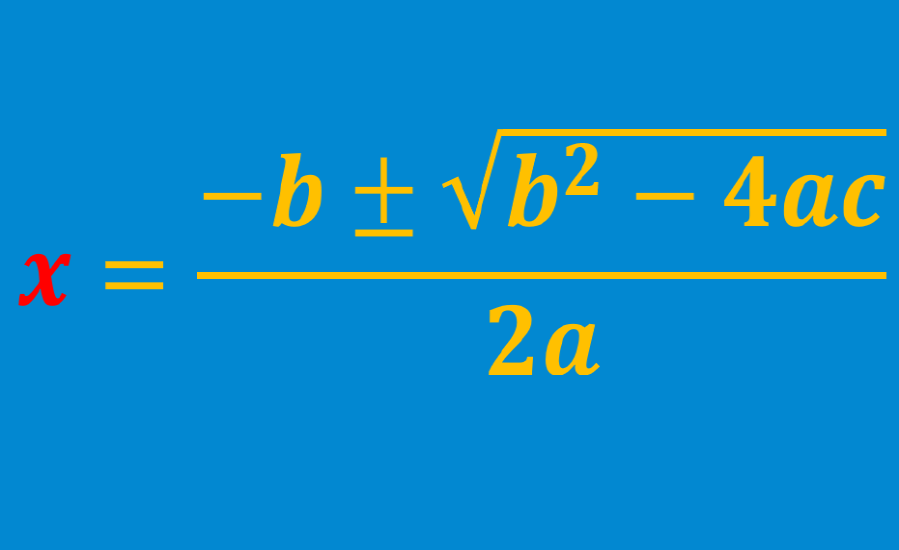An easy way to solve quadratic equations is through factoring. However, many of the quadratic equations cannot be factored easily. In these cases, we can use the general quadratic formula to solve any quadratic equation.
In this article, we will learn how to derive the quadratic formula step by step. Then, we will use it to solve quadratic equations.
How to derive the quadratic formula?
The quadratic formula $latex x=\frac{{-b\pm \sqrt{{{{b}^{2}}-4ac}}}}{{2a}}$ is derived using steps similar to those we use to complete the square. This formula takes into account the fact that any equation of the form $latex a{{x}^2}+bx+c=0$ can be solved to find its roots.
The roots of the equation are points where the graph of the equation crosses the x-axis.
Therefore, to find the roots of the equation $latex a{{x}^2}+bx+c=0$, we are going to use the steps used in completing the square to obtain the general formula:
Step 1: Write the equation in the general form $latex a{{x}^2}+bx+c=0$, where a, b, and c are real numbers, and a≠0.
Step 2: Move the constant c to the right hand side of the equation:
$latex a{{x}^2}+bx+c-c=0-c$
$latex a{{x}^2}+bx=-c$
Step 3: Divide the equation by the coefficient a:
$latex \frac{1}{a}\left( {a{{x}^{2}}+bx=-c} \right)$
$latex {{x}^{2}}+\frac{b}{a}x=-\frac{c}{a}$
Step 4: Identify the coefficient $latex \frac{b}{a}$, divide it by 2, square it and simplify it:
$latex {{\left( {\frac{{\frac{b}{a}}}{2}} \right)}^{2}}={{\left( {\frac{b}{{2a}}} \right)}^{2}}=\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}$
Step 5: Add the result of step 4 to both sides of the equation:
$latex {{x}^{2}}+\frac{b}{a}x+\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}=-\frac{c}{a}+\frac{b}{{4{{a}^{2}}}}$
Step 6: Simplify the right hand side of the equation:
$latex {{x}^{2}}+\frac{b}{a}x+\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}=-\frac{c}{a}\left( {\frac{{4a}}{{4a}}} \right)+\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}$
$latex =-\frac{{4ac}}{{4{{a}^{2}}}}+\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}$
$latex {{x}^{2}}+\frac{b}{a}x+\frac{{{{b}^{2}}}}{{4{{a}^{2}}}}=\frac{{{{b}^{2}}-4ac}}{{4{{a}^{2}}}}$
Step 7: Form the square of the binomial in the expression on the left:
$latex {{\left( {x+\frac{b}{{2a}}} \right)}^{2}}=\frac{{{{b}^{2}}-4ac}}{{4{{a}^{2}}}}$
Step 8: Take the square root of both sides and simplify without forgetting the ± sign on the right side:
$latex x+\frac{b}{{2a}}=\pm \sqrt{{\frac{{{{b}^{2}}-4ac}}{{4{{a}^{2}}}}}}$
Step 9: Isolate the x on the left and simplify:
$latex x+\frac{b}{{2a}}-\frac{b}{{2a}}=\pm \frac{{\sqrt{{{{b}^{2}}-4ac}}}}{{2a}}-\frac{b}{{2a}}$
$latex x=-\frac{b}{{2a}}\pm \frac{{\sqrt{{{{b}^{2}}-4ac}}}}{{2a}}$
$latex x=\frac{{-b\pm \sqrt{{{{b}^{2}}-4ac}}}}{{2a}}$
How to solve quadratic equations with the quadratic formula?
To solve quadratic equations with the general quadratic formula, we have to start by organizing the equation in the form $latex a{{x}^2}+bx+c=0$. Once we have the equation written that way, we simply plug in the coefficients a, b, and c into the quadratic formula:
$latex x=\frac{{-b\pm \sqrt{{{{b}^{2}}-4ac}}}}{{2a}}$
Something important that we must not forget is the ± sign since in this way we will obtain both solutions to the quadratic equation.
EXAMPLE 1
Solve the equation $latex {{x}^2}+3x-4=0$.
Solution: Using $latex a=1$, $latex b=3$ and $latex c=-4$, we have:
$latex x=\frac{{-\left( 3 \right)\pm \sqrt{{{{{\left( 3 \right)}}^{2}}-4\left( 1 \right)\left( {-4} \right)}}}}{{2\left( 1 \right)}}$
$latex =\frac{{-3\pm \sqrt{{9+16}}}}{2}$
$latex =\frac{{-3\pm \sqrt{{25}}}}{2}$
$latex =\frac{{-3\pm 5}}{2}$
$latex =\frac{{-3-5}}{2},~~\frac{{-3+5}}{2}$
$latex =\frac{{-8}}{2},~\frac{2}{2}=-4,~1$
Therefore, the solutions are $latex x=-4$ and $latex x=1$.
EXAMPLE 2
Solve the equation $latex 9{{x}^2}+12x+4=0$.
Solution: Using $latex a=9$, $latex b=12$ and $latex c=4$ with the quadratic formula, we have:
$latex x=\frac{{-\left( 12 \right)\pm \sqrt{{{{{\left( 12 \right)}}^{2}}-4\left( 9 \right)\left( {4} \right)}}}}{{2\left( 9 \right)}}$
$latex =\frac{{-12\pm \sqrt{{144-144}}}}{18}$
$latex =\frac{{-12\pm \sqrt{{0}}}}{18}$
$latex =\frac{{-12\pm 0}}{18}$
$latex =\frac{{-12}}{18}=-\frac{{2}}{3}$
In this case, we got a zero within the square root, therefore we don’t get any change from adding or subtracting zero. This means that the only solution is $latex x=-\frac{{2}}{3}$.
EXAMPLE 3
Solve the equation $latex 3{{x}^2}+4x+2=0$.
Solution: Using $latex a=3$, $latex b=4$ and $latex c=2$ with the quadratic equation, we have:
$latex x=\frac{{-\left( 4 \right)\pm \sqrt{{{{{\left( 4 \right)}}^{2}}-4\left( 3 \right)\left( {2} \right)}}}}{{2\left( 3 \right)}}$
$latex =\frac{{-4\pm \sqrt{{16-24}}}}{6}$
$latex =\frac{{-4\pm \sqrt{{-8}}}}{6}$
We can see that we got a negative number inside the square root. This has no solution if we are restricted to real numbers. If you haven’t learned about complex numbers yet, then we would stop here and conclude that this equation “has no solution.” However, this can be solved with knowledge of complex numbers:
$latex =\frac{{-4\pm \sqrt{{-8}}}}{6}=\frac{{-4\pm 2\sqrt{{-2}}}}{6}$
$latex =\frac{{-2\pm \sqrt{{2}}i}}{3}$
$latex =-\frac{2}{3}\pm \frac{{\sqrt{2}}}{3}i$
Therefore, depending on whether we only consider real numbers or we also consider complex numbers, we have the solutions:
Only real numbers: no solution.
Complex numbers: the solution is $latex =-\frac{2}{3}\pm \frac{{\sqrt{2}}}{3}i$.
Quadratic formula – Practice problems
See also
Interested in learning more about algebraic expressions and factoring? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
