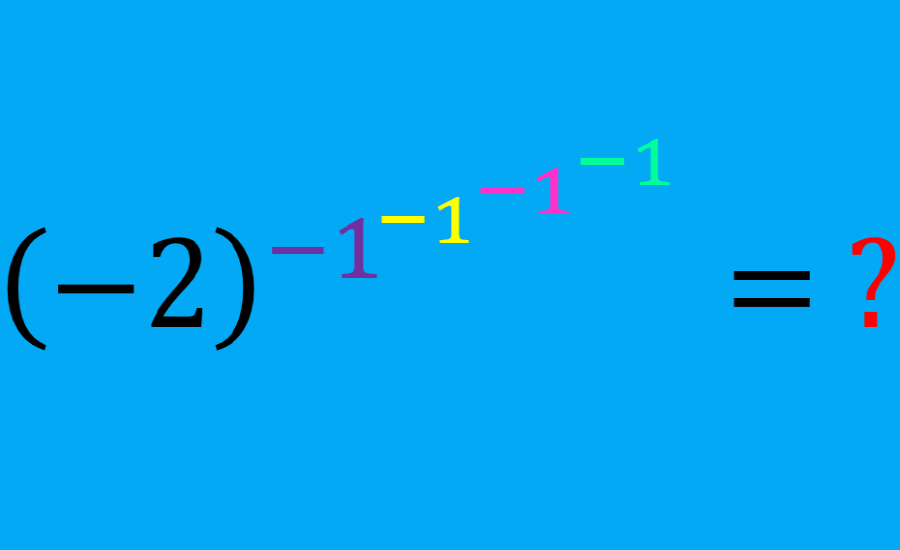When we have the power of the power in exponential expressions, we find the new power by multiplying the two powers. For example, in the following expression, x squared is being raised to the power of 5, so we multiply 2 and 5 to find the new power.
$latex \left(x^2 \right)^5=x^{(2)(5)}=x^{10}$
Power of a power rule
Remember that exponential expressions have two parts. The first part is the base and the second part is the exponent as can be seen in the illustration:
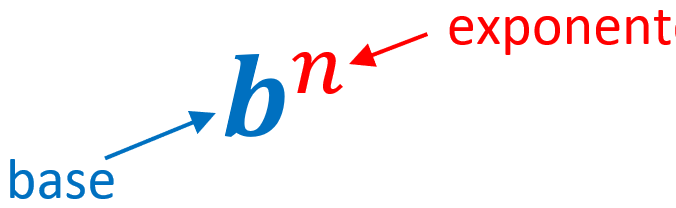
- The exponent is the number that indicates how many times the base will be multiplied by itself.
- The base is the number or variable that is being multiplied repeatedly.
The power of a power rule tells us that when we have an exponential expression raised to a power, we simply have to copy the base and multiply the exponents. Here, we assume that the base is nonzero and that the exponents are integers:
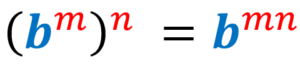
Power of a power – Examples with answers
The following exercises show us how we can solve expressions that have a power of a power:
EXAMPLE 1
Each factor within the parentheses is raised to the exponent that is outside the parentheses:
- $latex {{\left( {{{3}^{4}}} \right)}^{5}}={{3}^{{\left( 4 \right)\left( 5 \right)}}}={{3}^{{20}}}$
- $latex {{\left( {{{4}^{{-2}}}} \right)}^{3}}={{4}^{{\left( {-2} \right)\left( 3 \right)}}}={{4}^{{-6}}}=\frac{1}{{{{4}^{6}}}}$
- $latex {{\left( {{{x}^{3}}} \right)}^{5}}={{x}^{{\left( 3 \right)\left( 5 \right)}}}={{x}^{{15}}}$
- $latex {{\left( {{{x}^{2}}{{y}^{4}}} \right)}^{3}}={{x}^{{\left( 2 \right)\left( 3 \right)}}}{{y}^{{\left( 4 \right)\left( 3 \right)}}}={{x}^{6}}{{y}^{{12}}}$
EXAMPLE 2
In the following exercise, we use the order of operations. First we raise the expressions inside the parentheses to their powers. Then, we multiply the two expressions. We apply the product rule to simplify the expressions by combining equal bases and adding exponents:
$latex {{\left( {2{{x}^{2}}{{y}^{4}}} \right)}^{3}}{{\left( {4{{x}^{3}}{{y}^{2}}} \right)}^{2}}$
$latex =\left( {{{2}^{3}}{{x}^{{2\times 3}}}{{y}^{{4\times 3}}}} \right)\left( {{{4}^{2}}{{x}^{{3\times 2}}}{{y}^{{2\times 2}}}} \right)$
$latex =\left( {8{{x}^{6}}{{y}^{{12}}}} \right)\left( {16{{x}^{6}}{{y}^{4}}} \right)$
$latex =128{{x}^{{12}}}{{y}^{{16}}}$
EXAMPLE 3
The following exercise is similar to the previous one, but with negative exponents:
$latex {{\left( {{{x}^{2}}{{y}^{3}}} \right)}^{-3}}{{\left( {{{x}^{-3}}{{y}^{-4}}} \right)}^{-4}}$
$latex =\left( {{{x}^{{2\times -3}}}{{y}^{{3\times -3}}}} \right)\left( {{{x}^{{-3\times -4}}}{{y}^{{-4\times -4}}}} \right)$
$latex =\left( {{{x}^{-6}}{{y}^{{-9}}}} \right)\left( {{{x}^{12}}{{y}^{16}}} \right)$
$latex ={{x}^{{6}}}{{y}^{{7}}}$
Power of a power – Practice problems
See also
Interested in learning more about exponents? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
