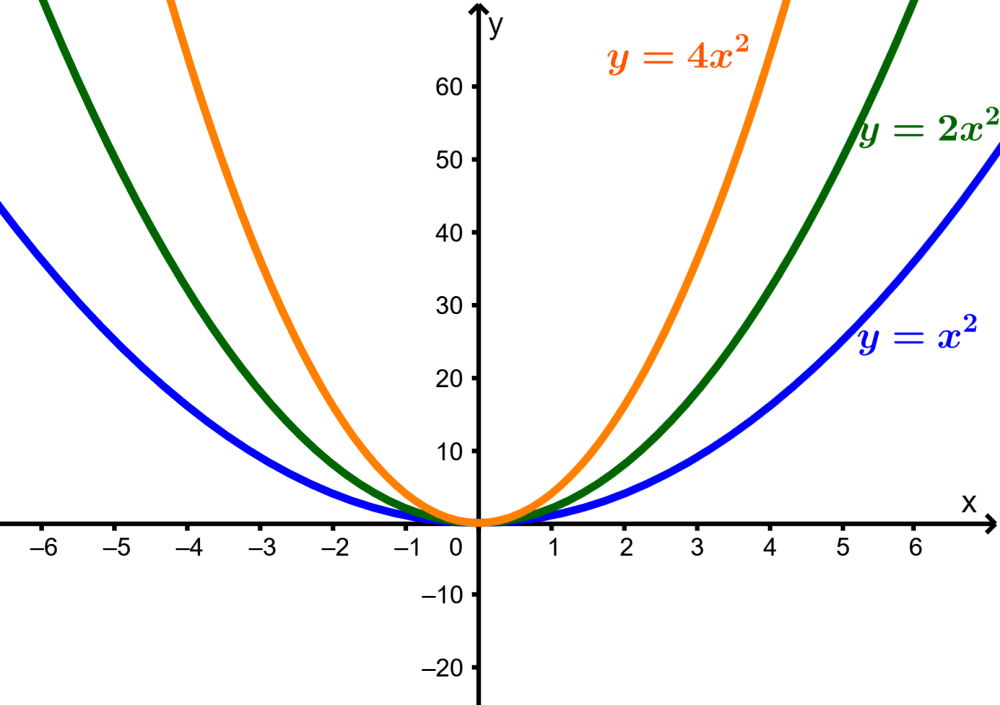
Quadratic functions are polynomial functions that have a maximum degree of two. The graphs of quadratic functions are called parabolas and have a “U” shape. Quadratic functions can be used to model various situations in everyday life such as the parabolic motion produced by throwing objects into the air.
Here, we will look at a summary of quadratic functions along with several examples with answers that will help us to better understand the concepts.
Summary of quadratic functions
A quadratic function has the form $latex f(x)=a{{x}^2}+bx+c$, where a, b, and c are real numbers and a is nonzero.
The graph of a quadratic function is a curve called a parabola. The parabolas open up or down and have different “widths” or “slopes,” but they all have the same basic U shape. The following are graphs of parabolas:
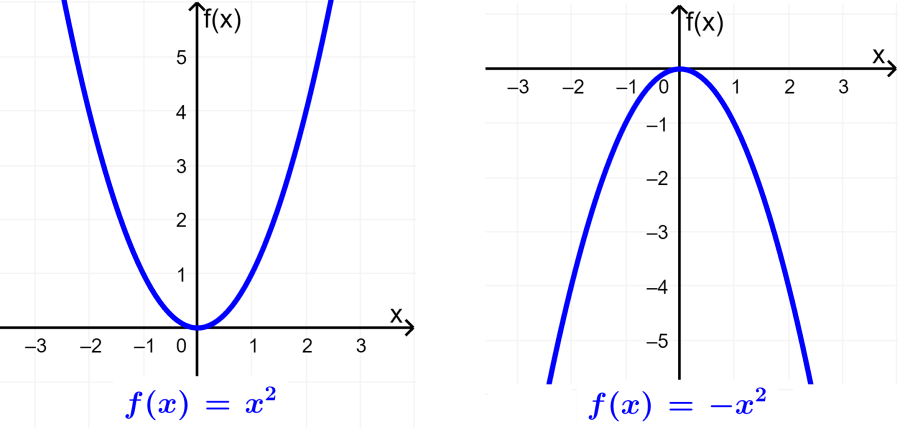
All parabolas are symmetric with respect to a line called the axis of symmetry. A parabola intersects its axis of symmetry at a point called the vertex of the parabola.
We know that two points determine a line. That is, if we have two points in the plane, there is only one line that contains both points. There is a similar statement for points and quadratic functions.
Given three points in the plane that have different coordinates and are not located on a straight line, there is exactly one quadratic function, which produces a graph that contains all three points.
Quadratic functions can be graphed by finding several points that are part of the curve and using their axis of symmetry.
We can find the roots of a quadratic function by using its factored form and remembering that if its factored form is $latex f(x)=(x-a)(x-b)$, then its roots are $latex x=a$ and $latex x=b$.
Examples with answers of quadratic function problems
The following quadratic function examples have their respective solution which details the process and reasoning used to arrive at the answer. Try to solve the exercises yourself before looking at the solution.
EXAMPLE 1
Graph the quadratic function $latex {{x}^2}+2$.
Solution
We can use three points to graph the quadratic function. We choose the values $latex x=0$, $latex x = 1$ and $latex x = 2$. Therefore, we have:
- When $latex x = 0$, we have $latex f(0)=0+2=2$
- When $latex x = 1$, we have $latex f(1)=1 + 2=3$
- When $latex x = 2$, we have $latex f(2)=4+2=6$
Then, we graph those points and draw a curve that passes through them and produce a reflection in their axis of symmetry:
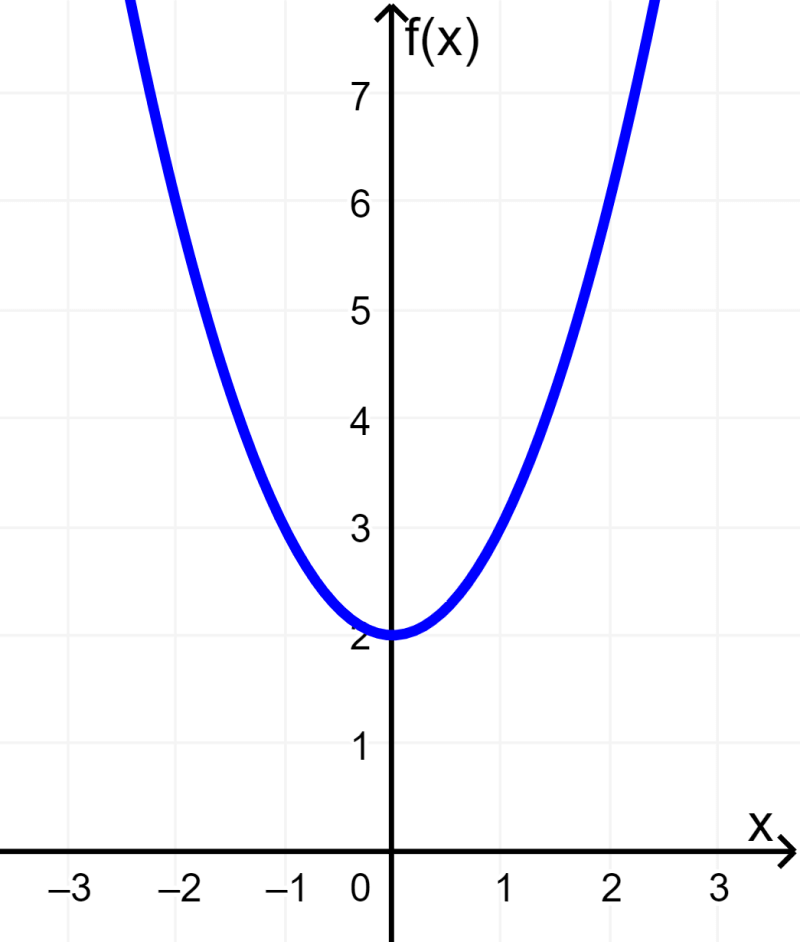
Alternatively, we can recognize that this graph is the graph of a standard quadratic function $latex f(x)={{x}^2}$ with a vertical translation of 2 units upwards.
EXAMPLE 2
Graph the quadratic function $latex {{x}^2}-1$.
Solution
Again, we can use the values $latex x = 0$, $latex x=1$, and $latex x=2$ to get three points. Therefore, we have:
- When $latex x=0$, we have $latex f(0)=0-1 = -1$
- When $latex x=1$, we have $latex f(1)=1-1=0$
- When $latex x=2$, we have $latex f(2)=4-1=3$
We plot those points and draw a curve. Then, we replicate that curve on its axis of symmetry:
Alternatively, it is possible to recognize that this graph is a standard quadratic $latex f(x)={{x}^2}$ with a vertical translation of 1 unit downwards.
EXAMPLE 3
Graph the quadratic function $latex -{{x}^2}+3$.
Solution
Using the values $latex x=0$, $latex x=1$ and $latex x=2$, we have:
- When $latex x=0$, we have $latex f(0)=0+3=3$
- When $latex x=1$, we have $latex f(1)=-1+3=2$
- When $latex x=2$, we have $latex f(2)=-4+2=-2$
Now, we plot the points and draw a curve. Then, we replicate this on its axis of symmetry:
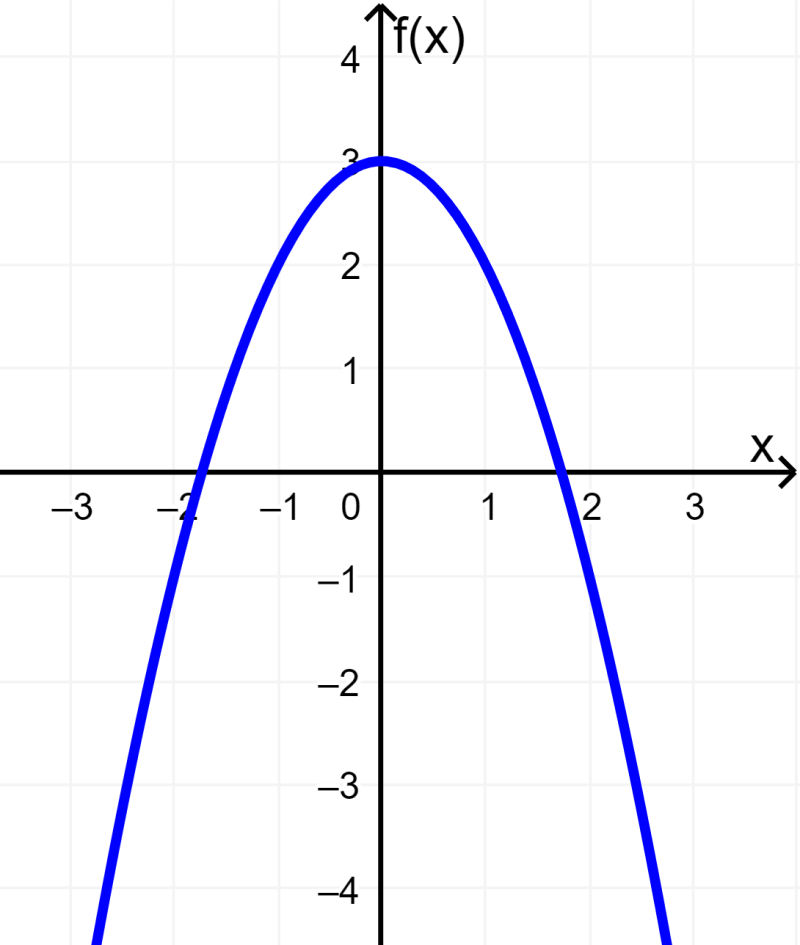
Alternatively, it is possible to recognize this function is a standard quadratic function $latex f(x)= {{x}^2}$ with a reflection on the y-axis and a vertical translation of 3 units upwards.
EXAMPLE 4
Find the roots of the quadratic function if they exist:
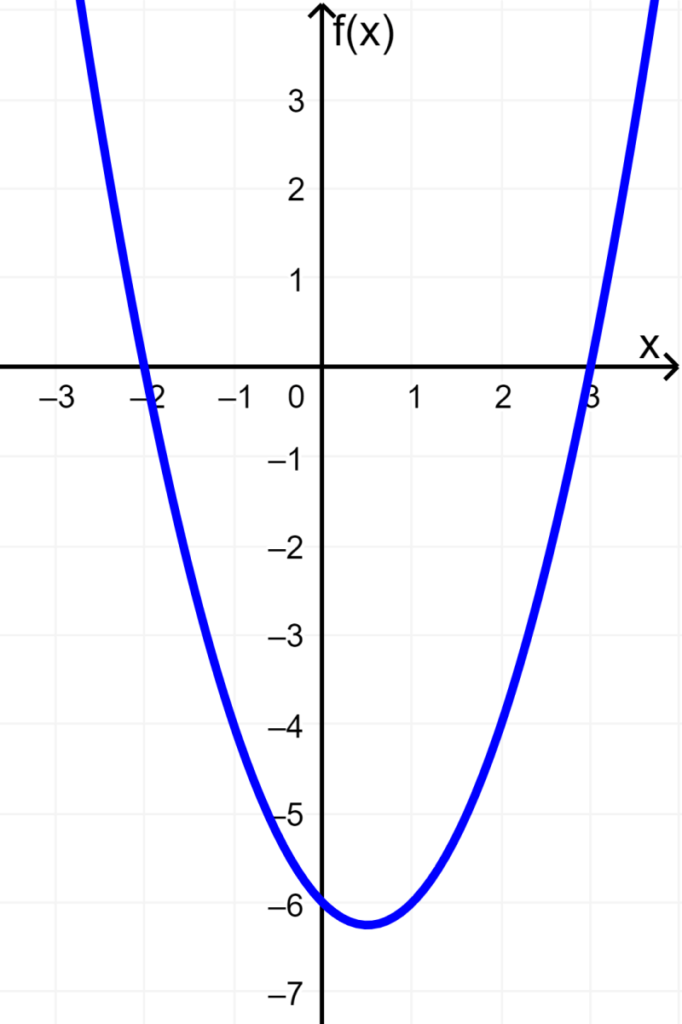
Solution
The roots of a quadratic function are the points where the graph crosses the x-axis. In this case, we see that the graph of the quadratic function crosses the x-axis at the points $latex x=-2$ and $latex x=3$, so these are the roots.
EXAMPLE 5
Find the roots of the following quadratic function if they exist:
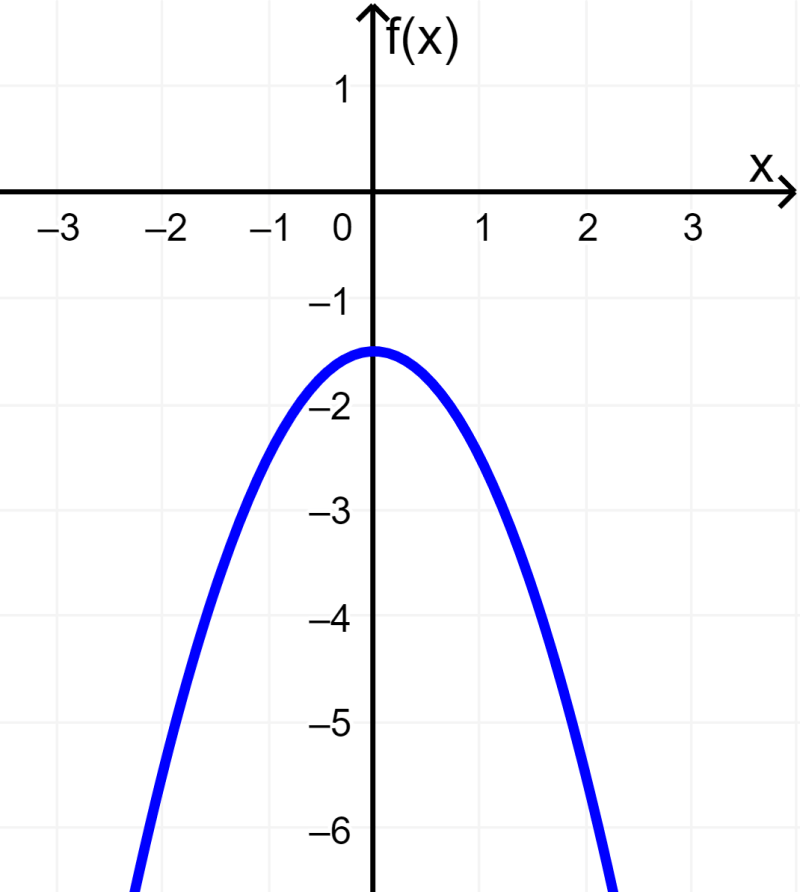
Solution
We see that in this case, the graph of the quadratic function does not cross the x-axis, so the function does not have real roots.
All quadratic functions have roots if we are not restricted to real numbers and can use imaginary numbers. However, in most cases, we can say that this function has no real roots.
EXAMPLE 6
Use the factored form to find the roots of the quadratic function $latex f(x)={{x}^2}+5x+6$.
Solution
To find the factored form of the quadratic function, we have to find two numbers so that their sum equals 5 and their product equals 6.
Two numbers that meet these conditions are 2 and 3 since $latex 2+3=5$ and $latex 2 \times 3=6$. Thus, to find the roots of the quadratic function, we rewrite the function in its factored form using the found numbers and set equal to zero:
$latex f(x)=(x+2)(x+3)=0$
The roots are $latex x=-2$ and $latex x=-3$.
EXAMPLE 7
Find the roots of the quadratic function $latex f(x)={{x}^2}+2x-8$.
Solution
In this case, we have to find two numbers so that their sum equals 2 and their product equals -8.
We can meet these conditions with the numbers 4 and -2 since $latex 4-2 = 2$ and $latex 4 \times -2=-8$. Therefore, we find the roots of the quadratic function by rewriting the function in its factored form using the found numbers and setting zero:
$latex f(x)=(x+4)(x-2)=0$
The roots are $latex x=-4$ and $latex x=2$.
EXAMPLE 8
Use the factored form to find the roots of the quadratic function $latex f(x)=2{{x}^2}+4x-6$.
Solution
We can start by taking the common factor 2 out of the function:
$latex f(x)= 2{{x}^2}+4x-6$
$latex =2({{x}^ 2} + 2x-3)$
Now, we find two numbers so that their sum equals 2 and their product equals -3.
The numbers 3 and -1 meet these conditions since $latex 3-1=2$ and $latex 3 \times -1=-3$. Thus, we rewrite the function in its factored form using the found numbers and set equal to zero:
$latex f(x)=2(x+2)(x-1)=0$
The roots are $latex x=-2$ and $latex x=1$.
Quadratic function problems – Practice
Test your knowledge of quadratic functions with the following problems. Choose an answer and check it to see that you selected the correct answer.
See also
Interested in learning more about quadratic functions? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
