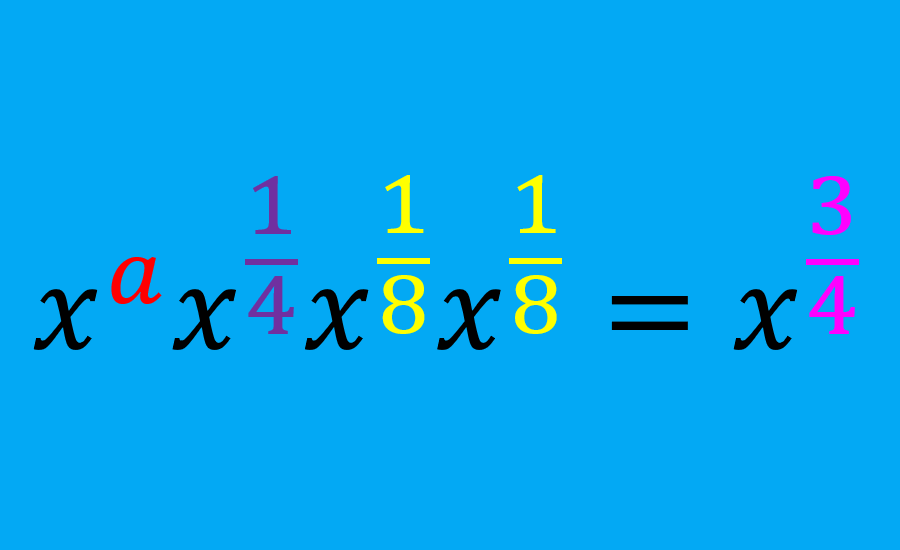Exponents are simpler ways to write repeated multiplication. However, even with exponents, algebraic expressions can become long and tedious. The laws of exponents allow us to simplify algebraic expressions that contain operations with exponents. Knowledge of these laws of exponents will make our study of algebra more productive.
Here, we will look at a summary of the seven laws of exponents along with some examples to understand the reasoning used when simplifying algebraic expressions.
Summary of laws of exponents
The laws of exponents tell us how to solve equations or simplify expressions that contain exponents. Exponents are values that tell us how many times we must multiply a number by itself. For example, if we have $latex {{5}^3}$, this means that we multiply 5 by itself 3 three times:
$latex {{5}^3}=5 \times 5 \times 5 = 125$
The number being raised to an exponent is called the base. In this case, the base is 5. The small number is the exponent. In this case, the exponent is 3.
There are different types of exponential expressions, which seem tedious at first glance. However, there are certain strategies that can be used to make the laws of exponents easy to follow.
There are seven laws of exponents that help us simplify exponential expressions. Each law shows how to solve different types of mathematical operations such as adding, subtracting, multiplying, and dividing exponents.
In the following laws, the letters a and b represent nonzero real numbers, and m and n represent integer numbers:
1) Law of zero exponents:
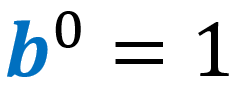
2) Law of negative exponents
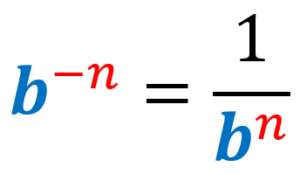
3) Law of the product of exponents
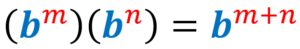
4) Law of the quotient of exponents
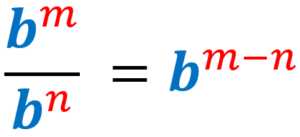
5) Law of power of a power
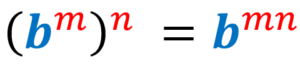
6) Law of power of a product
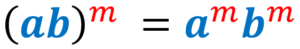
7) Law of the quotient of a product
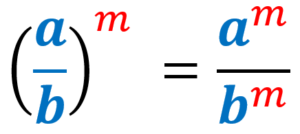
Laws of exponents – Examples with answers
The following examples apply the laws of exponents to simplify algebraic expressions. Try to solve the exercises yourself. However, each example has a detailed solution so that you can follow the reasoning used in each problem.
EXAMPLE 1
Find the result of $latex {{8}^{- 2}}$.
Solution
To solve for the negative exponent, we apply the law of negative exponents, which tells us that we can change an exponent from negative to positive by taking the reciprocal of its base:
$latex 8^{-2}=\frac{1}{{{8}^2}}$
Now, we square 8 to simplify:
$latex \frac{1}{{{8}^2}}=\frac{1}{64}$
EXAMPLE 2
Simplify the expression $latex {{({{2}^3})}^{-2}}$.
Solution
Here, we apply the power of a power law, which tells us that we have to multiply the exponents:
$latex {{({{2}^3})}^{-2}}={{2}^{-6}}$
Again, we apply the law of negative exponents to change the exponent 6 negative to 6 positive:
$latex {{2}^{-6}}=\frac{1}{{{2}^6}}$
Simplifying, we have:
$latex \frac{1}{{{2}^6}}=\frac{1}{64}$
EXAMPLE 3
Find the result of $latex \frac{{{5}^6}}{{{5}^4}}$.
Solution
We apply the quotient law to simplify the division of powers. Therefore, we have the base 4 and we subtract the exponent of the denominator from the exponent of the numerator:
$latex \frac{{{5}^6}}{{{5}^4}}={{5}^{6-4}}$
$latex ={{5}^2}$
Applying the exponent we have:
$latex {{5}^2}=25$
EXAMPLE 4
Find the result of $latex \frac{{{5}^4}\times {{3}^4}}{{{15}^2}}$.
Solution
We have different bases in the numerator, so we cannot simplify. However, we note that the base can be rewritten since 15 equals 5×3:
$latex \frac{{{5}^4}\times {{3}^4}}{{{15}^2}}=\frac{{{5}^4}\times {{3}^4}}{{{(5\times 3)}^2}}$
$latex =\frac{{{5}^4}\times {{3}^4}}{{{5}^2}\times {{3}^2}}$
Now, we apply the quotient law to both the power with base 5 and the power with base 3:
$latex \frac{{{5}^4}\times {{3}^4}}{{{5}^2}\times {{3}^2}}={{5}^{4-2}}\times {{3}^{4-2}}$
$latex ={{5}^2}\times {{3}^2}$
$latex =25\times 9$
$latex =225$
EXAMPLE 5
Simplify the expression $latex {{\left(\frac{2}{{{3}^2}} \right)}^{-3}} \times \left(\frac{{{2}^3}}{{{3}^2}} \right)$.
Solution
We start by changing the negative exponent to positive by flipping the fraction:
$latex {{\left(\frac{2}{{{3}^2}} \right)}^{-3}} \times \left(\frac{{{2}^3}}{{{3}^2}} \right)={{\left(\frac{{{3}^2}}{2} \right)}^3} \times \left(\frac{{{2}^3}}{{{3}^2}} \right)$
Now, we apply the law of the power of a power:
$latex {{\left(\frac{{{3}^2}}{2} \right)}^3} \times \left(\frac{{{2}^3}}{{{3}^2}} \right)=\frac{{{3}^6}}{{{2}^3}} \times \frac{{{2}^3}}{{{3}^2}} $
We can rewrite the expression to apply the quotient law:
$latex \frac{{{3}^6}}{{{2}^3}} \times \frac{{{2}^3}}{{{3}^2}}=\frac{{{3}^6}}{{{3}^2}} \times \frac{{{2}^3}}{{{2}^3}}$
$latex ={{3}^{6-2}}\times{{2}^{3-3}}$
Simplifying and applying the law of zero exponents, we have:
$latex {{3}^{6-2}}\times{{2}^{3-3}}={{3}^4}\times {{2}^0}$
$latex =81\times 1$
$latex =81$
EXAMPLE 6
Simplify the algebraic expression $$\frac{{{a}^{-3}}{{b}^{2}}}{{{b}^2}{{a}^2}}$$
Solution
In this problem, we have the variables a and b, but we apply the laws of exponents in the same way. Therefore, we start with the law of negative exponents:
$latex \frac{{{a}^{-3}}{{b}^2}}{{{b}^2}{{a}^2}}=\frac{{{b}^2}}{{{b}^2}{{a}^2}{{a}^3}}$
Now, we apply the quotient law to the variable a and the product law to the variable b:
$latex \frac{{{b}^2}}{{{b}^2}{{a}^2}{{a}^3}}=\frac{{{b}^{2-2}}}{{{a}^{2+3}}}$
$latex =\frac{1}{{{a}^5}}$
EXAMPLE 7
Simplify the expression $latex {{(10{{x}^4})}^{-2}}{{y}^{-1}}$.
Solution
We start by applying the law of negative exponents. Therefore, we take the reciprocal of the bases and change the exponents to positive:
$latex {{(10{{x}^4})}^{-2}}{{y}^{-1}}=\frac{1}{{{(10{{x}^4})}^2}{{y}^1}}$
Now, we apply the law of the power of a power:
$latex \frac{1}{{{(10{{x}^4})}^2}{{y}^1}}=\frac{1}{{{10}^2}{{x}^8}{{y}^1}}$
$latex =\frac{1}{100{{x}^8}y}$
EXAMPLE 8
Simplify the expression $latex {{({{x}^{-3}}z)}^2}\times {{({{x}^{2}}{{z}^3})}^{-3}}$.
Solution
We start by applying the law of negative exponents to the right side:
$latex {{({{x}^{-3}}z)}^2}\times {{({{x}^{2}}{{z}^3})}^{-3}}=\frac{{{({{x}^{-3}}z)}^2}}{{{({{x}^{2}}{{z}^3})}^3}}$
We eliminate the parentheses when applying the law of the power of a power:
$latex \frac{{{({{x}^{-3}}z)}^2}}{{{({{x}^{2}}{{z}^3})}^3}}=\frac{{{x}^{-6}}{{z}^2}}{{{x}^6}{{z}^9}}$
We apply the law of negative exponents again:
$latex \frac{{{x}^{-6}}{{z}^2}}{{{x}^6}{{z}^9}}=\frac{{{z}^2}}{{{x}^6}{{x}^6}{{z}^9}}$
Now, we apply the quotient law to z and the product law to x:
$latex \frac{{{z}^2}}{{{x}^6}{{x}^6}{{z}^9}}=\frac{1}{{{x}^{6+6}}{{z}^{9-2}}}$
$latex =\frac{1}{{{x}^{12}}{{z}^7}}$
Laws of exponents – Practice problems
Test your knowledge of the laws of exponents with the following problems. Simplify or solve the expressions and select the correct answer. See the solved examples above if you need help.
See also
Interested in learning more about exponents? Take a look at these pages: