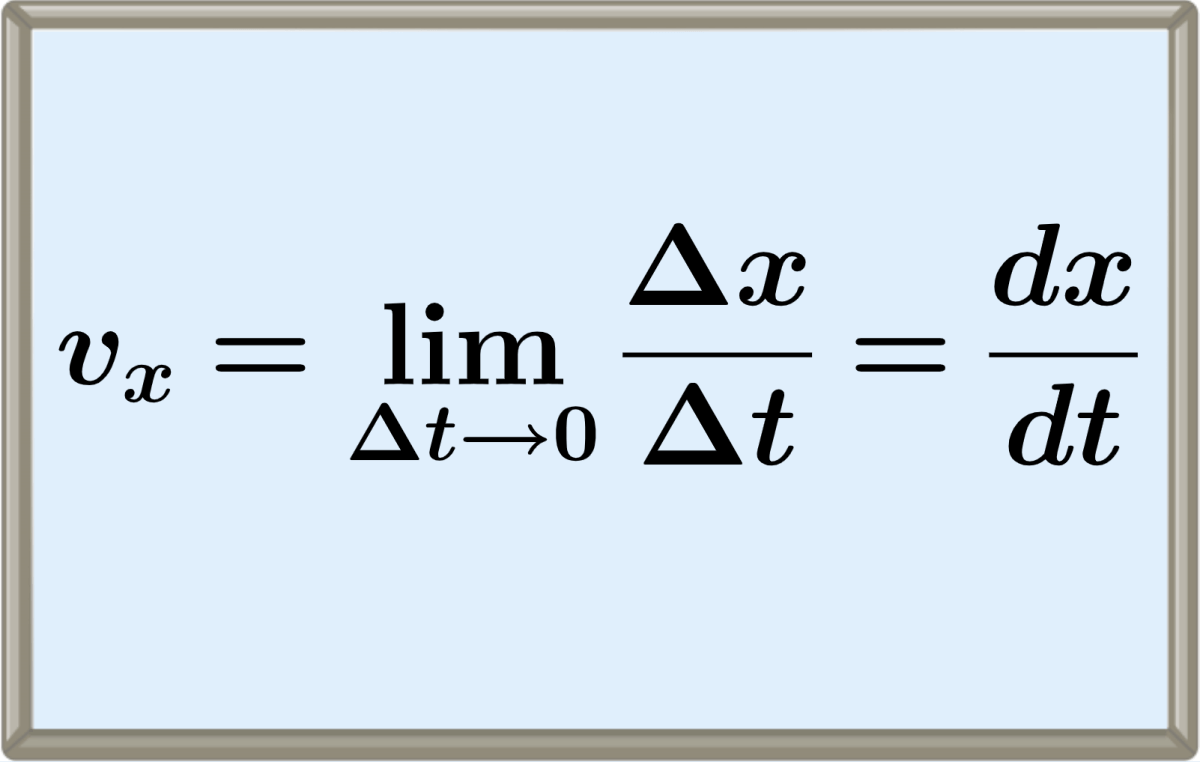Instantaneous velocity in physics describes an object’s speed and direction at a specific moment in time. It can be calculated using calculus to find the derivative of the object’s position with respect to time.
In this article, we’ll delve into the mathematical formula and calculations of instantaneous velocity. We’ll also look at some examples to learn how to calculate it.
What is Instantaneous Velocity in Physics?
Instantaneous velocity is the velocity of an object at a specific instant or point in time. It provides a more detailed and precise measure of an object’s motion compared to average velocity.
Instantaneous velocity is different from average velocity, which is the total displacement of an object over a certain period of time, divided by the time interval. On the other hand, instantaneous velocity concerns the rate of change of an object’s position at a specific time – think of it as the speed and direction of an object at an exact instant.
A helpful way to visualize instantaneous velocity is to imagine you’re driving a car. Your speedometer gives you your instantaneous velocity. As you accelerate, decelerate, or change direction, the speedometer shows your speed and direction at that precise moment. In contrast, your average velocity would be the total distance you’ve covered divided by the time taken, which doesn’t necessarily reflect your speed at any given moment during your journey.
One crucial feature of instantaneous velocity is that it takes into account the direction of motion, making it a vector quantity. This is important because it means the velocity can be positive or negative, depending on the direction of the object’s movement.
Understanding instantaneous velocity is crucial because it plays a vital role in various aspects of physics, including kinematics and dynamics. It’s also central to calculus, where it’s formally defined as the derivative of the position with respect to time. This understanding allows physicists to predict an object’s future positions, calculate acceleration, and more.
Instantaneous Velocity Formula and Calculation
Mathematically, instantaneous velocity is the rate of change of displacement with respect to time at a particular moment. In calculus, it’s the derivative of the position function with respect to time, or equivalently, it can be defined as the limit of average velocity as the time interval approaches zero.
This can be represented as:
$$v(t)= \lim\limits_{\Delta t\to 0} \frac{ \Delta s}{ \Delta t}$$
$$v(t) = \frac{ds}{dt}$$
Where:
- $latex v(t)$ is the instantaneous velocity
- $latex \frac{ds}{dt}$ is the derivative of the displacement ($latex s$) with respect to time ($latex t$)
- $latex \Delta t$ is the change in time
- $latex \lim\limits_{\Delta t\to 0}$ denotes the limit as $latex \Delta t$ approaches zero
This formula stems from the concept of a limit in calculus. The average velocity over a time interval $latex \Delta t$ is given by $latex \frac{\Delta s}{\Delta t}$, where $latex \Delta s$ is the change in position.
As we make this time interval infinitesimally small (approaching zero), the average velocity becomes the instantaneous velocity. That’s why instantaneous velocity is defined as the derivative of position with respect to time.
Calculating instantaneous velocity step-by-step
Now, let’s discuss the steps to calculate instantaneous velocity:
- Identify the Position Function: The position function of the moving object could be a simple linear function, a quadratic function, or any other function representing the object’s position at different times.
- Derive the Position Function: This will provide us with the velocity function.
- Substitute the Specific Time: The result will be the instantaneous velocity at that particular moment in time.
It’s important to note that if the velocity is negative at a certain time, it indicates that the object was moving in the opposite direction at that time. Conversely, a positive velocity signifies motion in the positive direction.
Instantaneous Velocity – Examples with Answers
To solidify the understanding of instantaneous velocity, let’s look at a few examples with detailed solutions.
EXAMPLE 1
Suppose an object is moving along a line according to the position function $latex s(t) = 2t^2 + 3t + 1$, where $latex s$ is in meters and $latex t$ is in seconds. What is the object’s instantaneous velocity at $latex t = 2$ seconds?
Solution
First, we need to derive the position function with respect to time to get the velocity function. The derivative of $latex s(t)$ is:
$$v(t) = \frac{ds}{dt}$$
$$v(t) = 4t + 3$$
Next, we substitute $latex t = 2$ into the velocity function:
$latex v(2) = 4\times 2 + 3$
$latex v(2) = 11 ~\frac{\text{m}}{\text{s}}$
Therefore, the object’s instantaneous velocity at $latex t = 2$ seconds is 11 m/s.
EXAMPLE 2
Consider a car moving along a track described by the position function $latex s(t) = 5t^3 – 7t + 9$, where $latex s$ is in kilometers and $latex t$ is in hours. Find the car’s instantaneous velocity at $latex t = 1$ hour.
Solution
Deriving the position function, we get the velocity function:
$$v(t) = \frac{ds}{dt}$$
$latex v(t) = 15t^2 – 7$
Substituting $latex t = 1$ into the velocity function:
$latex v(1) = 15\times 1 – 7$
$latex v(1) = 8 ~\frac{\text{km}}{\text{h}}$
Then, the car’s instantaneous velocity at $latex t = 1$ hour is 8 km/h.
EXAMPLE 3
A particle is moving along a path defined by the position function $latex s(t) = 3t^2 – 2t + 5$, where $latex s$ is in meters and $latex t$ is in seconds. Calculate the particle’s instantaneous velocity at $latex t = 3$ seconds.
Solution
We start by finding the derivative of $latex s(t)$:
$$v(t) = \frac{ds}{dt}$$
$latex v(t) = 6t – 2$
Substituting $latex t = 3$ into the velocity function:
$latex v(3) = 6\times 3 – 2$
$latex v(3) = 16 ~\frac{\text{m}}{\text{s}}$
Thus, the particle’s instantaneous velocity at $latex t = 3$ seconds is 16 m/s.
EXAMPLE 4
A train’s position is described by the function $latex s(t) = 4t^3 – 3t^2 + 2t – 1$, where $latex s$ is in kilometers and $latex t$ is in minutes. What is the train’s instantaneous velocity at $latex t = 2$ minutes?
Solution
The derivative of the position function is:
$$v(t) = \frac{ds}{dt}$$
$latex v(t) = 12t^2 – 6t + 2$
Substituting $latex t = 2$ into the velocity function:
$latex v(2) = 12\times 4 – 12 + 2 $
$latex v(2)= 34 ~\frac{\text{km}}{\text{min}}$
Therefore, the train’s instantaneous velocity at $latex t = 2$ minutes is 34 km/min.
Applications of Instantaneous Velocity
Instantaneous velocity may seem like a concept primarily reserved for physics textbooks, but it has numerous applications in our daily lives. Let’s explore some of the most common ones.
Space Exploration
In space exploration, knowing the instantaneous velocity of a spacecraft is crucial. Calculations of instantaneous velocity allow engineers to make real-time decisions about trajectory and speed adjustments. This is essential in executing complex maneuvers.
Vehicle Motion Analysis
In the automotive industry, the concept of instantaneous velocity is fundamental in the design and testing phases. For example, crash tests need to know the exact velocity at the moment of impact to analyze the effectiveness of safety measures.
Another more common example is the use of speedometers as they indicate the instantaneous velocity of a car at a specific time.
Sports and Athletics
In sports, especially those involving balls or other fast-moving objects, knowing the instantaneous velocity can be crucial. For example, in baseball, the speed of a pitch or the velocity of a hit ball at any particular moment can significantly affect the game’s outcome.
Advanced tracking systems provide such data, enhancing both the strategy and the spectator’s experience.
Instantaneous Velocity in Theoretical Physics
Beyond its practical applications, instantaneous velocity is also a foundational concept in theoretical physics. It plays a crucial role in the study of motion and dynamics, kinematics, and quantum mechanics.
See also
Interested in learning more about motion? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
