The pentagonal prism is a three-dimensional figure formed by two pentagonal bases. The pentagonal bases are congruent and parallel to each other. These bases are connected by five rectangular side faces. These prisms are characterized by having a total of 7 faces, 10 vertices, and 15 edges. Pentagonal prisms are also considered as heptahedra.
Here, we will learn some of the most important characteristics of this type of prism. In addition, we will learn about its most used formulas and we will apply them to solve some problems.
Definition of a pentagonal prism
A pentagonal prism is a prism that has two pentagonal bases and five lateral rectangular faces. These prisms are a type of heptahedron with 7 faces, 10 vertices, and 15 edges.
Each vertex of the pentagonal prism is a point where three faces, two rectangular faces, and one pentagonal face meet. The following is a diagram of a pentagonal prism.
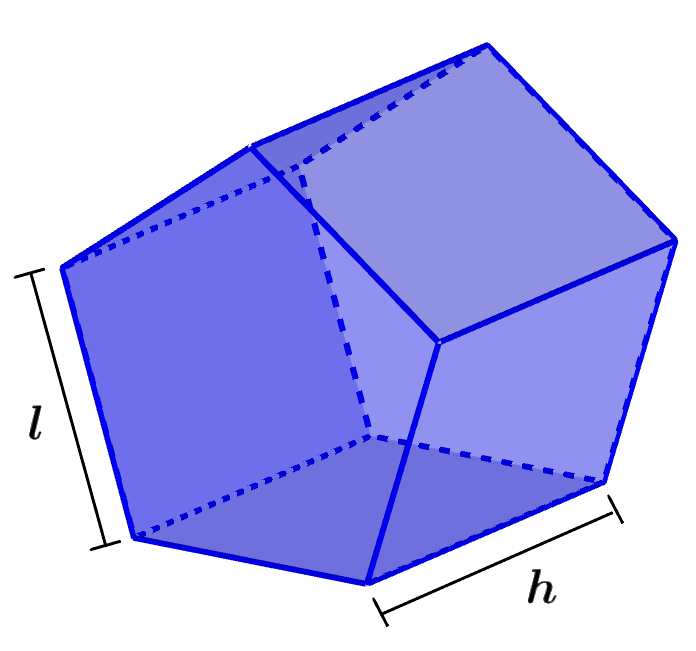
There are two main types of pentagonal prisms:
- Regular Pentagonal Prisms
- Right Pentagonal Prisms
Regular Pentagonal Prisms
Regular pentagonal prisms are formed when all sides of the pentagonal base are the same length. In these prisms, all the rectangular side faces are congruent, that is, they have the same dimensions. The lateral faces are often called the sides of the prism.
Right Pentagonal Prisms
A pentagonal prism is right when it has two pentagonal bases that are congruent and parallel to each other and when the lateral faces form a right angle (90 degrees) with the bases.
Fundamental characteristics of a pentagonal prism
The following are the most important characteristics of a pentagonal prism:
- The bases of the prism are pentagons.
- The bases are congruent and parallel with each other
- The lateral faces of the prism are rectangles.
- If the prism is regular, the lateral faces are congruent.
- These prisms have 7 faces, 10 vertices, and 15 edges.
- Two rectangular faces and a pentagonal face meet at each vertex.
- A pentagonal prism is right when the bases form 90 degree angles with the lateral faces.
Important pentagonal prism formulas
Pentagonal prisms are 3D figures, so their most important formulas are the volume formula and the surface area formula.
Formula for the volume of a pentagonal prism
The volume of a prism is equal to the area of the base times the height of the prism. In this case, we have the formula:
| $latex V=\frac{5}{2}alh$ |
where a represents the length of the apothem of the hexagon, l is the length of one of the sides of the hexagon and h is the height of the prism.
Formula for the surface area of a pentagonal prism
The surface area of a prism is equal to the sum of the areas of all its faces. We have two pentagonal faces and five rectangular faces, so its formula for the surface area is:
| $latex A_{s}=5al+5lh$ |
Examples of pentagonal prism problems
The following exercises are solved by applying the pentagonal prism formulas seen above.
EXAMPLE 1
If a pentagonal prism has an apothem of 5.5 m, a height of 6 m, and sides of length 8 m, what is its volume?
Solution: We have the lengths $latex a=5.5$, $latex l=6$ and $latex h=8$. Using these values in the volume formula, we have:
$latex V=\frac{5}{2}alh$
$latex V=\frac{5}{2}(5.5)(8)(6)$
$latex V=251.3$
The volume of the prism is 660 m³.
EXAMPLE 2
What is the volume of a prism that has a height of 10 m and a pentagonal base with sides of a length of 9 m and an apothem of 6.2 m?
Solution: We have the values $latex a=6.2$, $latex l=9$ and $latex h=10$. We use the volume formula with these values:
$latex V=\frac{5}{2}alh$
$latex V=\frac{5}{2}(6.2)(9)(10)$
$latex V=1395$
The volume of the prism is 1395 m³.
EXAMPLE 3
What is the surface area of a prism that has a height of 10 m, an apothem of 5.5 m, and sides of length 6 m?
Solution: We have the lengths $latex h=10$, $latex a=5.5$, and $latex l=6$, so we use these values in the formula for surface area:
$latex A_{s}=5al+5lh$
$latex A_{s}=5(5.5)(6)+5(6)(10)$
$latex A_{s}=165+300$
$latex A_{s}=465$
The surface area is 465 m².
EXAMPLE 4
What is the surface area of a prism that has a height of 6 m, an apothem of 4.8 m, and sides of length 8 m?
Solution: We can use the values given in the formula for surface area:
$latex A_{s}=5al+5lh$
$latex A_{s}=5(4.8)(8)+5(8)(6)$
$latex A_{s}=192+240$
$latex A_{s}=854.5$
The surface area is 432 m².
See also
Interested in learning more about pentagonal prisms? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
