The volume of a pentagonal prism can be calculated by multiplying the area of the pentagonal base by the height of the prism. On the other hand, the surface area of a pentagonal prism is calculated by adding the areas of all the faces of the prism.
Here, we will look at the formulas that we can use to calculate the volume and the surface area of pentagonal prisms. Also, we will use these formulas to solve some practice problems.
GEOMETRY
Relevant for…
Learning to calculate the volume and area of a pentagonal prism.
GEOMETRY
Relevant for…
Learning to calculate the volume and area of a pentagonal prism.
How to find the volume of a pentagonal prism
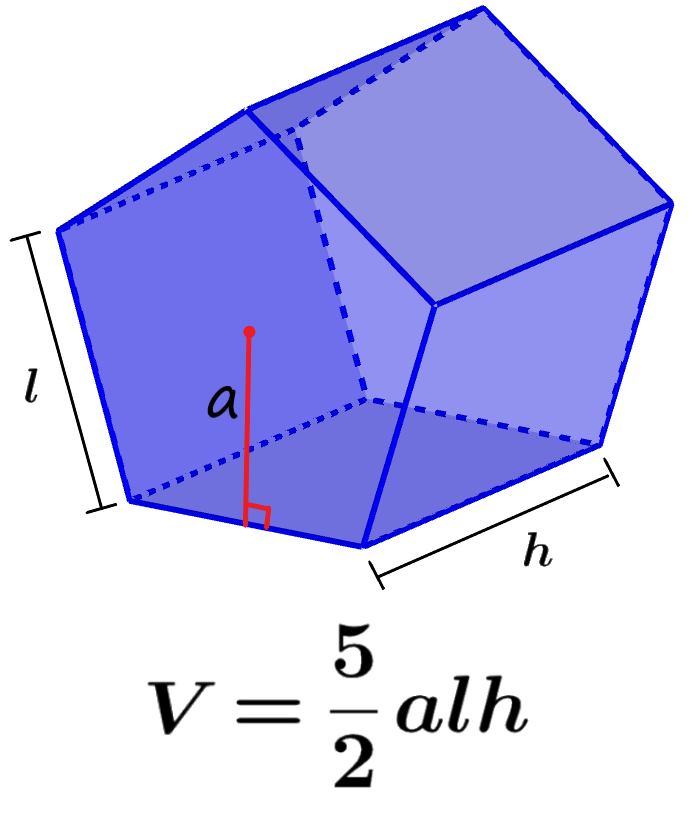
We can find the volume of a pentagonal prism by multiplying the area of the base by the height of the prism. Recall that we can use the apothem to calculate the area of polygons easily. Therefore, we have the following formula:
$latex V = \frac{5}{2} alh$
where a represents the length of the apothem, l represents the length of the sides of the pentagonal base and h represents the length of the height of the prism.
Furthermore, we can also find the volume of a prism using only the length of its height and the length of one of the sides of its hexagonal base. For this, we use the following formula:
$latex V=\frac{1}{4}\sqrt{5(5+2\sqrt{5}})~{{l}^2}h$
This formula is more complicated, but we can approximate it to the following expression:
$latex V=1.72{{l}^2}h$
How to find the surface area of a pentagonal prism
The surface area of any three-dimensional figure is found by adding the areas of all its faces. In the case of pentagonal prisms, we have two pentagonal faces and five rectangular faces.
We can find the area of each pentagonal face using the formula $latex 3.44{{l}^2}$, where, l represents the length of one of the sides of the pentagonal face. Therefore, the area of both pentagonal faces is $latex 6.88{{l}^2}$.
On the other hand, the area of each rectangular face is found using the formula lh, where, l is the length of one of the sides of the pentagonal face and h is the length of the height of the prism. Therefore, the area of the five rectangular areas is 5hl.
By adding these two parts, we have the formula for the surface area of a pentagonal prism:
$latex A_{s} = 6.88 {{l}^2}+5hl$
Volume and area of a pentagonal prism – Examples with answers
EXAMPLE 1
What is the volume of a prism that has a height of 6 m and a base with sides of length 8 m and an apothem of 5.5 m?
Solution
We have the following information:
- Prism height, $latex h=6$
- Pentagon sides, $latex l=8$
- Apothem, $latex a=5.5$
Using the first volume formula with this information, we have:
$latex V=\frac{5}{2}alh$
$latex V=\frac{5}{2}(5.5)(8)(6)$
$latex V=660$
The volume of the prism is 660 m³.
EXAMPLE 2
What is the surface area of a pentagonal prism with a height of 4 m and sides of length 4 m?
Solution
We have $latex h=4$ and $latex l=4$. Therefore, we use the formula for surface area with these values:
$latex A_{s}=6.88{{l}^2}+5lh$
$latex A_{s}=6.88{{(4)}^2}+5(4)(4)$
$latex A_{s}=110.1+80$
$latex A_{s}=190.1$
The surface area is equal to 190.1 m².
EXAMPLE 3
A prism has a height of 8 m and its base is a pentagon with sides of length 9 m and an apothem of 6.2 m. What is its volume?
Solution
We recognize the following values:
- Prism height, $latex h=8$
- Pentagon sides, $latex l=9$
- Apothem, $latex a=6.2$
We substitute these values in the first formula of the volume:
$latex V=\frac{5}{2}alh$
$latex V=\frac{5}{2}(6.2)(9)(8)$
$latex V=1116$
The volume of the prism is 1116 m³.
EXAMPLE 4
If a prism has a height of 9 m and a pentagonal base with sides of 5 m, what is its surface area?
Solution
We use the lengths $latex h=9$ and $latex l=5$ in the formula for surface area:
$latex A_{s}=6.88{{l}^2}+5lh$
$latex A_{s}=6.88{{(5)}^2}+5(5)(9)$
$latex A_{s}=172+225$
$latex A_{s}=395$
The surface area is equal to 395 m².
EXAMPLE 5
If a prism has a height of 10 m and a pentagonal base with sides of length 5 m, what is its volume?
Solution
We have the following values:
- Prism height, $latex h=10$
- Pentagon sides, $latex l=5$
We have to use the second volume formula with these values:
$latex V=1.72{{l}^2}h$
$latex V=1.72{{(5)}^2}(10)$
$latex V=430$
The volume of the prism is 430 m³.
EXAMPLE 6
What is the surface area of a pentagonal prism that has sides of 6 m and a height of 11 m?
Solution
We have the lengths $latex h=11$ and $latex l=6$, so we use these values in the formula for surface area:
$latex A_{s}=6.88{{l}^2}+5lh$
$latex A_{s}=6.88{{(6)}^2}+5(6)(11)$
$latex A_{s}=247.7+330$
$latex A_{s}=577.7$
The surface area is equal to 577.7 m².
EXAMPLE 7
A prism has a pentagonal base with sides of length 3 m. If its height is 5 m, what is its volume?
Solution
We have the following:
- Prism height, $latex h=5$
- Pentagon sides, $latex l=3$
Using the second formula with these values, we have:
$latex V=1.72{{l}^2}h$
$latex V=1.72{{(3)}^2}(5)$
$latex V=77.4$
The volume of the prism is 77.4 m³.
EXAMPLE 8
If a pentagonal prism has sides that are 8 m long and 12 m high, what is its surface area?
Solution
We replace the lengths $latex h=12$ and $latex l=8$ in the formula for the surface area:
$latex A_{s}=6.88{{l}^2}+5lh$
$latex A_{s}=6.88{{(8)}^2}+5(8)(12)$
$latex A_{s}=440.3+480$
$latex A_{s}=920.3$
The surface area is equal to 920.3 m².
EXAMPLE 9
What is the volume of a prism that has a height of 12 m and a pentagonal base with sides of 6 m?
Solution
We have the following values:
- Prism height, $latex h=12$
- Pentagon sides, $latex l=6$
If we use the second formula, we have:
$latex V=1.72{{l}^2}h$
$latex V=1.72{{(6)}^2}(12)$
$latex V=743.04$
The volume of the prism is 743.04 m³.
Volume and area of a pentagonal prism – Practice problems
If we have a pentagonal prism with a height of 7 m and sides of length 3 m, what is its surface area?
Write the answer to one decimal place.
See also
Interested in learning more about pentagonal prisms? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
