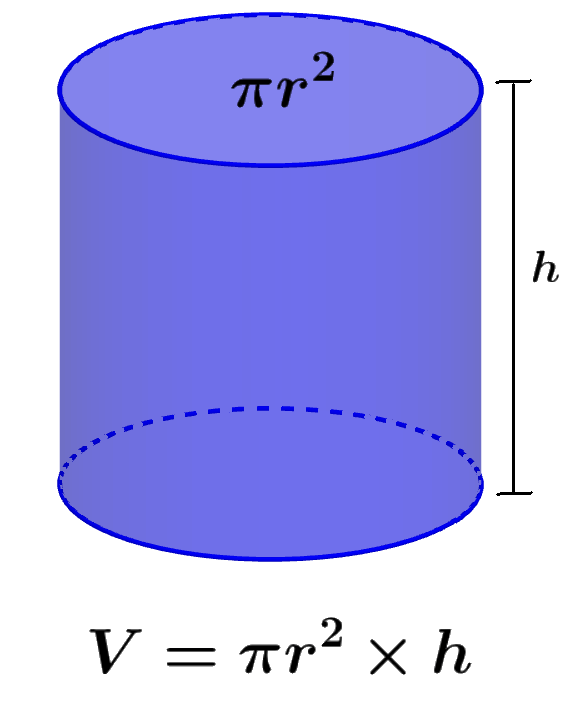
The volume of a cylinder is the density of the cylinder, which means the amount of material that can fit in the cylinder or the amount of space occupied by the cylinder. Remember that the cylinder is a three-dimensional figure that has circular bases. A cylinder can be seen as a pair of circular discs that are placed one on top of the other. Therefore, we calculate its volume by multiplying the area of the circular bases by the height of the cylinder.
Here, we will learn about the formula that we can use to calculate the volume of a cylinder both in terms of the radius and the height and in terms of the diameter. Also, we will learn how to calculate the volume of hollow cylinders. Finally, we will use the learned formulas to solve some exercises.
Formula to find the volume of a cylinder
A cylinder can be seen as a set of congruent discs located one on top of the other. To calculate the space occupied by the cylinder, we calculate the space occupied by each disk and then add them together. Therefore, the volume of a cylinder is given by the product of the area of the base and the height:
Volume = Base × Height
We know that a cylinder has circular bases, so the area of the base is equal to πr², where r is the radius. Therefore, the formula for the volume of a cylinder is:
| $latex V=\pi {{r}^2}\times h$ |
where r is the length of the cylinder’s radius and h is the length of its height.
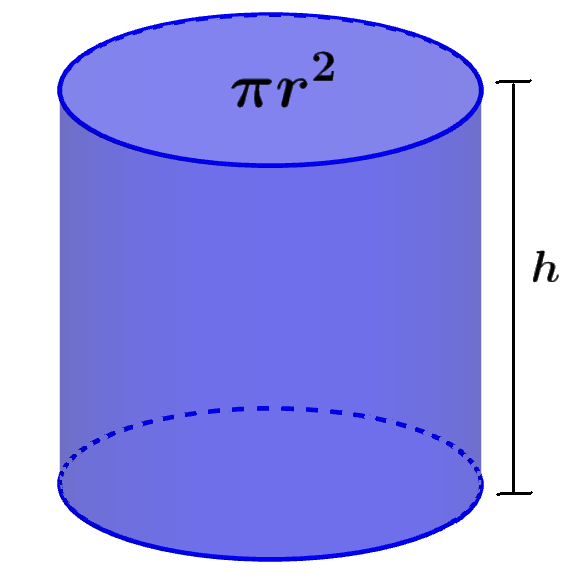
Formula to find the volume of a cylinder using the diagonal
It is also possible to calculate the volume of a cylinder using the length of its diameter. We can use two methods to achieve this.
The first method is to remember that the diameter of a cylinder is equal to 2r, where r is the length of the radius of the cylinder. Therefore, if we know the diameter of the cylinder, we can simply divide the diameter by 2 to get the length of the radius and apply the volume formula above.
Alternatively, it is also possible to write the equivalence of the radius and the diameter and obtain a formula for the volume in terms of the diameter of the cylinder:
$latex V=\pi {{r}^2}\times h$
$latex V=\pi {{(\frac{d}{2})}^2}\times h$
$latex V=\pi \frac{{{d}^2}}{4}\times h$
| $latex V= \frac{\pi{{d}^2}}{4}\times h$ |
where d is the length of the diameter.
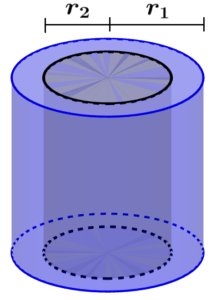
Formula to find the volume of a hollow cylinder
In the case of hollow cylinders, we measure two radii, one for the inner circle and one for the outer circle formed by the base of the hollow cylinder. The volume of this type of cylinder can be calculated with the following formula:
| $latex V=\pi h({{r_{1}}^2}-{{r_{2}}^2})$ |
where, $latex r_{1}$ is the radius of the outer circle, $latex r_{2}$ is the radius of the inner circle, and h is the height of the cylinder.
This formula is equivalent to calculating the volume of a whole cylinder and then extracting the volume of the inner cylinder that was removed.
Volume of a cylinder – Examples with answers
The formulas for the volume of cylinders detailed above are applied to solve the following examples. Each example has its respective solution, where the process and reasoning used are detailed.
EXAMPLE 1
What is the volume of a cylinder that has a radius of 5 m and a height of 10 m?
Solution
We have the following values:
- Radius, $latex r=5$
- Height, $latex h=10$
Using the volume formula with these values, we have:
$latex V=\pi {{r}^2}\times h$
$latex V=\pi {{(5)}^2}\times 10$
$latex V=\pi (25)\times 10$
$latex V=785.4$
The volume is equal to 785.4 m³.
EXAMPLE 2
If a cylinder has a radius of 6 m and a height of 8 m, what is its volume?
Solution
We have the following information:
- Radius, $latex r=6$
- Height, $latex h=8$
By substituting these values into the volume formula, we have:
$latex V=\pi {{r}^2}\times h$
$latex V=\pi {{(6)}^2}\times 8$
$latex V=\pi (36)\times 8$
$latex V=904.8$
The volume is equal to 904.8 m³.
EXAMPLE 3
What is the volume of a cube that has a diameter of 8 m and a height of 12 m?
Solution
We have the following values:
- Diameter, $latex d=8$
- Height, $latex h=12$
In this case, we use the second volume formula since we have the diameter of the cylinder:
$latex V=\pi (\frac{{{d}^2}}{4})\times h$
$latex V=\pi (\frac{{{(8)}^2}}{4})\times 12$
$latex V=\pi (\frac{64}{4})\times 12$
$latex V=\pi (16)\times 12$
$latex V=603.2$
The volume is equal to 603.2 m³.
EXAMPLE 4
What is the volume of a cylinder that has a diameter of 12 m and a height of 11 m?
Solution
We have the following information:
- Diameter, $latex d=12$
- Height, $latex h=11$
We use those values in the second volume formula:
$latex V=\pi (\frac{{{d}^2}}{4})\times h$
$latex V=\pi (\frac{{{(12)}^2}}{4})\times 11$
$latex V=\pi (\frac{144}{4})\times 11$
$latex V=\pi (36)\times 11$
$latex V=1244.1$
The volume is equal to 1244.1 m³.
EXAMPLE 5
A hollow cylinder has a height of 15 m, an internal radius of 5 m, and an external radius of 6 m. What is its volume?
Solution
We have the following information:
- Height, $latex h=15$
- External radius, $latex r_{1}=6$
- Internal radius, $latex r_{2}=5$
We have a hollow cylinder, so we have to use the third volume formula with the given values:
$latex V=\pi h({{r_{1}}^2}-{{r_{2}}^2})$
$latex V=\pi (15)({{6}^2}-{{5}^2})$
$latex V=\pi (15)(36-25)$
$latex V=\pi (15)(11)$
$latex V=518.4$
The volume is equal to 518.4 m³.
EXAMPLE 6
What is the volume of a hollow cylinder that has a height of 20 m, an internal radius of 6 m, and an external radius of 8 m?
Solution
We have the following values:
- Height, $latex h=20$
- External radius, $latex r_{1}=8$
- Internal radius, $latex r_{2}=6$
We use the third volume formula with these values:
$latex V=\pi h({{r_{1}}^2}-{{r_{2}}^2})$
$latex V=\pi (20)({{8}^2}-{{6}^2})$
$latex V=\pi (20)(64-36)$
$latex V=\pi (20)(28)$
$latex V=1759.3$
The volume is equal to 1759.3 m³.
Volume of a cylinder – Practice problems
Use the formulas for the volume of cylinders to solve the following problems. Select an answer and check it to see if you got the correct answer. If you need help with this, you can look at the solved examples above.
See also
Interested in learning more about cylinders? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
