Thales’ theorem tells us that the diameter of a circle always forms a right triangle when we connect it to any point located on the circumference of the circle. This theorem can be proved by using two isosceles triangles inscribed in a circle and using their angles.
Here, we will look at a more detailed explanation of Thales’ theorem. Also, we will learn how to prove this theorem and use it to solve some practice examples.
Explanation of Thales’ theorem
Thales’ theorem is considered a special case of the inscribed angles theorem. Thales’ theorem is applied to right triangles that are inscribed in a circle.
We are going to use the following diagram to describe Thales’ theorem.
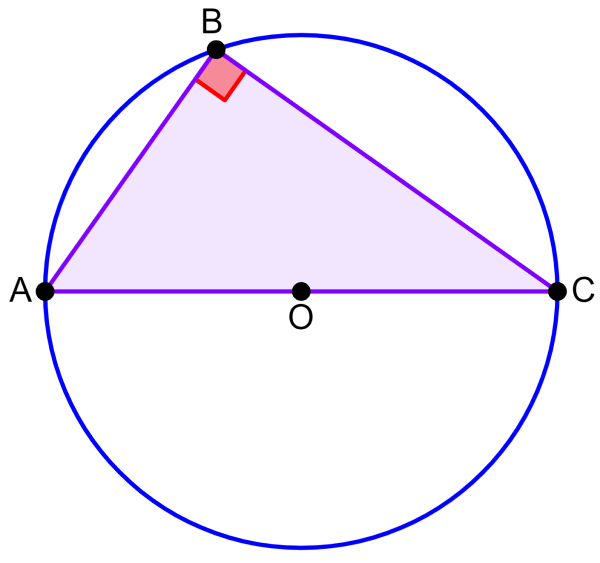
Thales’ theorem indicates that, if points A, B, C are different points located on the circumference of a circle with center O, where line AC is a diameter of the circle, the triangle ΔABC has a right angle (90°) at point B.
Therefore, triangle ΔABC is a right triangle. This means that the diameter of a circle always forms a right angle to any point on the circle.
Proof of Thales’ theorem
We can prove Thales’ theorem in several different ways using algebraic and geometric techniques. However, here we will focus on a geometric method using bisectors and the sum of interior angles.
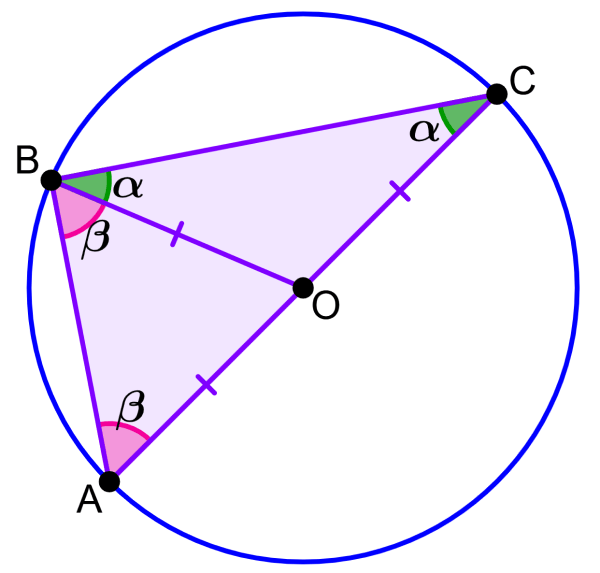
When we connect the center of circle O to point B, we create two triangles ΔABO and ΔOBC. Both triangles are isosceles since the segments OA, OC, OB are equal because they are radii of the circle.
We know that the base angles of an isosceles triangle are equal. Therefore, the angles α of the triangle ΔABO are equal. Similarly, the angles β of the triangle ΔOBC are equal.
We also know that the sum of the interior angles of any triangle is always equal to 180°. Thus, in the triangle ΔABC, we have:
$latex \alpha+(\alpha+\beta)+\beta=180$°
$latex 2\alpha+2\beta=180$°
By dividing the expression by 2, we have:
$latex \alpha+\beta=90$°
The angle α+β is the angle of the triangle ΔABC at point B. Therefore, we have proved the theorem.
Examples with answers of Thales’ Theorem
EXAMPLE 1
Segment AB corresponds to the diameter of the circle. Determine the measure of angle X.
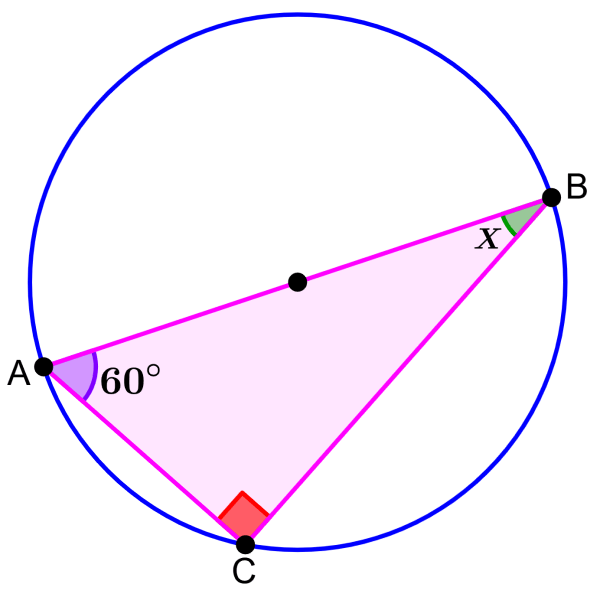
Solution: Since segment AB is the diameter, by Thales’ theorem, we know that the angle formed at vertex C is a 90 ° angle. Also, we know that the sum of interior angles in any triangle is equal to 180°. Therefore, we have:
90°+60°+X=180°
150°+X=180°
X=180°-150°
X=30°
The measure of angle X is 30°.
EXAMPLE 2
Point M is the center of the circle. What is the measure of angle Z?
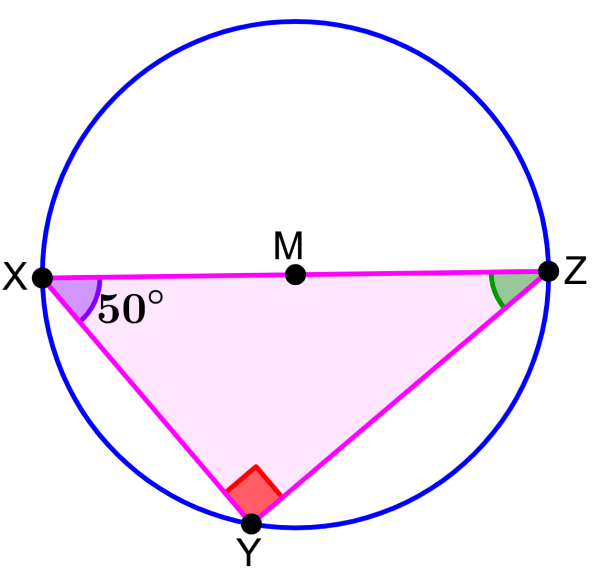
Solution: If point M is the center, it means that segment XZ is the diameter of the circle, so we can apply Thales’ theorem. Therefore, we know that the angle formed at vertex Y is a 90° angle. We can use the sum of interior angles of a triangle to get the measure of Z:
90°+50°+Z=180°
140°+Z=180°
Z=180°-140°
Z=40°
The measure of angle Z is 40 °.
EXAMPLE 3
The XY segment is the diameter of the circle. Determine the length of the diameter.
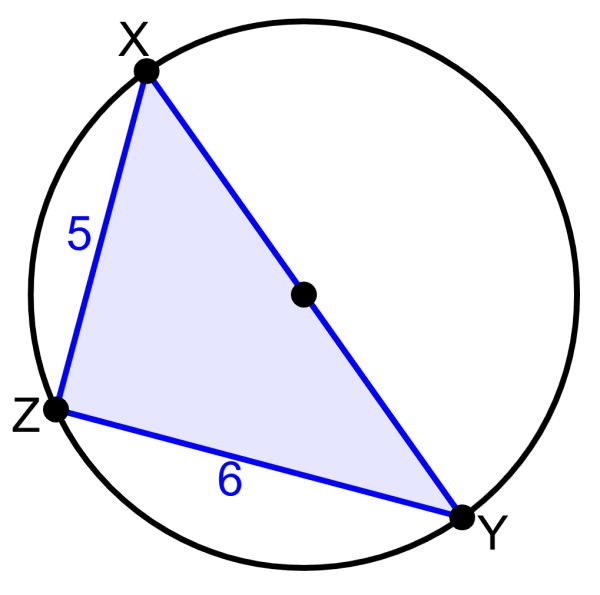
Solution: By Thales’ theorem, we know that triangle XYZ is a right triangle, where angle Z is right. Therefore, we can apply the Pythagorean theorem to determine the length of the XY segment:
$latex {{c}^2}={{a}^2}+{{b}^2}$
$latex {{c}^2}={{5}^2}+{{6}^2}$
$latex {{c}^2}=25+36$
$latex {{c}^2}=61$
$latex c=7.81$
The length of the XY segment is 7.81 units.
EXAMPLE 4
Determine the measure of angle ∠ABC assuming point C is the center of the circle.
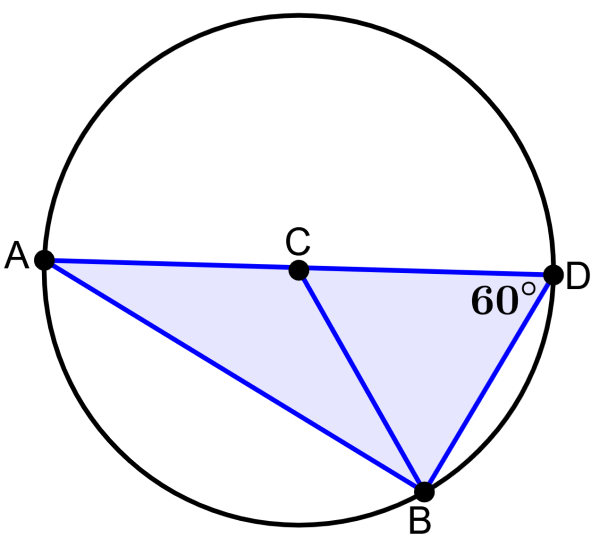
Solution: Point C is the center of the circle, so segment AD is the diameter and we can apply Thales’ theorem.
Earlier, we saw that triangles ABC and BCD must be isosceles triangles. So, we have:
∠CBD = ∠CDB =60°
By Thales’ theorem, we know that:
∠ABD =90°
Therefore, we have:
∠ABC = 90°-60°=30°
Thus, the measure of angle ∠ABC is 30°.
See also
Interested in learning more about Thale’s theorem? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
