Stellations are formed when we extend the sides of a polygon and those sides intersect each other. The number of times the sides intersect outside the polygon determines the degree of the stellation.
Here, we will learn more details about stellations. We will use diagrams and various examples to understand the concepts.
Stellation process
Stellation is the process of building polyhedra by extending the sides of the polygons until they intersect. If the sides never intersect at a point outside the polygon, the polygon cannot be stellated.
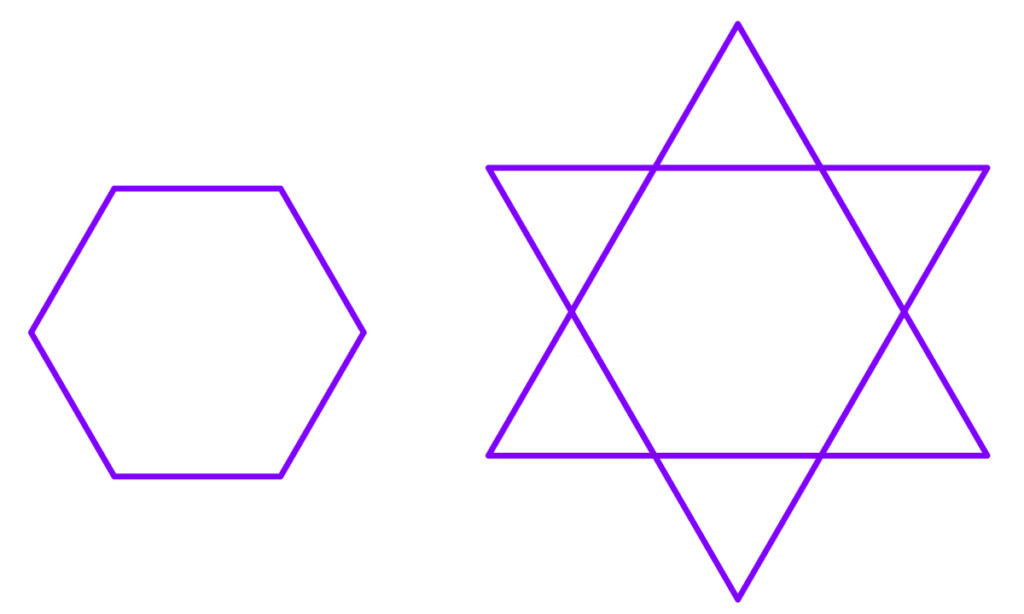
For example, the stellation of a regular hexagon is shown in the following image.
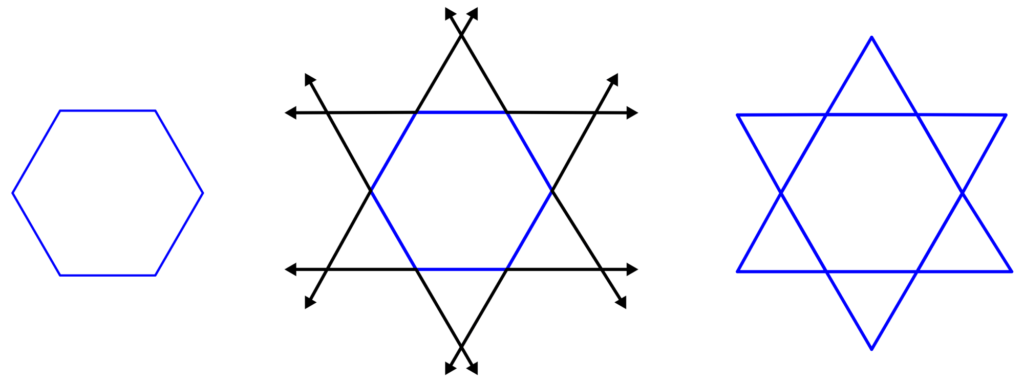
Now, let’s think about which of the following polygons cannot be stellated:
• Square
• Regular pentagon
• Regular octagon
• Regular decagon
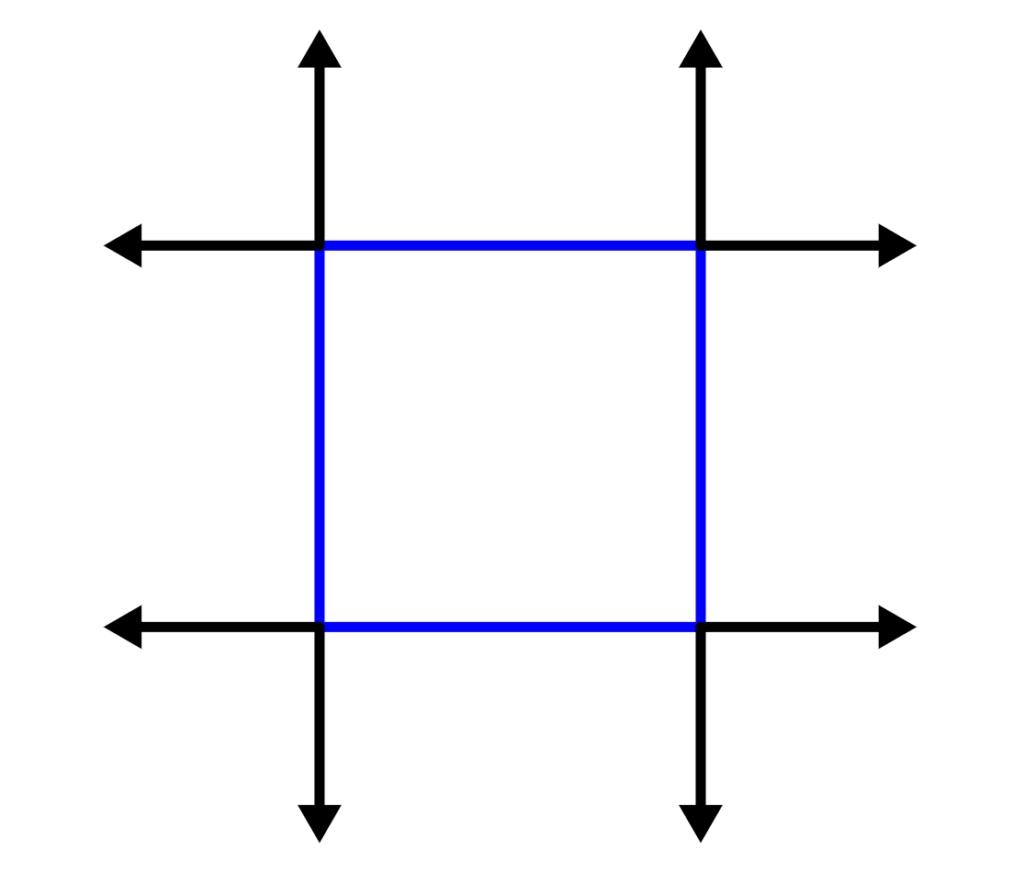
The answer is a square. By extending the sides of a square, we form parallel lines that never intersect at one point.
Stellations of n order
A first-order stellation of a polygon extends the sides until the lines first cross outside the polygon. An n-order stellation extends the sides of a polygon until the lines intersect n times in each direction.
For example, the following diagram shows a second-order stellation since the lines leaving the polygon to intersect twice.
We can determine the measure of angle x using the following diagram:
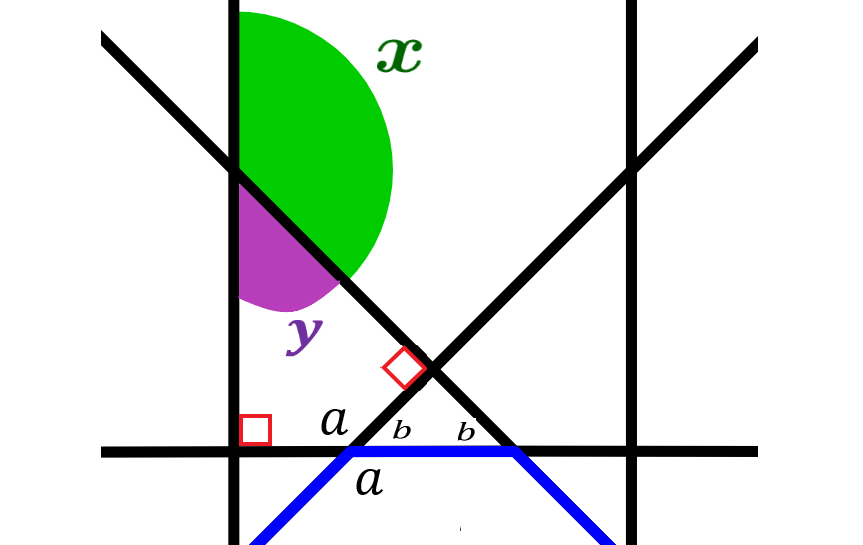
Since the octagon is regular, the interior angles of the octagon measure 135°. Therefore, in the diagram we have a=135°. Since a and b are a linear pair, b=180°-135° = 45°. This implies that each triangle in the stellation is a right isosceles triangle, with right angles.
The angles of a quadrilateral add up to 360°, therefore, y= 360°-90°-90°-135° = 45°.
Finally, since y and x are a linear pair, we have x=180°-45°=135°.
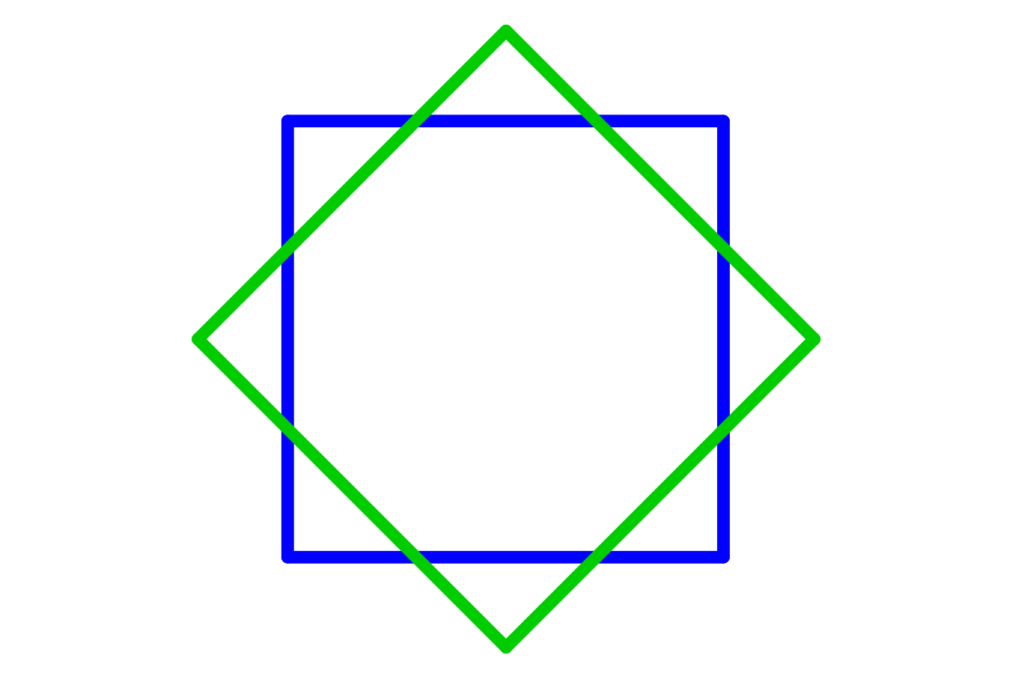
Now, let’s look at other ways to form stellations. If we start with a square and then place a congruent square rotated by 45°, we will generate a stellated octagon of the first order.
If we start with an equilateral triangle and place multiple copies rotated by 30° we will form a dodecagon (12 sides). If we rotate 30°, after 120° of rotation, the triangle will be overlapping the original triangle. That means there are (120°)/(30°)=4 equilateral triangles, which form a polygon with 3×4=12 sides.
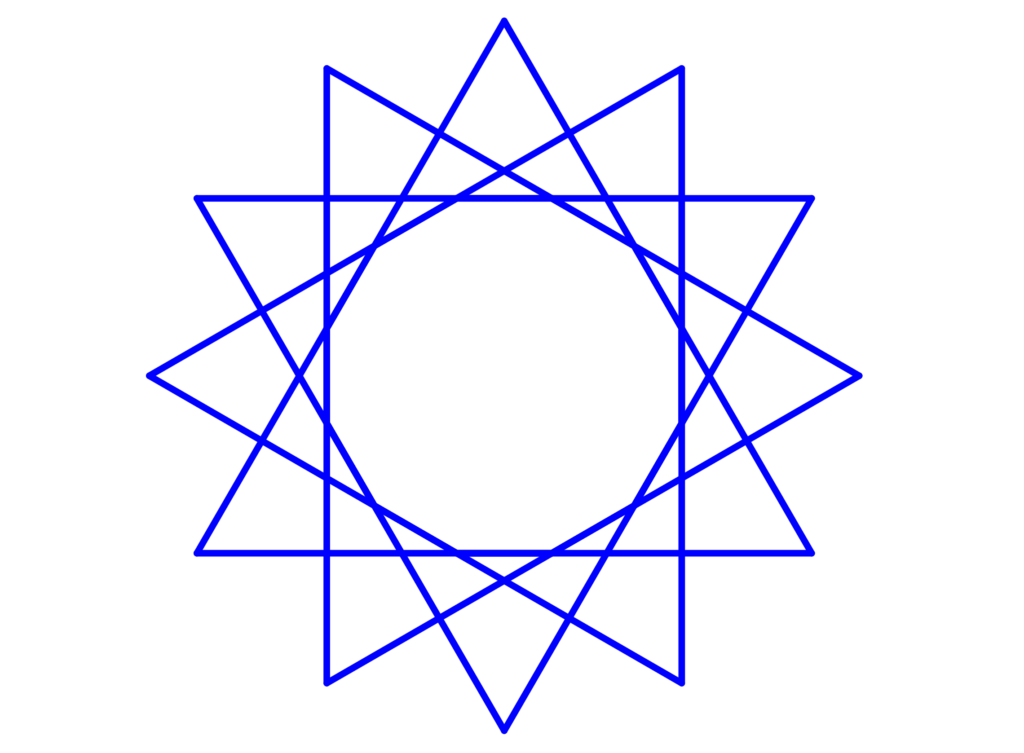
The figure is a third-order stellated polygon since the points of the triangles are at the third intersection.
Solved problems of stellations of polygons
EXAMPLE 1
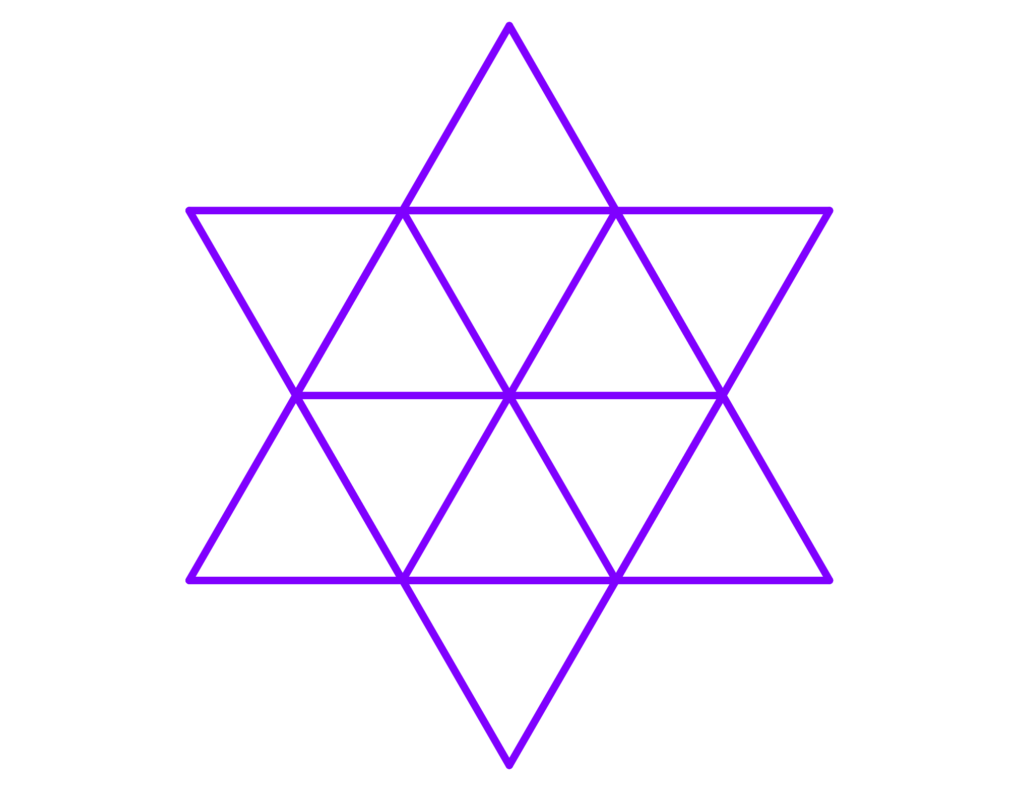
The following image is a regular polygon with area A, and that same hexagon is stellated to form a star. What is the area of the star?
Solution: A regular hexagon can be divided into equilateral triangles. All angles will be 60°.
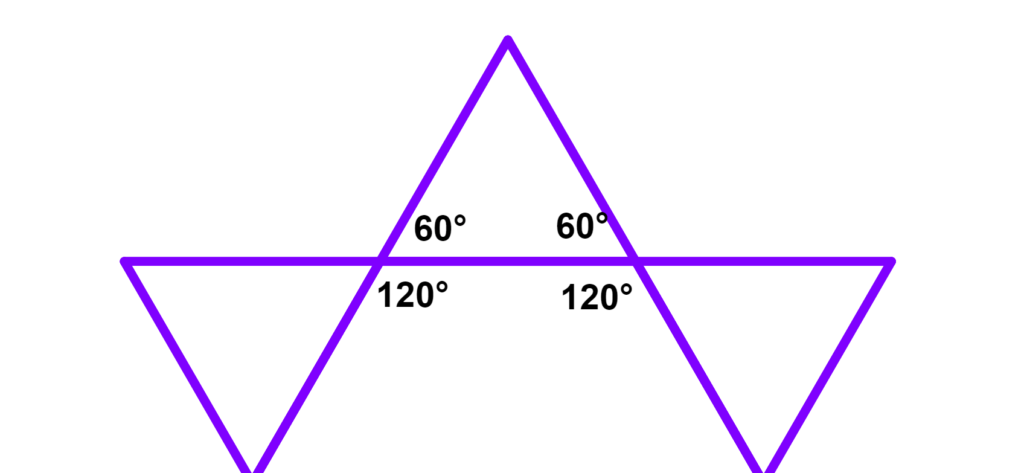
The stellations are also equilateral triangles. Since each angle of the original regular hexagon is $latex \frac{((6-2) 180^{\circ})}{6}=120$°, the angles of the exterior triangles must be 180°-120°=60°.
Since the triangles are equal, the area of the original pentagon and the sum of the areas of the outer triangles are the same. Therefore, the total area is 2A.
EXAMPLE 2
What fraction of the total area of the stellated pentagon does the green region represent?
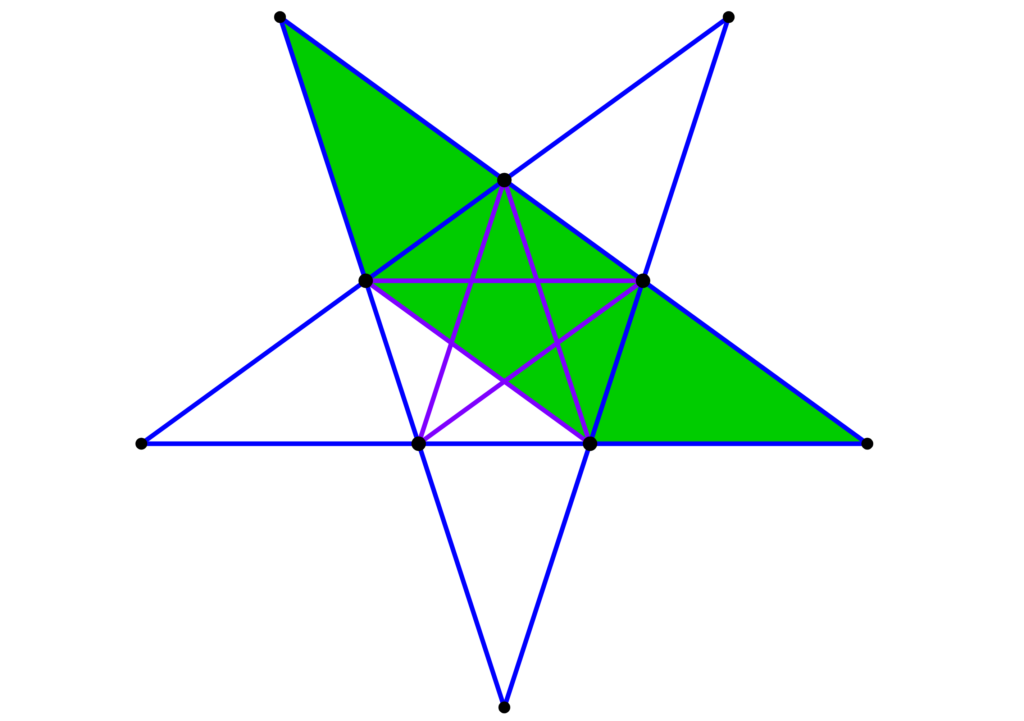
Solution: Each part of the figure in the green region has a congruent part in the white region. These parts are marked in the figure.
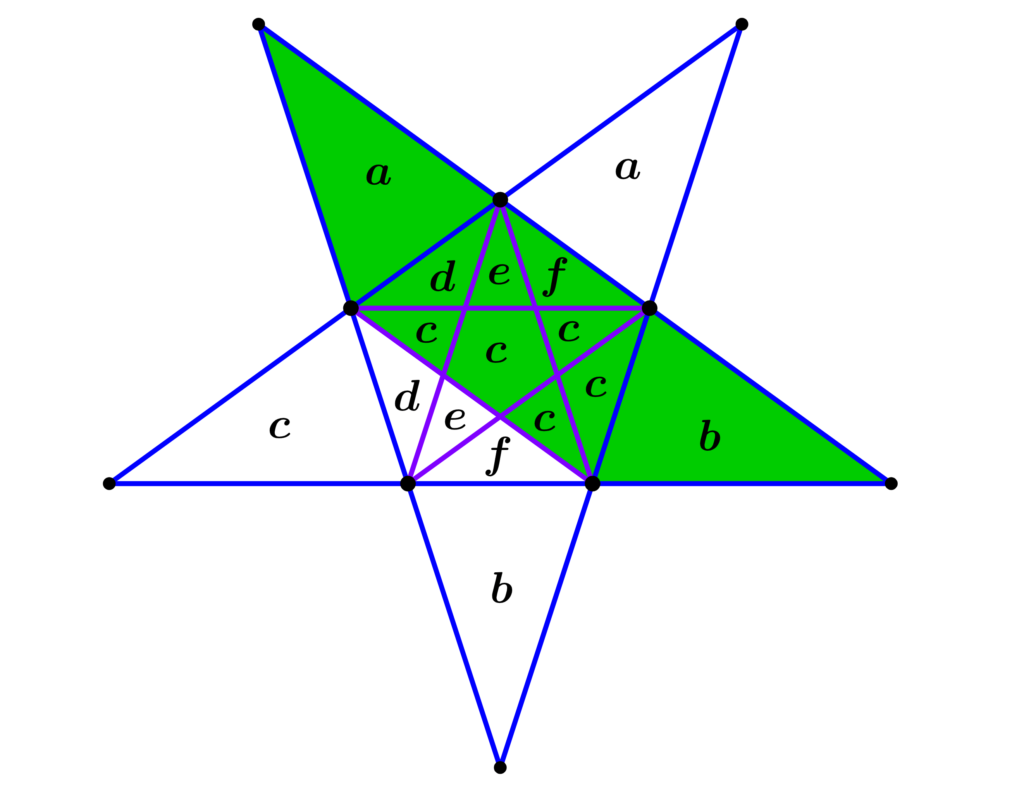
Because of this, the area of the green region is exactly half the area of the entire figure.
EXAMPLE 3
A regular octagon with a perimeter of 8 units is stellated to form a star as shown in the following image. What is the perimeter of the star?
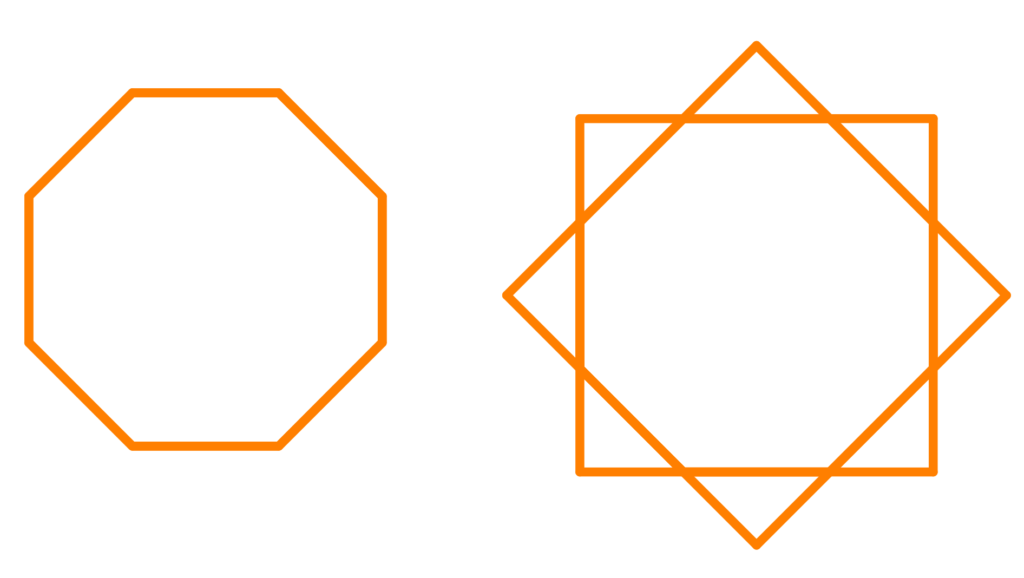
Solution: 8√2
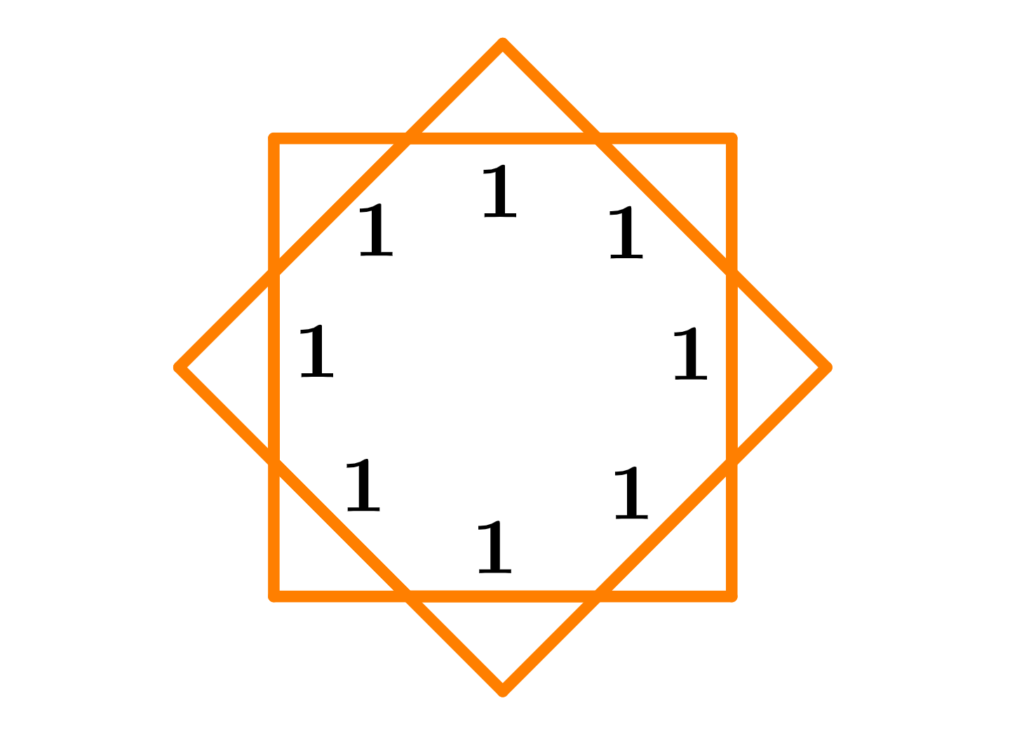
Since the octagon is regular, each of the outer triangles is an isosceles right triangle with a hypotenuse of 8/8 = 1.
The sides of the star are congruent. We can use x to represent the length of its sides and using the Pythagorean theorem, we have:
$latex {{x}^2}+{{x}^2}={{1}^2}$
$latex {{2x}^2}=1$
$latex {{x}^2}=\frac{1}{2}$
$latex x=\frac{1}{\sqrt{2}}=\frac{\sqrt{2}}{2}$
Since the star has 16 sides, it means that the perimeter is $latex 16 \times \frac{\sqrt{2}}{2} = 8 \sqrt{2}$.
See also
Interested in learning more about similar figures, congruency, and other geometry topics? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
