The Pythagorean theorem has a large number of applications in various areas. For example, in architecture and construction, the Pythagorean theorem can be used to find lengths of various objects that form right angles. In topography, the steepness of hills or mountains is calculated using this theorem.
Additionally, we can use the Pythagorean theorem in navigation to find the shortest distance between two points and the angle to follow.
GEOMETRY
Relevant for…
Learning about some of the applications of the Pythagorean theorem.
GEOMETRY
Relevant for…
Learning about some of the applications of the Pythagorean theorem.
Architecture and construction
The Pythagorean theorem allows us to calculate the length of the diagonal that connects two straight lines that form a right angle. This application is frequently used in architecture to find the lengths of different objects to be used in the building or construction.
Additionally, this is also useful in woodworking and other physical object construction projects that require precise measurements. For example, an architect could design a sloped roof.
By knowing the height of the roof, as well as its length, you can easily determine the length of the roof slope using the Pythagorean theorem.
Surveying
Surveying is the process that cartographers use to calculate the distances and heights of different points to make maps with high precision. Given that the terrain is most often uneven, surveyors need to use systematic methods to find distance measurements.
With the Pythagorean theorem, it is possible to calculate the inclination of mountains and hills. A surveyor uses a telescope to look at a measuring rod that is positioned at a fixed distance so that the point of view of the telescope and the measuring rod are at right angles.
Since the surveyor knows the exact distance to the location of the bar and its height, he can use the Pythagorean theorem to calculate the length of the slope and the measure of its steepness.
Navigation
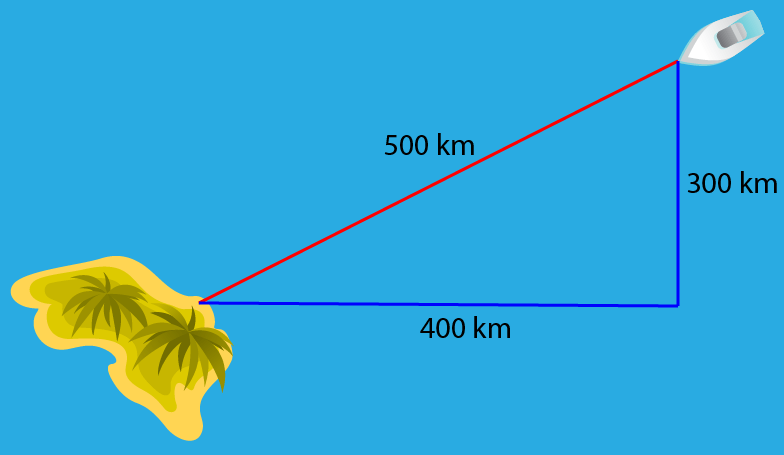
The Pythagorean theorem can be used to navigate the two-dimensional plane. Specifically, we can use the theorem to find the shortest distance between two points.
For example, if we were sailing in the ocean, and we wanted to move to an island that is 300 kilometers to the south and 400 kilometers to the west, we can calculate the shortest distance between the points and even find the angle in the southwest that we have to continue to get to the island.
The south and west distances will be the legs of a right triangle and the shortest distance is the hypotenuse of the triangle.
The same principle can be used for aerial navigation. For example, if the aircraft is close to the landing airport, we can use its height from the ground and its horizontal distance from the airport to find the correct place where we need to start descending.
Laying out square angles
The Pythagorean theorem can be used in the construction of houses or buildings to make sure that the constructions are square. This is because a triangle that has sides that correspond to the Pythagorean theorem, such as a 3 meters by 4 meters by 5 meters triangle, will always be a right triangle.
Therefore, when working on the foundation or building a square corner between two walls, builders can form a triangle with three strings corresponding to these lengths. As long as the strings have been measured correctly, the corner opposite the hypotenuse of the triangle corresponds to a right angle, so builders can follow those lines to build a square corner.
See also
Interested in learning more about the Pythagorean theorem? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
