The apothem of a hexagon is the length of the line that joins the center of the hexagon with the center of one side. The apothem is the perpendicular line that connects the center of the hexagon with one side. The apothem can be very useful when we want to find the area of a hexagon since it allows us to use a simpler formula.
We can calculate the apothem by dividing the hexagon into six congruent triangles and using one of the triangles. Then, we use the Pythagorean theorem or trigonometry to derive different formulas.
Formula to find the apothem of a hexagon
There are two main methods that we can use to derive a formula for the apothem of a hexagon. Both methods involve dividing the hexagon into six congruent triangles as in the following image:
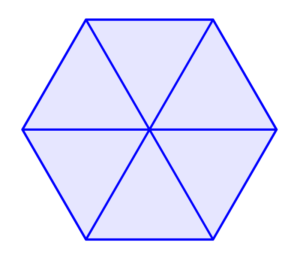
First method: We can use the Pythagorean theorem on one of the triangles formed. We know that the triangles formed are equilateral, so their three sides are equal. Also, drawing the apothem, we see that it divides the base into two equal parts as in the image:
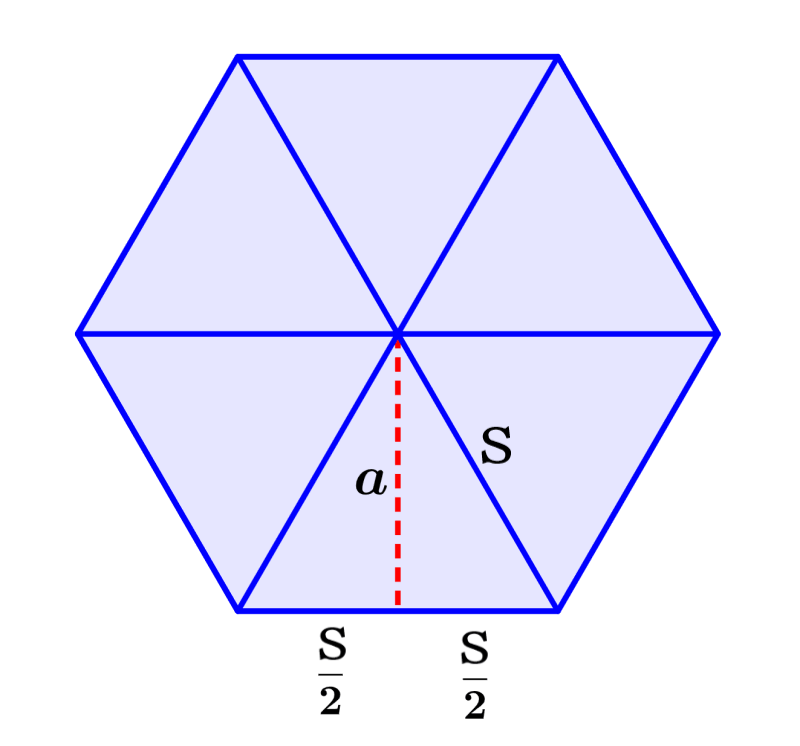
Therefore, using these lengths, we have:
$latex {{s}^2}={{a}^2}+{{(\frac{s}{2})}^2}$
$latex {{a}^2}={{s}^2}-{{(\frac{s}{2})}^2}$
$latex {{a}^2}={{s}^2}-\frac{{{s}^2}}{4}$
$latex {{a}^2}=\frac{3{{s}^2}}{4}$
| $latex a=\frac{\sqrt{3}s}{2}$ |
Second method: Similar to the previous method, we divide the hexagon into six congruent triangles. When plotting the apothem, we divide the triangle in half, which means that if we did this with all the triangles, we would have 12 small triangles in total.
To use trigonometry, we have to find the central angle. Since the total central angle equals 360°, the central angle of each small triangle measures $latex 360 \div 12 = 30$°:
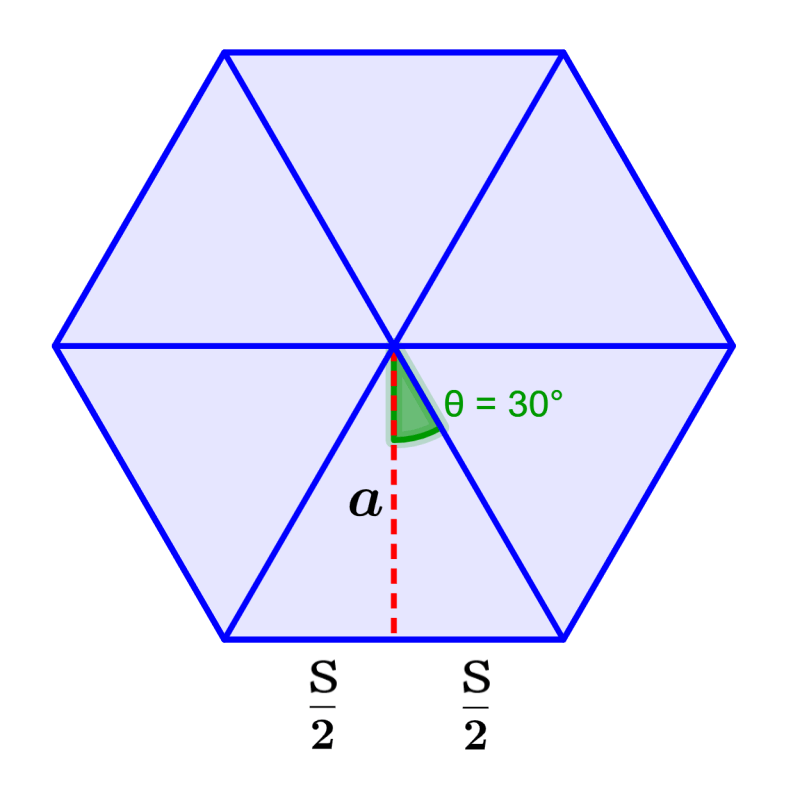
Now, we can use the tangent. We know that the tangent of an angle is equal to the opposite side over the adjacent side:
$latex \tan(30°)=\frac{\text{opposite}}{\text{adjacent}}$
$latex \tan(30°)=\frac{\frac{s}{2}}{a}$
$latex \tan(30°)=\frac{s}{2a}$
| $latex a=\frac{s}{2\tan(30°)}$ |
Apothem of a hexagon – Examples with answers
The following examples use both formulas for the apothem of a hexagon seen above. Each example has its respective solution, but it is recommended that you try to solve the exercises yourself before looking at the result.
EXAMPLE 1
What is the length of the apothem of a hexagon that has sides of length 6 m?
Solution
First formula: Using the first formula with $latex s = 6$, we have:
$latex a=\frac{\sqrt{3}s}{2}$
$latex a=\frac{\sqrt{3}(6)}{2}$
$latex a=5.2$
The length of the apothem is 5.2 m.
Second formula: Using the second formula with $latex s= 6$, we have:
$latex a=\frac{s}{2\tan(30°)}$
$latex a=\frac{6}{2\tan(30°)}$
$latex a=5.2$
We see that we got the same result using both formulas.
EXAMPLE 2
A hexagon has sides of length 8 m. What is the length of its apothem?
Solution
First formula: We can use $latex s = 8$ in the first formula:
$latex a=\frac{\sqrt{3}s}{2}$
$latex a=\frac{\sqrt{3}(8)}{2}$
$latex a=6.93$
The length of the apothem is 6.93 m.
Second formula: If we now use the second formula with $latex s = 8$, we have:
$latex a=\frac{s}{2\tan(30°)}$
$latex a=\frac{8}{2\tan(30°)}$
$latex a=6.93$
We got the same length with both formulas.
EXAMPLE 3
What is the length of the apothem of a hexagon with sides of length 10 m?
Solution
First formula: Using the first formula with $latex s = 10$, we have:
$latex a=\frac{\sqrt{3}s}{2}$
$latex a=\frac{\sqrt{3}(10)}{2}$
$latex a=8.66$
The length of the apothem is 8.66 m.
Second formula: Using the second formula with $latex s=10$, we have:
$latex a=\frac{s}{2\tan(30°)}$
$latex a=\frac{10}{2\tan(30°)}$
$latex a=8.66$
The same result was obtained with both formulas.
EXAMPLE 4
What is the length of the sides of a hexagon that has an apothem of 7.5 m?
Solution
In this case, we have the length of the apothem and we want to find the length of the sides of the hexagon.
First formula: We use the first formula with $latex a = 7.5$ and solve for s:
$latex a=\frac{\sqrt{3}s}{2}$
$latex 7.5=\frac{\sqrt{3}s}{2}$
$latex 15=\sqrt{3}s$
$latex s=8.66$
The length of the sides of the hexagon is 8.66 m.
Second formula: We use the second formula with $latex a = 7.5$ and solve for s:
$latex a=\frac{s}{2\tan(30°)}$
$latex 7.5=\frac{s}{2\tan(30°)}$
$latex s=7.5(2\tan(30°))$
$latex s=8.66$
We got the same length with both formulas.
EXAMPLE 5
What is the length of the sides of a hexagon that has an apothem of length 12 m?
Solution
Again, we use both formulas and solve for s.
First formula: We substitute $latex a=12$ and solve for s:
$latex a=\frac{\sqrt{3}s}{2}$
$latex 12=\frac{\sqrt{3}s}{2}$
$latex 24=\sqrt{3}s$
$latex s=13.87$
The length of the sides of the hexagon is 13.87 m.
Second formula: We substitute $latex a=12$ and we solve for s:
$latex a=\frac{s}{2\tan(30°)}$
$latex 12=\frac{s}{2\tan(30°)}$
$latex s=12(2\tan(30°))$
$latex s=13.86$
In this case, we got a small difference in the decimals. This is due to the rounding used when performing the operations.
Apothem of a hexagon – Practice problems
Practice using the formulas of the hexagon apothem and solve the following problems. Select an answer and click “Check” to check your answer. If you need help with this, you can look at the solved examples above.
See also
Interested in learning more about parallelograms? Take a look at these pages:




