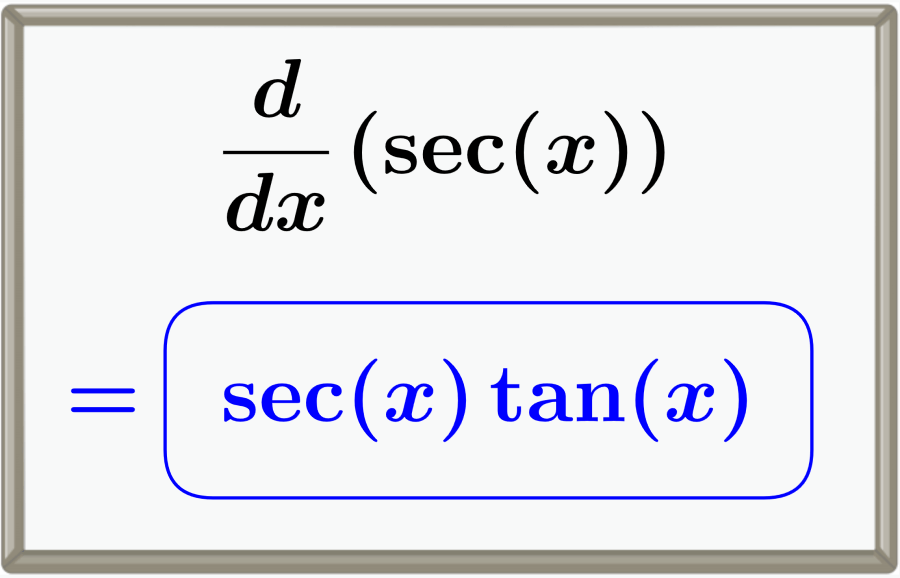
The derivative of the secant function is secant times tangent, sec(x)tan(x). We can prove this derivative using limits and trigonometric identities.
In this article, we will learn how to derive the trigonometric secant function. We will cover a demonstration of the derivative, a comparison of graphs of the secant and its derivative, and some examples.
Proof of the Derivative of the Secant Function
The trigonometric function secant of an angle is defined as the ratio of hypothenuse to the adjacent side of an angle in a right triangle. Illustrating it through a figure, we have
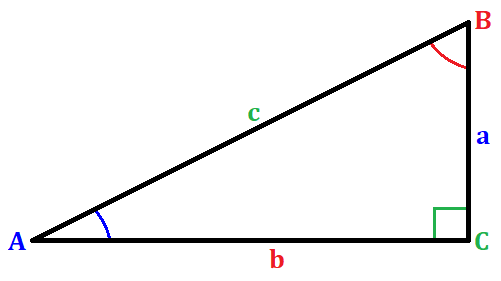
where C is 90°. For the sample right triangle, getting the secant of angle A can be evaluated as
$latex \sec{(A)} = \frac{c}{b}$
where A is the angle, c is the hypothenuse, and b is its adjacent side.
Before learning the proof of the derivative of the secant function, you are hereby recommended to learn the Pythagorean theorem, Soh-Cah-Toa & Cho-Sha-Cao, and the first principle of limits as prerequisites.
To review, any function can be derived by equating it to the limit of
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\frac{f(x+h)-f(x)}{h}}$$
Suppose we are asked to get the derivative of
$latex f(x) = \sec{(x)}$
we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\frac{ \sec{(x+h)} – \sec{(x)} }{h}}$$
Analyzing our equation, we can observe that both the first and second terms in the numerator of the limit is a secant of a sum of two angles x and h and a secant of angle x. With this observation, we can try to apply the defining relation identities for secant and cosine. Applying this, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\frac{ \frac{1}{\cos{(x+h)}} – \frac{1}{\cos{(x)}} }{h}}$$
Algebraically re-arranging by applying some rules of fraction, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\frac{ \frac{ \cos{(x)} – \cos{(x+h)} }{\cos{(x+h)}\cos{(x)}} }{h}}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} { \frac{ \cos{(x)} – \cos{(x+h)} }{h\cos{(x+h)}\cos{(x)}} }$$
Looking at the re-arranged numerator, we can try to apply the product-sum identities of cosine.
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ -2\sin{\left(\frac{x+(x+h)}{2}\right)} \sin{\left(\frac{x-(x+h)}{2}\right)} }{h\cos{(x+h)}\cos{(x)}} \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ -2\sin{\left(\frac{x+x+h}{2}\right)} \sin{\left(\frac{x-x-h}{2}\right)} }{h\cos{(x+h)}\cos{(x)}} \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ -2\sin{\left(\frac{2x+h}{2}\right)} \sin{\left(\frac{-h}{2}\right)} }{h\cos{(x+h)}\cos{(x)}} \right)}$$
Based on the trigonometric identities of a sine of a negative angle, it is equal to negative sine of that same angle but in positive form. Applying this to the second multiplicand of the numerator, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ -2\sin{\left(\frac{2x+h}{2}\right)} \cdot \left( -\sin{\left(\frac{h}{2}\right)} \right) }{h\cos{(x+h)}\cos{(x)}} \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ 2\sin{\left(\frac{2x+h}{2}\right)} \sin{\left(\frac{h}{2}\right)} }{h\cos{(x+h)}\cos{(x)}} \right)}$$
Re-arranging algebraically and by applying the limit of product of two functions, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{ \sin{\left(\frac{2x+h}{2}\right)} \cdot 2\sin{\left(\frac{h}{2}\right)} }{\cos{(x+h)}\cos{(x)} \cdot h} \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+h}{2}\right)} }{ \cos{(x+h)}\cos{(x)} } \cdot \frac{ 2\sin{\left(\frac{h}{2}\right)} }{h} \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+h}{2}\right)} }{ \cos{(x+h)}\cos{(x)} } \right)} \cdot \lim \limits_{h \to 0} {\left( \frac{ 2\sin{\left(\frac{h}{2}\right)} }{h} \right)}$$
Applying some rules of fractions to the second multiplicand, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+h}{2}\right)} }{ \cos{(x+h)}\cos{(x)} } \right)} \cdot \lim \limits_{h \to 0} {\left( \frac{ \sin{\left(\frac{h}{2}\right)} }{ \frac{h}{2} } \right)}$$
In accordance with the limits of trigonometric functions, the limit of the trigonometric function $latex \sin{(\theta)}$ to $latex \theta$ as $latex \theta$ approaches zero is equal to one. The same can be applied to $latex \sin{\left(\frac{h}{2}\right)}$ over $latex \frac{h}{2}$. Applying, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+h}{2}\right)} }{ \cos{(x+h)}\cos{(x)} } \right)} \cdot \lim \limits_{h \to 0} {1}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+h}{2}\right)} }{ \cos{(x+h)}\cos{(x)} } \right)}$$
Finally, we have successfully made it possible to evaluate the limit of whatever is left in the equation. Evaluating by substituting the approaching value of $latex h$, we have
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x+(0)}{2}\right)} }{ \cos{(x+(0))}\cos{(x)} } \right)}$$
$$\frac{d}{dx} f(x) = \lim \limits_{h \to 0} {\left( \frac{\sin{\left(\frac{2x}{2}\right)} }{ \cos{(x)}\cos{(x)} } \right)}$$
$$\frac{d}{dx} f(x) = \frac{\sin{(x)} }{ \cos{(x)}\cos{(x)} }$$
Applying some trigonometric identities to simplify the derivative formula by the use of defining relation identities, we have
$$\frac{d}{dx} f(x) = \frac{ \sin{(x)} }{ \cos{(x)} } \cdot \frac{1}{\cos{(x)}} $$
$$\frac{d}{dx} f(x) = \tan{(x)} \cdot \sec{(x)} $$
$$\frac{d}{dx} f(x) = \sec{(x)} \tan{(x)}$$
Therefore, the derivative of the trigonometric function ‘secant‘ is:
$$\frac{d}{dx} (\sec{(x)}) = \sec{(x)} \tan{(x)}$$
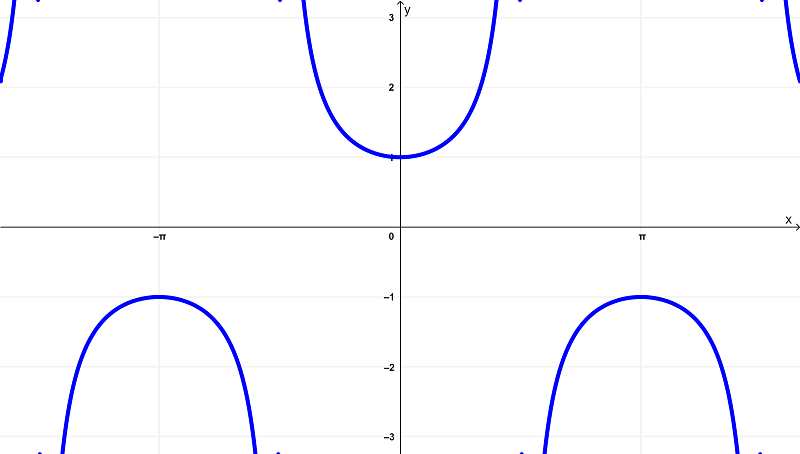
Graph of Secant of x VS. The Derivative of Secant of x
The graph of the function
$latex f(x) = \sec{(x)}$
is
Deriving $latex f(x) = \sec{(x)}$, we get
$latex f'(x) = \sec{(x)}\tan{(x)}$
which is illustrated graphically as
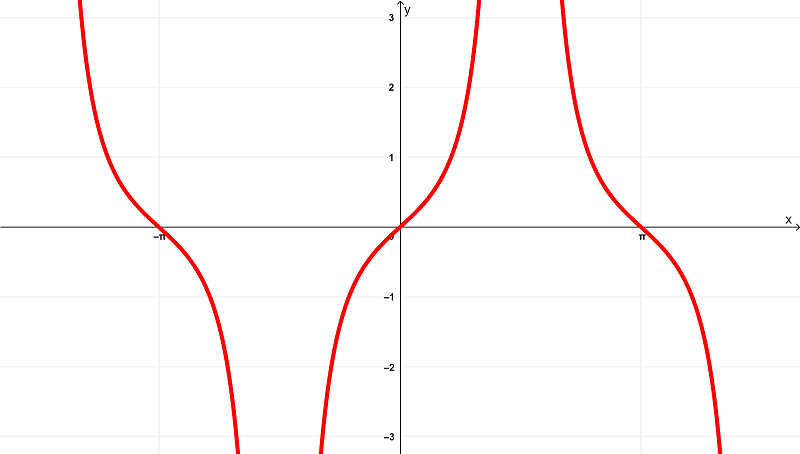
Making a comparison of their graphs, we have
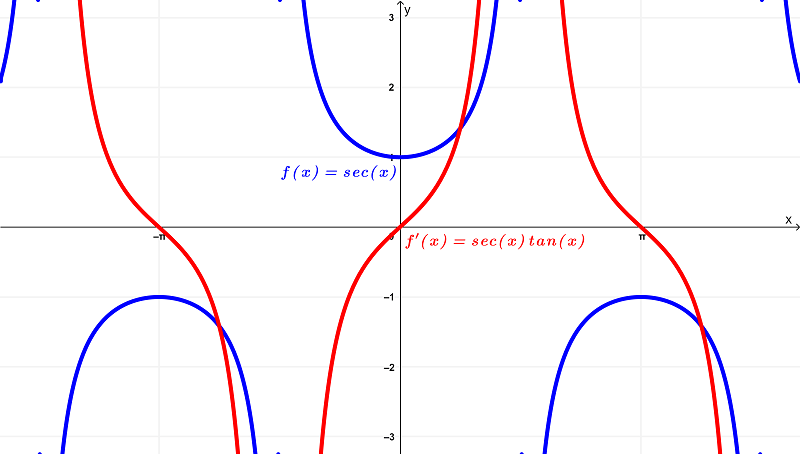
We can observe that the original function $latex f(x) = \sec{(x)}$ has a domain of
$latex \left(-\frac{3\pi}{2},-\frac{\pi}{2}\right) \cup \left(-\frac{\pi}{2},\frac{\pi}{2}\right) \cup \left(\frac{\pi}{2},\frac{3\pi}{2}\right)$
within the finite intervals of
$latex \left(-\frac{3\pi}{2},\frac{3\pi}{2}\right)$
and exists within the range of
$latex (-\infty,-1] \cup [1,\infty)$
whereas the derivative $latex f'(x) = \sec{(x)}\tan{(x)}$ has a domain of
$latex \left(-\frac{3\pi}{2},-\frac{\pi}{2}\right) \cup \left(-\frac{\pi}{2},\frac{\pi}{2}\right) \cup \left(\frac{\pi}{2},\frac{3\pi}{2}\right)$
within the finite intervals of
$latex \left(-\frac{3\pi}{2},\frac{3\pi}{2}\right)$
and exists within the range of
$latex (-\infty,\infty)$ or all real numbers
Examples
The following are some examples of how to derive compound secant functions with the chain rule.
EXAMPLE 1
Find the derivative of $latex f(x) = \sec(10x)$.
Solution
The given secant function is a composite function since it is a secant of the function $latex 10x$. Therefore, we are going to use the chain rule.
If $latex u=10x$ is the inner function, we have $latex f(u)=\sec(u)$ and using the chain rule, we have:
$$\frac{dy}{dx}=\frac{dy}{du} \frac{du}{dx}$$
$$\frac{dy}{dx}=\sec(u)\tan(u) \times 10$$
Finally, we plug $latex u=10x$ back into the function and we have:
$$\frac{dy}{dx}=10\sec(10x)\tan(10x)$$
EXAMPLE 2
Find the derivative of $latex F(x) = \sec(8x^2-4)$
Solution
Since we have a composite secant function, we have to use the chain rule to derive.
We start by expressing the secant function as $latex f (u) = \sec(u)$, where $latex u = 8x^2-4$.
Then, we calculate the derivative of the exterior function $latex f(u)$, that is, the derivative of the secant function in terms of $latex u$:
$$\frac{d}{du} ( \sec{(u)} ) = \sec(u)\tan(u)$$
Now, we calculate the derivative of the inner function $latex g(x)$ or $latex u$:
$$\frac{d}{dx}(g(x)) = \frac{d}{dx}(8x^2-4)$$
$$\frac{d}{dx}(g(x)) = 16x$$
Using the chain rule, we multiply the derivative of the outer function by the derivative of the inner function:
$$\frac{dy}{dx} = \frac{d}{du} (f(u)) \cdot \frac{d}{dx} (g(x))$$
$$\frac{dy}{dx} = \sec(u)\tan(u) \cdot 16x$$
Finally, we substitute $latex u=8x^2-4$ back:
$$\frac{dy}{dx} = \sec(8x^2-4)\tan(8x^2-4) \cdot 16x$$
$$\frac{dy}{dx} = 16x\sec(8x^2-4)\tan(8x^2-4)$$
EXAMPLE 3
What is the derivative of $latex f(x) = \sec(\sqrt{x})$?
Solution
We can derive this function by considering $latex u=\sqrt{x}$ as the inner function.
Therefore, we start by writing $latex u=\sqrt{x}$ as $latex u=x^{\frac{1}{2}}$ to find the derivative of $latex u$ in terms of $latex x$:
$$\frac{du}{dx}=\frac{1}{2}x^{-\frac{1}{2}}$$
Now, we consider that $latex f(u)=\sec(u)$ and use the chain rule:
$$\frac{dy}{dx}=\frac{dy}{du} \frac{du}{dx}$$
$$\frac{dy}{dx}=\sec(u)\tan(u) \times \frac{1}{2}x^{-\frac{1}{2}}$$
Substituting $latex u=\sqrt{x}$ back and simplifying, we have:
$$\frac{dy}{dx}=\sec(\sqrt{x})\tan(\sqrt{x}) \times \frac{1}{2}x^{-\frac{1}{2}}$$
$$\frac{dy}{dx}=\frac{1}{2\sqrt{x}}\sec(\sqrt{x})\tan(\sqrt{x})$$
Practice of derivatives of composite secant functions
See also
Interested in learning more about the derivatives of trigonometric functions? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
