Cotangent Calculator (Degrees and Radians)
Result:
Graph of cotangent
With this calculator, you can get the cotangent of any entered angle. You can use degrees, radians, and π radians. When you enter an angle, the calculator will display its cotangent immediately.
Below you can find additional information about using the cotangent calculator. In addition, you can also learn about the definition, graph, and important values of the cotangent.
How to use the cotangent calculator?
Step 1: Start by selecting the type of angle you want to use. By clicking the blue button, you will be able to select between degrees, radians, and π radians.
Step 2: Enter the angle in the corresponding input box. It is possible to use positive and negative angles.
Step 3: The cotangent of the entered angle will be displayed in the right panel.
Difference between using degrees, radians and π radians in calculator
To find the relationship between degrees and radians, we can remember that a complete revolution has a total of 360° or 2π radians. This means that 180° is equivalent to π radians.
The difference between π radians and radians, on the other hand, is simply that by using “π radians” in the calculator, we will be multiplying whatever value is entered by π. For example, if we enter 1.5, we will be calculating the cotangent of 1.5π radians.
Therefore, we can enter 0.5π into the calculator, by selecting the “π radians” option and simply entering 0.5. However, we can also select the “radians” option and enter 1.571, which is equivalent to 0.5π, since π has a value of approximately 3.1415…
What is the cotangent of an angle?
The cotangent is the reciprocal function of the tangent. This means that the cotangent is equal to 1 over the tangent of an angle. Considering that the tangent is equal to sine over cosine, the cotangent is equal to cosine over sine.
In addition, we can also define the cotangent relative to the sides of a right triangle. Doing this, we have that the cotangent of an angle is equal to the length of the side adjacent to the angle divided by the length of the side opposite the angle.
For example, in the following right triangle, we can define the cotangent of angle A as the length of side b (side adjacent to angle A) divided by the length of side a (side opposite angle A).
Similarly, we can define the cotangent of angle B as the length of side a (side adjacent to angle B) divided by the length of side b (side opposite angle B).
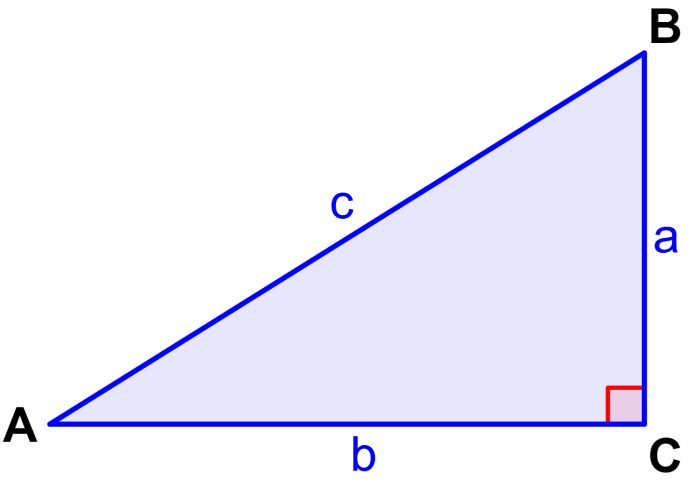
If you want to learn more about the cotangent of an angle, you can visit our article Cotangent of an Angle – Formulas and Examples .
Why is the Cotangent of 0° and 180° undefined?
The cotangent function is the reciprocal of the tangent and will be undefined when the tangent of an angle equals 0. This is because we cannot have denominators equal to 0.
Since the cotangent can be defined as cosine over the sine of the angle, it will be undefined whenever the sine of the angle is equal to 0. This happens when we have an angle of 0.
Also, because the sine function is periodic, that value repeats every time we add 180°n, where n is any positive or negative integer.
For example, the sine of 0°+180°(2)=360°, is also equal to 0, so the cotangent of 360° is undefined.
Graph of the cotangent of an angle
The cotangent can be graphed by considering angles that are outside of a right triangle. That is, we can use both positive and negative angles that are greater than 180°.
The cotangent function is periodic. This means that its graph repeats itself after a fixed interval. The period of the cotangent function is equal to 180° or π radians.
Domain of the cotangent of an angle
We can use the graph of the cotangent to find its domain. From the graph, we can deduce that the function extends from negative infinity to positive infinity.
However, the cotangent function has asymptotes, at which the function approaches infinity and becomes undefined. Asymptotes are located every 180° starting from 0. Therefore, the domain of the cotangent is all real numbers except 180°n or πn, where n is a positive or negative integer.
Range of the cotangent of an angle
In the graph of the cotangent, we can see that the function can result in any value, both positive and negative. We do not have any restrictions on the range.
Therefore, the range of the cotangent is equal to all real numbers.
Table of the cotangent of common angles
| Degrees | Radians | Cotangent |
| 90° | 0 | |
| 60° | ||
| 45° | 1 | |
| 30° | ||
| 0° | 0 | Undefined |
Related calculators:
- Cosine Calculator (Degrees and Radians)
- Sine Calculator (Degrees and Radians)
- Tangent Calculator (Degrees and Radians)
- Secant Calculator (Degrees and Radians)
- Cosecant Calculator (Degrees and Radians)
You can explore other calculators here.
