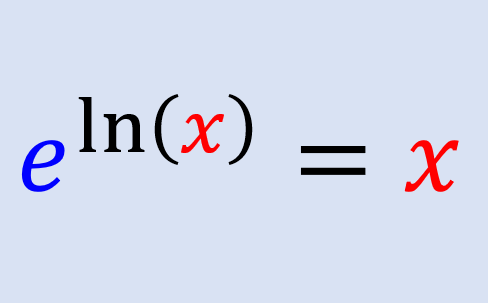With the laws of natural logarithms, we can simplify logarithmic expressions and rewrite them in more convenient ways. We have eleven main laws of natural logarithms. With these eleven laws, we can expand natural logarithms, condense them, and solve logarithmic equations. Although these laws are specified for natural logarithms, the laws of logarithms apply to logarithms of any base.
Here, we will look at the eleven main laws of logarithms. In addition, we will solve some logarithm exercises to apply these laws.
What are the laws of natural logarithms?
Recall that a natural logarithm is a logarithm with base e. The number e is called the natural constant and has an approximate value of 2.71828. The natural logarithm is denoted as ln. The number e has many applications in various areas such as mathematics, economics, among others.
Given that e appears in many areas, many people need to calculate the logarithm with a base of e frequently, so there was a need to create a shortcut with the natural logarithm and define it as a logarithm with base e.
The following are the most important laws of natural logarithms:
1. Product law
If we have a natural logarithm of a product, we can write it as the sum of the logarithms of each factor separately:
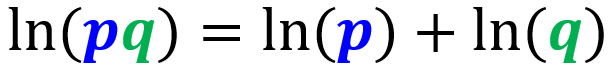
2. Quotient law
If we have a natural logarithm of a quotient, we can rewrite it as the natural logarithm of the numerator minus the natural logarithm of the denominator:
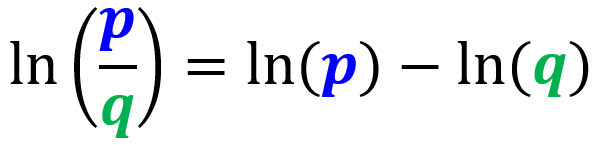
3. Power law
The natural logarithm of an exponential argument can be rewritten as the exponent multiplied by the logarithm of the base (without the exponent):

4. Law of the logarithm of the reciprocal
The natural logarithm of the reciprocal of x is the opposite of the natural logarithm of x:
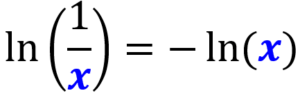
5. Law of the natural logarithm of 1
The natural logarithm of 1 is equal to zero:
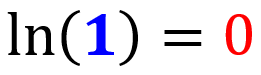
6. Law of the natural logarithm of e
The natural logarithm of the natural number, e, is equal to 1:
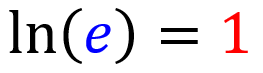
7. Law of the natural logarithm of zero
The natural logarithm of zero is undefined:

8. Law of the natural logarithm of a negative number
The natural logarithm of a negative number is also undefined.
9. Law of the natural logarithm of infinity
The natural logarithm of infinity is equal to infinity:
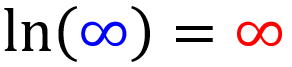
10. Law of the natural logarithm of exponential e
The natural logarithm of exponential e is equal to the exponent of e:

11. Law of e to the natural logarithm
The number e raised to the natural logarithm of a number is equal to the number:
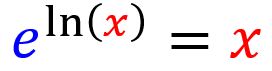
Laws of natural logarithms – Examples with answers
The following examples use the laws of natural logarithms described above. It is recommended that you try to solve the exercises yourself before looking at the answer.
EXAMPLE 1
Simplify the following expression: $latex \ln(8)+\ln(10)$.
Solution
We have a sum of logarithms, so we can apply the product law to simplify:
$latex \ln(8)+\ln(10)=\ln(8\times 10)$
$latex =\ln(80)$
We can leave the logarithm in that form or solving it, we have
$latex \ln(80)\approx 4.382$
EXAMPLE 2
Simplify the logarithmic expression: $latex \ln(72)-\ln(3)$.
Solution
In this case, we have a subtraction of natural logarithms, so we can apply the quotient law:
$latex \ln(72)-\ln(3)=\ln(\frac{72}{3})$
$latex =\ln(24)$
We can leave the natural logarithm as this or we can apply it:
$latex \ln(24)\approx 3.178$
EXAMPLE 3
What is the value of $latex \ln({{e}^{15}})$?
Solution
Here, we can use the law of the natural logarithm of e exponential. This law tells us that when we have the natural logarithm of e raised to an exponent, this is equivalent to the exponent:
$latex \ln({{e}^{15}})=15$
EXAMPLE 4
Simplify the following expression: $latex \ln(100)-\ln(5)+\ln(4)$.
Solution
In this expression, we have subtractions and additions of natural logarithms. We can form a single logarithm by applying both the quotient law and the product law. Therefore, we have:
$latex \ln(100)-\ln(5)+\ln(4)=\ln(\frac{100 \times 4}{5})$
$latex =\ln(\frac{400}{5})$
$latex =\ln(80)$
Now, we can leave the logarithm like this or we can calculate it to get:
$latex \ln(80)\approx 4.382$
EXAMPLE 5
Simplifiy the expression: $latex 2\ln(5)+\ln(40)-3\ln(10)$.
Solution
We start by using the power law to rewrite the natural logarithms.
$latex 2\ln(5)+\ln(40)-3\ln(10)$
$latex =\ln({{5}^2})+\ln(40)-\ln({{10}^3})$
$latex =\ln(25)+\ln(40)-\ln(1000)$
Now, we can use the product law and the quotient law to obtain a single logarithm:
$latex =\ln(\frac{25\times 40}{1000})$
$latex =\ln(1)$
Finally, we apply the law of the natural logarithm of 1 to solve:
$latex \ln(1)=0$
EXAMPLE 6
Write the expanded version of the natural logarithm: $latex\ln({{e}^3}{{x}^3}{{y}^4})$.
Solution
We can apply the product law to separate the factors and form an addition of natural logarithms:
$latex \ln({{e}^3}{{x}^3}{{y}^4})=\ln({{e}^3})+\ln({{x}^3})+\ln({{y}^4})$
Now, we can use the power law to write the exponents as a multiplication of each individual logarithm:
$latex =3\ln(e)+3\ln(x)+4\ln(y)$
$latex =3(1)+3\ln(x)+4\ln(y)$
$latex =3+3\ln(x)+4\ln(y)$
Laws of natural logarithms – Practice problems
Test your knowledge of the laws of natural logarithms by solving the following problems. If you need help, you can look at the list of laws of natural logarithms or the solved examples above.
See also
Interested in learning more about natural logarithms? Take a look at these pages: