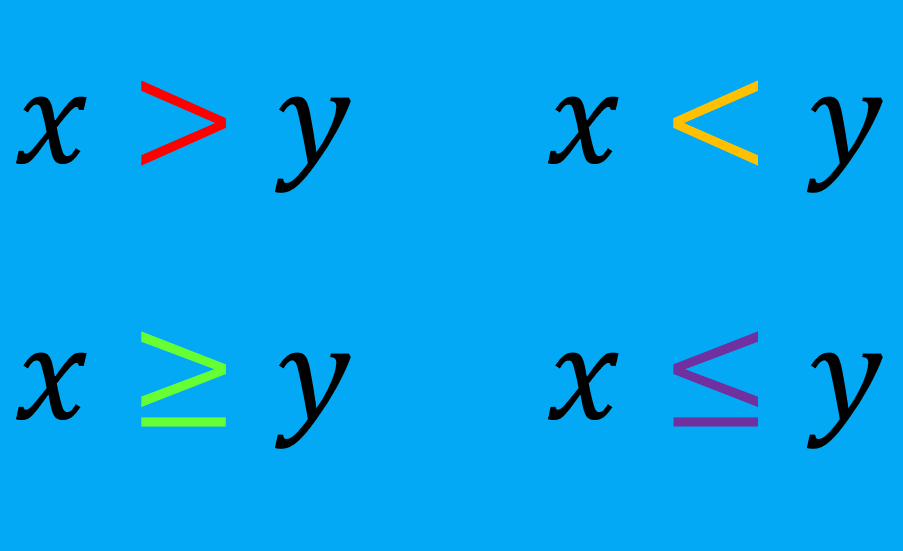Inequalities with absolute value follow the same rules as an absolute value in numbers; the difference is that in inequalities we have a variable. In this article, we will look at a brief introduction to inequalities with absolute value and we will learn a method for solving problems related to inequalities with absolute value.
Finally, we will look at some examples with answers to improve our understanding of the concepts.
What are inequalities with absolute value
Before we analyze inequalities with absolute value, let’s start by looking at a brief summary of the absolute value of a number.
Let’s start with the definition: the absolute value of a number is the distance of a value from the origin regardless of direction. The absolute value is denoted by two vertical lines that enclose the number or expression.
For example, the absolute value of x is expressed as $latex |x|=a$, which means that $latex x=+a$ and $latex x=-a$. Now, let’s see what inequalities with absolute value mean.
An inequality with absolute value is an expression that contains the absolute value function, as well as the absolute value signs. For example, the expression $latex | x +5|> 2$ is an inequality with an absolute value that contains a “greater than” sign.
We have four different inequality symbols: greater than (>), less than (<), greater than or equal to (≥), and less than or equal to (≤).
EXAMPLES
The following are inequalities with absolute value:
- $latex \left| x+1\right|<3$
- $latex \left| x-2\right|≥5$
- $latex \left| x+5\right|>1$
How to solve inequalities with absolute value?
The steps to solve inequalities with absolute value are similar to the steps to solve equations, with the difference that we have to take into account a little extra information to solve the inequalities.
The following steps are general rules that can be followed to solve these types of algebraic problems:
Step 1: Completely isolate the expression with the absolute value.
Step 2: Solve the positive and negative versions of the absolute value inequalities.
- When the number on the other side of the inequality sign is negative, we conclude that either all real numbers are solutions or that the inequality has no solution.
- When the number on the other side is positive, we proceed to form a compound inequality by removing the bars from the absolute value.
Step 3: The type of inequality sign determines the format of the compound inequality to be formed.
- If a problem contains greater than or greater/equal than signs, form a compound inequality as follows:
(values within the absolute value sign) <– (the number on the other side) or (values within the absolute value sign)> (the number on the other side)
- In the same way, if a problem contains less than or less/equal than signs, it forms an inequality composed of three parts as follows:
(the number on the other side of the sign)<(values within the absolute value sign)<(the number on the other side of the sign)
Step 4: Solve the inequalities.
Inequalities with absolute value – Examples with answers
EXAMPLE 1
Solve the inequality $latex |x+4|-6<9$.
Step 1: Solve for the absolute value:
$latex |x+4|-6<9$
$latex |x+4|<9+6$
$latex |x+4|<15$
Step 2: Is the number on the other side negative? No, it is a positive number, 15. We move on to step 3.
Step 3: Form a compound inequality: The inequality sign in this problem is a less than sign, so we form a three-part inequality:
$latex -15<x+4<15$
Step 4: Solve the inequality:
$latex -15-4<x<15-4$
$latex -19<x<11$
EXAMPLE 2
Solve the inequality $latex |2x-1|-7≥-3$.
Step 1: Solve for the absolute value:
$latex |2x-1|-7≥-3$
$latex |2x-1|≥-3+7$
$latex |2x-1|≥4$
Step 2: Is the number on the other side negative? No, it is a positive number, 4. We move on to step 3.
Step 3: Form a compound inequality: The inequality sign in this problem is a greater/equal than sign, so we form a compound inequality with the word “or”:
$latex 2x-1≤-4$ o $latex 2x-1≥4$
Step 4::Solve the inequalities:
$latex 2x-1≤-4$ or $latex 2x-1≥4$
$latex 2x≤-3$ or $latex 2x≥5$
$latex x\le -\frac{3}{2}$ or $latex x\ge \frac{5}{2}$
EXAMPLE 3
Solve the inequality $latex |5x+6|+4<1$.
Step 1: Solve for the absolute value:
$latex |5x+6|+4<1$
$latex |5x+6|<1-4$
$latex |5x+6|<-3$
Step 2: Is the number on the other side negative? Yes, it is a negative number, -3. We will look at the signs on each side of the inequality to determine the solution to the problem:
$latex |5x+6|<-3$
positive $latex <$ negative
This statement is never true, so the problem has no solution.
EXAMPLE 4
Solve the inequality $latex |3x-4|+9>5$.
Step 1: Solve for the absolute value:
$latex |3x-4|+9>5$
$latex |3x-4|>5-9$
$latex |3x-4|>-4$
Step 2: Is the number on the other side negative? Yes, it is a negative number, -4. Let’s look at the signs on each side of the inequality to determine the solution to the problem:
$latex |3x-4|>-4$
positive $latex >$ negative
This statement is always true, so the solution to the problem is all real numbers.
Inequalities with absolute value – Practice problems
See also
Interested in learning more about absolute value? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
