The law of sines is an equation that allows us to relate the sines of an angle to their respective opposite sides. The law of sines is applied to find the measures of an angle or the length of a side in a triangle. To use the law of sines, we need to know the measures of two angles and the length of an opposite side or the lengths of two sides and the measure of an opposite angle.
Here, we will do a review of the law of sines and use it to solve some practice problems.
Summary of the law of sines
The law of sines is expressed as follows:
| $latex \frac{a}{\sin(A)}=\frac{b}{\sin(B)}=\frac{c}{\sin(C)}$ |
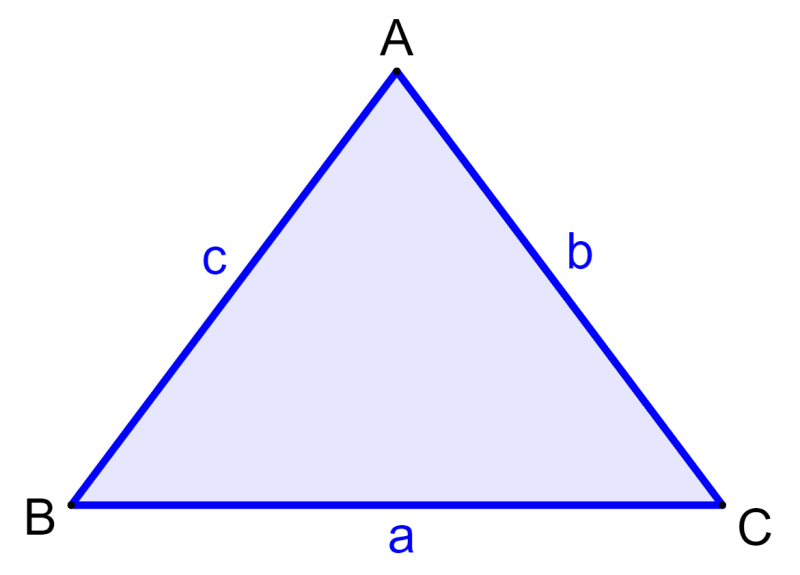
where, a, b, c represent the lengths of the sides of the triangle and A, B, C represent the angles of the triangle. The sides are denoted using lower case letters with respect to their opposite angle.
For example, a represents the side opposite angle A, b represents the side opposite angle B, and c represents the side opposite angle C.
We can apply the law of sines when we have the following situations:
- We have the measure of two angles and the length of one side and we want to calculate the length of another side of the triangle.
- We have the measure of two sides and an angle opposite one of the sides and we want to calculate the measure of the other angle.
For example, in the triangle above, we can use the law of sines if we have the measure of angles A and B and the length of side a and we want to find the length of side b.
Alternatively, we can use the law of sines when we have the lengths of the sides a, b, and the measure of angle B and we want to find the measure of angle A.
Law of sines – Examples with answers
The following examples are solved by applying the law of sines. These examples can be used to study the process used to solve these types of problems. Try to solve the problems yourself before looking at the answer.
EXAMPLE 1
In a triangle, we have the angles A=50° and B=30° and we have the side a=10. Determine the length of side b.
Solution
We can observe the following information:
- A=50°
- B=30°
- a=10
We apply the law of sines together with the given values and solve for b:
$latex \frac{a}{\sin(A)}=\frac{b}{\sin(B)}$
$latex \frac{10}{\sin(50)}=\frac{b}{\sin(30)}$
$latex \frac{10}{0.766}=\frac{b}{0.5}$
$latex 13.05=\frac{b}{0.5}$
$latex b=13.05(0.5)$
$latex b=6.53$
The length of b is 6.53.
EXAMPLE 2
What is the length of c if we have angles B=25° and C=75° and the length of b is 12?
Solution
We observe the following:
- B=25°
- C=75°
- b=12
We substitute these values in the formula of the law of sines:
$latex \frac{b}{\sin(B)}=\frac{c}{\sin(C)}$
$latex \frac{12}{\sin(25)}=\frac{c}{\sin(75)}$
$latex \frac{12}{0.423}=\frac{c}{0.966}$
$latex 28.37=\frac{c}{0.966}$
$latex c=28.37(0.966)$
$latex c=27.4$
The length of c is 27.4.
EXAMPLE 3
What is the measure of angle A if we have a=12, B=40°, and b=8?
Solution
We have the following:
- a=12
- B=40°
- b=8
We substitute these values in the formula of the law of sines:
$latex \frac{a}{\sin(A)}=\frac{b}{\sin(B)}$
$latex \frac{12}{\sin(A)}=\frac{8}{\sin(40)}$
$latex \frac{12}{\sin(A)}=\frac{8}{0.643}$
$latex \frac{12}{\sin(A)}=12.44$
$latex \sin(A)=\frac{12}{12.44}$
Using the inverse sine function, we can find the value of the angle:
$latex A={{\sin}^{-1}}(\frac{12}{12.44})$
$latex A=74.7$°
El ángulo A mide 74.7°.
EXAMPLE 4
If we have the angles A=36° and B=68° in a triangle and we have the length c=11. What is the length of a?
Solution
We observe the following information:
- A=36°
- B=68°
- c=11
We have a side that is not opposite to any of the given angles, so we are going to find the measure of the third angle. For this, we use the fact that the interior angles of a triangle add up to 180°. Therefore, we have:
$latex A+B+C=180$
$latex 36+68+C=180$
$latex C=180-36-68$
$latex C=76$
Now, we apply the law of sines with the values we have:
$latex \frac{a}{\sin(A)}=\frac{c}{\sin(C)}$
$latex \frac{a}{\sin(36)}=\frac{11}{\sin(76)}$
$latex \frac{a}{0.588}=\frac{11}{0.97}$
$latex \frac{a}{0.588}=11.34$
$latex a=11.34(0.588)$
$latex a=6.67$
Side a measures 6.67.
Law of sines – Practice problems
Apply what you have learned about the law of sines to solve the following practice problems. Select an answer and click “Check” to check that you got the correct answer.
See also
Interested in learning more about the law of sines and cosines? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
