In geometry, a sphere is a solid that has a completely round shape defined in three-dimensional space. Mathematically, the sphere is defined as the set of points that are located at a constant distance from a fixed point in three-dimensional space. This constant distance is called the radius and the fixed point is called the center of the sphere. An example of a sphere in real life is a ball.
Here, we will learn about the main characteristics of these geometric figures. Also, we will learn about its most used formulas and we will apply them to solve some problems.
Definition of a sphere
A sphere is a round geometric figure defined in three-dimensional space. The sphere is a three-dimensional solid, so it has a volume and a surface area. Similar to a circle, each point on the sphere is located the same distance from the center.
The shape of a sphere is round and has no faces. The sphere is a three-dimensional geometric figure that has a curved surface. Unlike other solids like the cube, the prism, the pyramid, a sphere does not have any flat surface. The spheres do not have vertices or edges either.
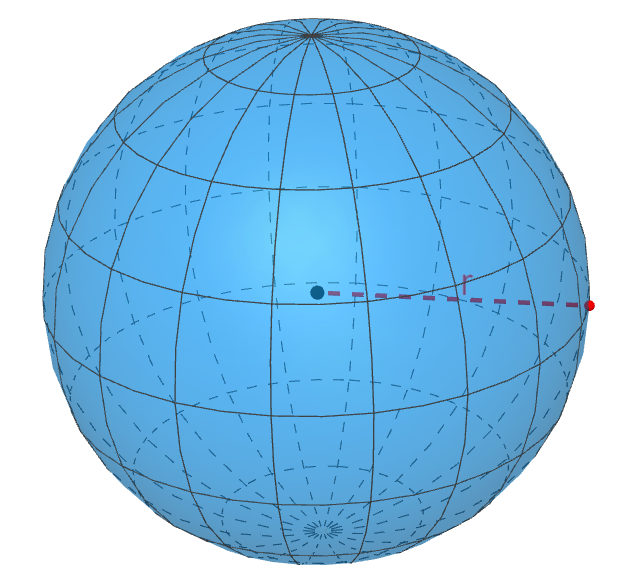
Fundamental characteristics of a sphere
The following are the fundamental characteristics of spheres:
- A sphere is perfectly symmetrical.
- Spheres are not polyhedra.
- All points on the surface of the sphere are equidistant from the center.
- Spheres do not have faces, vertices, or edges.
- The spheres have a constant mean curvature.
- They have a constant width and circumference.
Important sphere formulas
Spheres are three-dimensional figures, so their most important formulas are the volume formula and the surface area formula.
Formula for the volume of a sphere
The volume of a sphere is calculated using the length of its radius. For this, we can use the following formula:
| $latex V=\frac{4}{3}\pi {{r}^3}$ |
where r represents the length of the radius of the sphere.
Formula for the surface area of a sphere
The surface area is equal to the entire outer surface of the sphere. To calculate this area, we also use the length of the radius and the following formula:
| $latex A_{s}=4\pi {{r}^2}$ |
Examples of sphere problems
The formulas seen above are used to solve the following exercises.
EXAMPLE 1
What is the volume of a sphere that has a radius of 4 m?
Solution: We use the volume formula with the value $latex r=4$:
$latex V=\frac{4}{3}\pi {{r}^3}$
$latex V=\frac{4}{3}\pi {{(4)}^3}$
$latex V=\frac{4}{3}\pi (64)$
$latex V=268.1$
The volume is 268.1 m³.
EXAMPLE 2
If a sphere has a radius of 7 m, what is its volume?
Solution: We use the volume formula with $latex r=7$:
$latex V=\frac{4}{3}\pi {{r}^3}$
$latex V=\frac{4}{3}\pi {{(7)}^3}$
$latex V=\frac{4}{3}\pi (343)$
$latex V=1436.8$
The volume is 1436.8 m³.
EXAMPLE 3
A sphere has a radius of 3 m. What is its surface area?
Solution: We have the radius $latex r=3$, so we use this value in the formula for surface area:
$latex A_{s}=4\pi {{r}^2}$
$latex A_{s}=4\pi {{(3)}^2}$
$latex A_{s}=4\pi (9)$
$latex A_{s}=113.1$
The surface area is 113.1 m².
EXAMPLE 4
What is the surface area of a sphere that has a radius of 6 m?
Solution: We use the value $latex r = 6$ in the formula for surface area:
$latex A_{s}=4\pi {{r}^2}$
$latex A_{s}=4\pi {{(6)}^2}$
$latex A_{s}=4\pi (36)$
$latex A_{s}=452.4$
The surface area is 452.4 m².
See also
Interested in learning more about spheres? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
