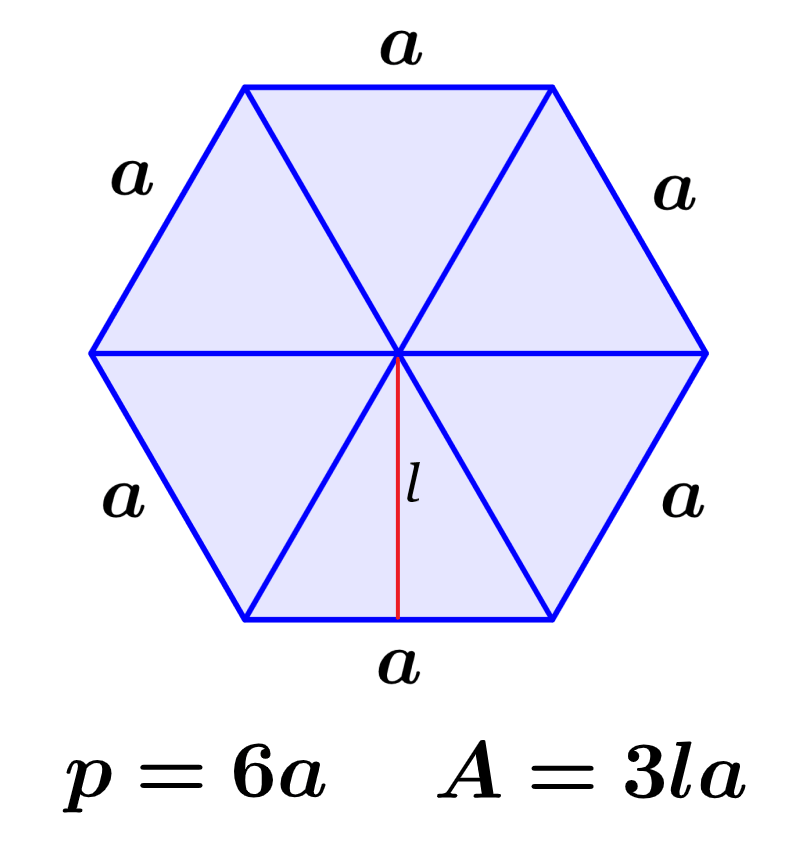
The perimeter of a hexagon is the total length of its outline. On the other hand, the area represents the two-dimensional space occupied by the figure. We can find the perimeter of a hexagon by adding the lengths of its six sides, and we can find its area by multiplying three by its apothem and by the length of one of its sides.
In this article, we will learn all about the perimeter and area of hexagons. We will explore its formulas and apply them to solve some practice problems.
How to find the perimeter of a hexagon?
We can calculate the perimeter of a hexagon by adding the lengths of its six sides. Therefore, we can use the following formula:
$latex p=a+b+c+d+e+f$
where, $latex a.~b,~c,~d,~e,~f$ are the six lengths of the sides of the hexagon.
If we have a regular hexagon, we know that all six sides have the same length, so the formula for the perimeter is:
| $latex p=6a$ |
where, a is the length of one of the sides of the regular hexagon.
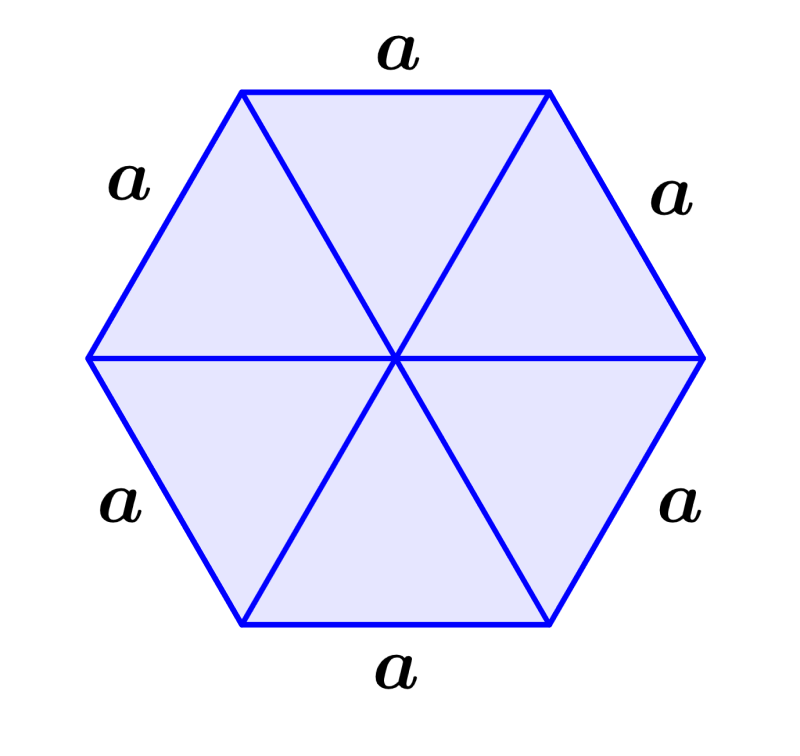
How to find the area of a hexagon?
We can calculate the area of a regular hexagon using the length of one of its sides and the length of its apothem. Then, we can use the following formula:
| $latex A=3sa$ |
where, s is the length of one of the sides of the hexagon and a is the length of the apothem. Remember that the apothem is the segment that connects the center of the hexagon with one of its sides.
Proof of the formula for the area of a hexagon
We can prove the formula for the area of a hexagon using the following diagram, where we divide the hexagon into six congruent triangles.
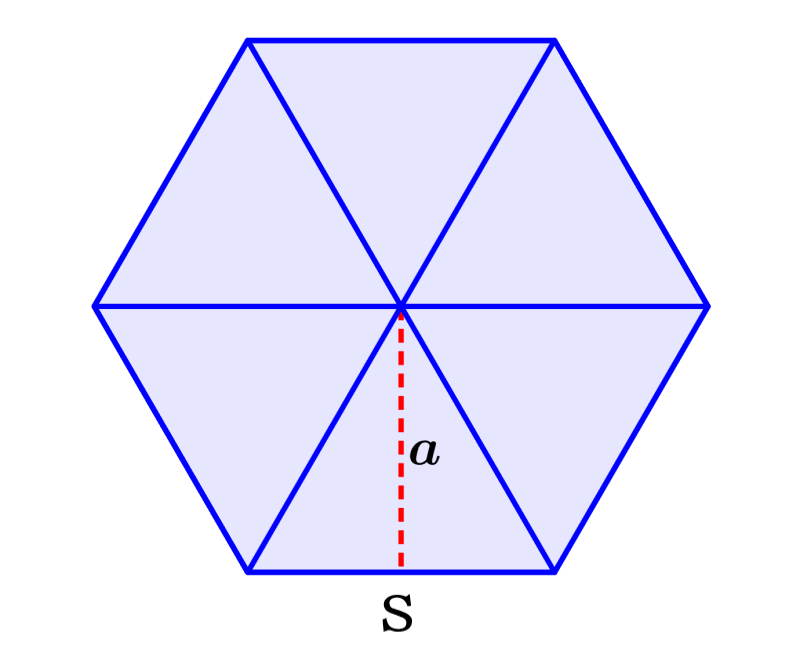
Now, we know that the area of any triangle can be calculated using the formula $latex A=\frac{1}{2}bh$, where b is the length of the base and h is the length of the height.
In this diagram, the base of each triangle is equal to one side of the hexagon and the height is equal to the apothem, so the area of each triangle is equal to $latex A=\frac{1}{2}sa$ .
Finally, we see that we have 6 equilateral triangles in the hexagon, so we multiply the area obtained by 6 to obtain $latex A=3sa$, which is the area of the hexagon.
Find the area of the hexagon without using the apothem
We can obtain a formula to find the area of a hexagon without using the length of the apothem. To accomplish this, we need to find an expression for the length of the apothem in terms of the length of one of the sides of the hexagon.
Noting that the triangles we traced in the hexagon are equilateral, we can use the Height of an Equilateral Triangle Formulas: $latex h=\frac{\sqrt{3}}{2}s$, where s is the length of one of the sides of the hexagon.
Therefore, replacing this value with the value of a, we have:
| $latex A=3s(\frac{\sqrt{3}}{2}s)$ $latex A=\frac{3\sqrt{3}}{2}{{s}^2}$ |
Area and perimeter of a hexagon – Examples with answers
EXAMPLE 1
Find the perimeter of a regular hexagon that has sides with a length of 5 inches.
Solution
Using the formula for the perimeter with the length $latex a=5$, we have:
$latex p=6a$
$latex p=6(5)$
$latex p=30$
The perimeter of the hexagon is equal to 30 in.
EXAMPLE 2
Find the area of a regular hexagon that has sides with a length of 4 feet and an apothem with a length of 3.46 ft.
Solution
We have the following lengths:
- Length, $latex s=4$ ft
- Apothem, $latex a=3.46$ ft
We can use the formula for the area with the given lengths:
$latex A=3sa$
$latex A=3(4)(3.46)$
$latex A=41.52$
The area of the hexagon is equal to 41.52 ft².
EXAMPLE 3
Find the perimeter of a regular hexagon that has sides with a length of 6 yards.
Solution
We use the length $latex a=6$ in the formula for the perimeter. Therefore, we have:
$latex p=6a$
$latex p=6(6)$
$latex p=36$
The perimeter of the hexagon is equal to 36 yd.
EXAMPLE 4
What is the area of a regular hexagon that has sides with a length of 6 inches and an apothem with a length of 5.2 inches?
Solution
We have the following information:
- Sides, $latex s=6$ in
- Apothem, $latex a=5.2$ in
Using the formula for the area with these lengths, we have:
$latex A=3sa$
$latex A=3(6)(5.2)$
$latex A=93.6$
The area of the hexagon is equal to 93.6 in².
EXAMPLE 5
What is the perimeter of a regular hexagon that has sides with a length of 13 inches?
Solution
Using the formula for the perimeter with the length $latex a=13$, we have:
$latex p=6a$
$latex p=6(13)$
$latex p=78$
The perimeter of the hexagon is equal to 78 in.
EXAMPLE 6
Find the area of a regular hexagon that has sides with a length of 7 feet and an apothem with a length of 6.06 feet.
Solution
We have the following:
- Sides, $latex s=7$ ft
- Apothem, $latex a=6.06$ ft
Using this in the formula for the area, we have:
$latex A=3sa$
$latex A=3(7)(6.06)$
$latex A=127.26$
The area of the hexagon is equal to 127.26 ft².
EXAMPLE 7
What is the length of the sides of a regular hexagon that has a perimeter of 72 yards?
Solution
Here, we know the perimeter and we are going to find the length of the sides of the hexagon. Therefore, we use the formula for the perimeter with $latex p=72$ and solve for a:
$latex p=6a$
$latex 72=6a$
$latex a=12$
The length of the sides is equal to 12 yd.
EXAMPLE 8
Find the area of a regular hexagon that has sides with a length of 5 inches.
Solution
In this case, we only know the length of one side of the hexagon, so we can use the second formula for the area with the length $latex s=5$:
$latex A=\frac{3\sqrt{3}}{2}{{s}^2}$
$latex A=\frac{3\sqrt{3}}{2}{{(5)}^2}$
$latex A=\frac{3\sqrt{3}}{2}(25)$
$latex A=64.96$
The area of the hexagon is equal to 64.96 in².
EXAMPLE 9
Find the length of the sides of a regular hexagon that has a perimeter of 126 feet.
Solution
We can find the length of the sides of the hexagon using the formula for the perimeter with $latex p=126$ and solve for a:
$latex p=6a$
$latex 126=6a$
$latex a=21$
The length of the sides is equal to 21 ft.
EXAMPLE 10
What is the area of a pentagon that has sides with a length of 8 feet?
Solution
We use the second formula for the area , since we only have the length of one side of the hexagon. Therefore, we use the length $latex s=6$ in the formula:
$latex A=\frac{3\sqrt{3}}{2}{{s}^2}$
$latex A=\frac{3\sqrt{3}}{2}{{(8)}^2}$
$latex A=\frac{3\sqrt{3}}{2}(64)$
$latex A=166.28$
The area of the hexagon is equal to 166.28 ft².
Area and perimeter of a hexagon – Practice problems
See also
Interested in learning more about perimeters and areas of geometric figures? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
