Arccot Calculator (Inverse Cotangent)
cot-1() =
Degrees:
Radians:
π radians:
Graph of the inverse cotangent
The domain of x is all real numbers.
The range is -π/2<y<π/2, not including 0.
Use this calculator to get the result of the inverse cotangent of a value of x. The result will be displayed in degrees, radians, and π radians. All real values of x can be used.
Here’s more information on how to use the inverse cotangent calculator. Also, you can learn about the definition, graph, and important values of arc cotangent.
How to use the inverse cotangent calculator?
Step 1: Enter the value of x in the first input box. Any real value of x can be used.
Step 2: The right panel will display the result in degrees.
Step 3: The solution in radians and π radians will be displayed at the bottom.
Difference between results in degrees, radians, and π radians
The equivalence between degrees and radians can be found by recalling that a complete revolution has a total of 360° or 2π radians. Therefore, we can deduce that 180° is equal to π radians.
On the other hand, the difference between the result in π radians and radians, is that “radians” already has the value of π included. For example, recalling that the value of π is approximated at 3.1415…, the result 1.5 π radians is equal to 4.712 radians.
What is the inverse cotangent?
The inverse cotangent, also known as the arc cotangent, is the inverse function of the cotangent. This means that the inverse cotangent reverses the effects of the cotangent function.
Therefore, the output values of the cotangent function are equal to the input values of the inverse cotangent and vice versa. For example, the cotangent of 45° is equal to 1, while the inverse cotangent of 1 is equal to 45°.
The inverse cotangent function is denoted as cot-1(x) or also as arccot(x).
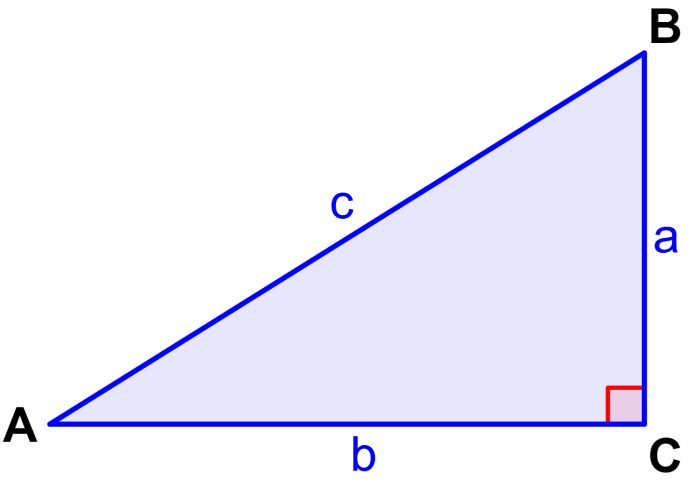
This function can be used to find the value of an angle if we know the proportions or the lengths of the sides of a right triangle. For example, angle A in the following triangle can be determined using arccot(x), where x equals b/a.
Graph of the inverse cotangent
The graph of the inverse cotangent is obtained by considering specific intervals for the range of the function. In this calculator, we only consider values of y that are between -π/2 to π/2.
Domain of the inverse cotangent
Using the graph of the inverse cotangent, we can easily see that the function can take any value of x. This means that the domain of the inverse cotangent is equal to all real numbers of x.
Range of the inverse cotangent
From the graph of the function, we can deduce that the y-values range from -π/2 to π/2, but do not include 0. Therefore, the range of the inverse cotangent is -π/2 ≤ y ≤ π/2, excluding 0.
Table of the inverse cotangent of common values
| Value of x | arccot(x)(°) | arccot(x)(rad) |
| 0 | 90° | |
| 60° | ||
| 1 | 45° | |
| 30° | ||
| -1 | -45° | – |
Related calculators:
- Arccos Calculator (Inverse Cosine) – Degrees and Radians
- Arcsin Calculator (Inverse Sine) – Degrees and Radians
- Arctan Calculator (Inverse Tangent) – Degrees and Radians
- Arcsec Calculator (Inverse Secant) – Degrees and Radians
- Arccsc Calculator (Inverse Cosecant) – Degrees and Radians
You can explore other calculators here.
