The domain can be calculated by finding the set of all possible values for the independent variable, usually x. The range can be calculated by finding the set of all possible values for the dependent variable, generally y.
Here, we will look at a summary of the domain and range of a function. We will also review how to find the domain and range. In addition, we will solve several domain and range exercises to learn the reasoning used when solving these types of exercises.
Summary of domain and range
Domain
The domain of a function is the set of all possible values of the independent variable. That is, the domain is the set of all values of x that will cause the function to produce real values of y.
To find the domain of a function, we remember the following:
- The denominator of a fraction cannot be equal to zero.
- The number under a square root sign cannot be negative.
Therefore, the domain of a function is found by looking for the values of the independent variable (usually x) that we can use. We have to avoid 0 in the denominator of a fraction and negative numbers in square roots.
Range
The range of a function is the set of all possible values of the dependent variable (usually y) after using the domain. This means that the range is the set of the values of y that we get after using all the values of x.
To find the range of a function, we consider the following:
- The range is the extent of the y values, from the minimum value to the maximum value.
- We can substitute values of x to analyze the result, considering whether the values are negative or positive.
- We have to make sure we are looking for maximum and minimum values.
Domain and range – Examples with answers
The following domain and range examples have their respective solution. Each solution details the process and reasoning used to obtain the answer.
EXAMPLE 1
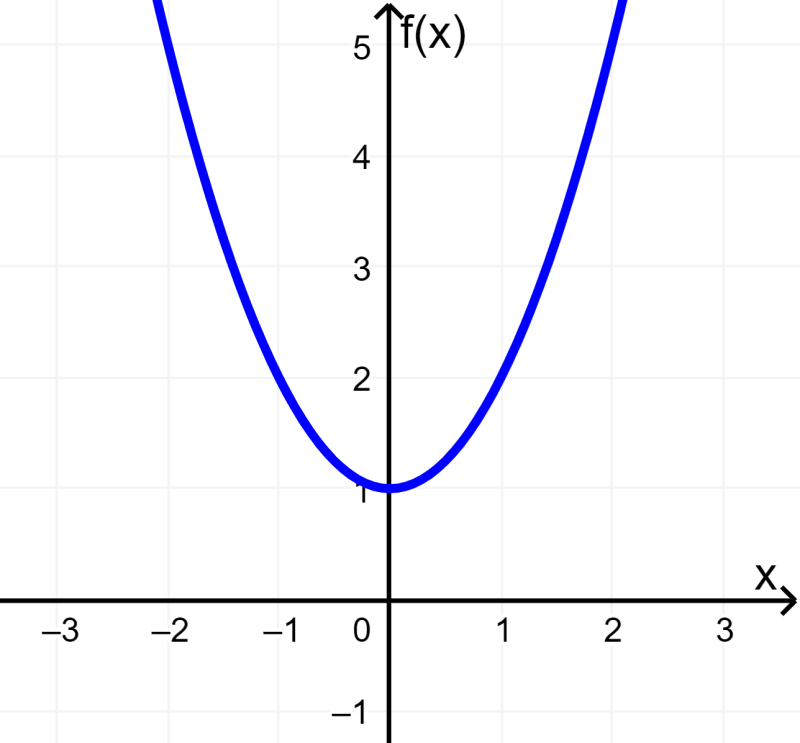
Find the domain and the range of the function $latex f(x)={{x}^2}+1$.
Solution
Domain: The function $latex f(x)={{x}^2}+1$ is defined for all real values of x because there are no restrictions of the values of x. Therefore, the domain of x is:
“All real values of x“
Range: Given that $latex {{x}^2}$ is never negative, $latex {{x}^2}+1$ is never less than 1. Therefore, the range of $latex f(x)$ is:
“All real values of $latex f(x) \geq 1$”
We can see that x can take any value in the graph, but the values of $latex y=f(x)$ are greater than or equal to 1.
EXAMPLE 2
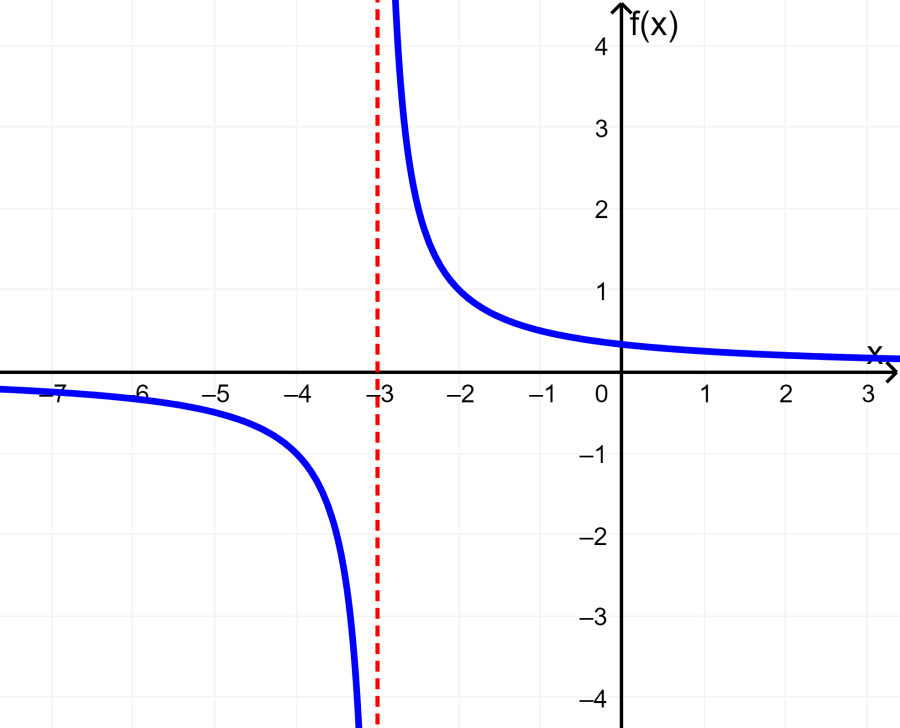
Find the domain and the range of the function $latex f(x)= \frac{1}{x+3}$.
Solution
Domain: The function $latex f(x)= \frac{1}{x+3}$ is not defined for $latex x=-3$ since this would produce a division by zero (we would have a 0 in the denominator). Therefore, the domain of $latex f(x)$ is:
“All real numbers except the -3”
Range: No matter how big or small x is, the values of $latex f(x)$ will never equal zero. If we try to solve the equation for 0, we have:
$latex 0= \frac{1}{x+3}$
Multiplying both sides by $latex x + 3$, we have:
$latex 0= 1$
This is impossible. Therefore, the range of $latex f(x)$ is:
“All real numbers except zero”
In the graph, we can see that the function is not defined for $latex x = -3$ and that the function takes all y values except zero:
EXAMPLE 3
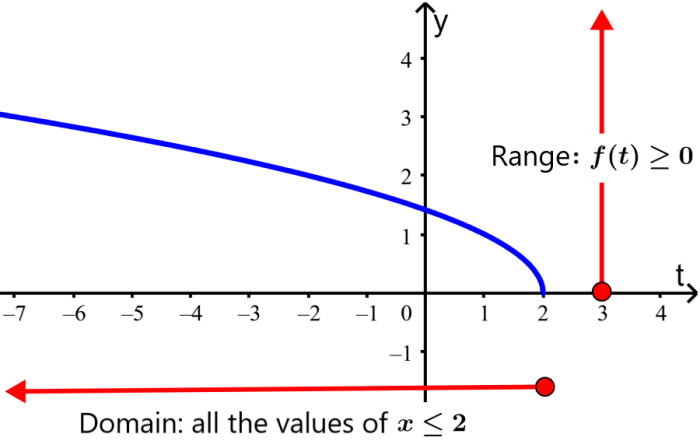
Find the domain and the range of the function $latex f(t)=\sqrt{5-t}$.
Solution
Domain: The function $latex f(t)=\sqrt{5-t}$ is not defined for real numbers greater than 5 since this would result in negative numbers below the square root and imaginary numbers for $latex f(t)$, so the domain of $latex f(t)$ is:
“All real numbers, $latex t\leq 5$”
Range: By definition of a square root we have:
$latex f(t)=\sqrt{5-t}\geq 0$
Therefore, the range of $latex f(t)$ is:
“All real numbers, $latex f(t)\geq 0$”
In the graph, we can see that $latex t$ does not take values greater than 5 and that the range is greater than or equal to 0:
EXAMPLE 4
Determine the domain and the range of the function $latex f(x)=\frac{{{x}^2}+x-2}{{{x}^2}-x-2}$.
Solution
Domain: The only problem we have with this function is that we have to be careful not to divide by zero. Then the values of x cannot take those values that produce a division by zero. Therefore, we make the denominator equal to zero and solve:
$latex {{x}^2}-x-2=0$
$latex (x+1)(x-2)=0$
$latex x=-1$ o $latex x=2$
Thus, the domain is:
“All real numbers except -1 and 2”
Range: The range is a bit more difficult to determine, but in this case, we have no visible constraints that make the range greater or less than a specific value. We check this with the graph of the function:
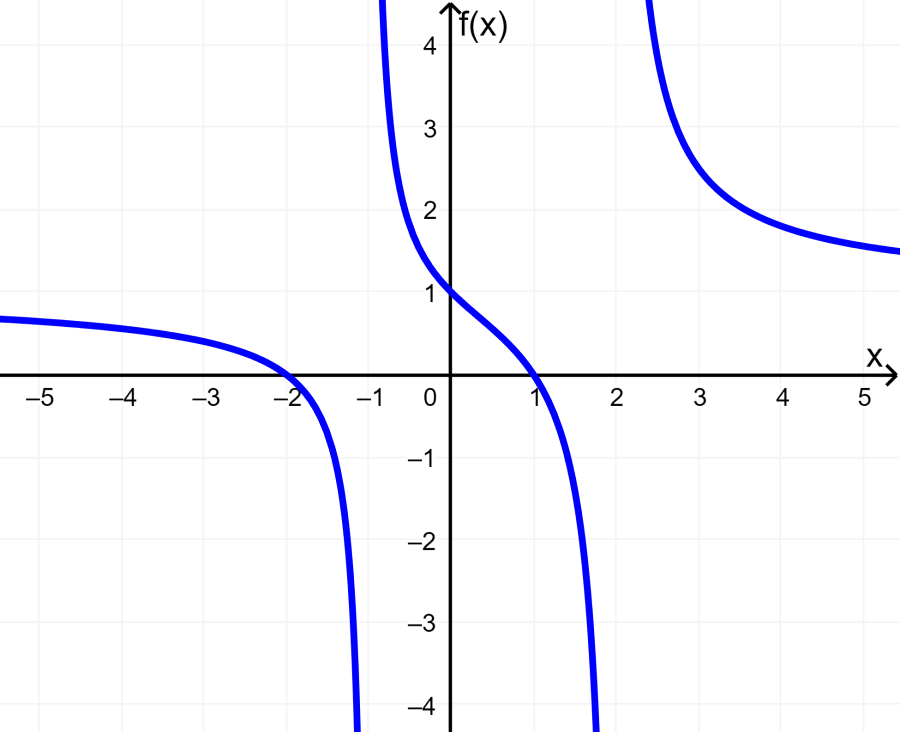
We see that the function takes all the values of y, so the range is:
“All real numbers”
EXAMPLE 5
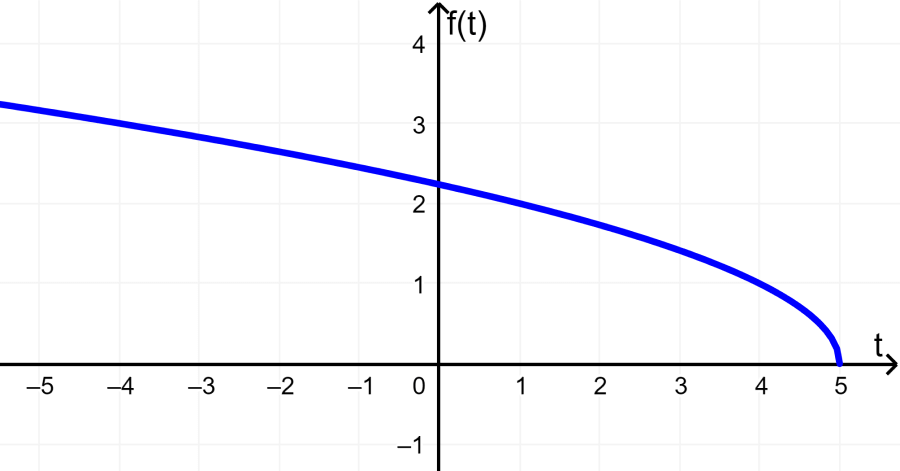
Determine the domain and the range of the function $latex g(x)=-\sqrt{-2x+5}$.
Solution
Domain: The only problem we have with this function is that we cannot have negative values within the square root sign. Therefore, we can make the expression inside the square root greater than or equal to zero and solve:
$latex -2x+5\geq 0$
$latex -2x\geq -5$
$latex 2x\leq 5$
$latex x\leq \frac{5}{2}$
Therefore, the domain is:
“All real numbers $latex x\leq \frac{5}{2}$”
Range: We know that by definition, the result of a square root must be greater than or equal to zero. However, in this case, we have a negative sign that precedes the square root, so the range is:
“All real numbers $latex g(x)\leq 0$”
We can look at the graph to check this:
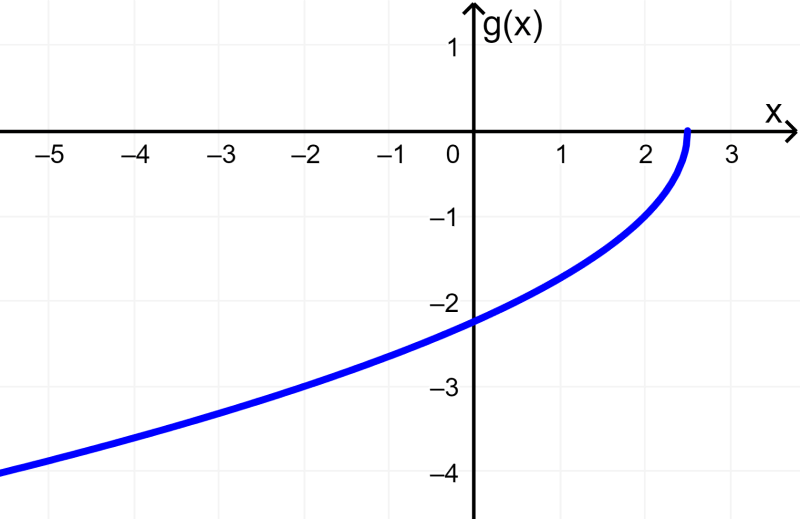
EXAMPLE 6
Find the domain and the range of the function $latex f(x)=-{{x}^4}+2$.
Solution
Domain: This is an easy problem. There are no denominators, so we don’t have division by zero problems and there are no radicals, so we don’t have problems with negative numbers in square roots. When we have a polynomial like in this case, the domain is:
“All real numbers”
Range: The range varies from polynomial to polynomial. In this case, we know that $latex {{x}^4} $ is always positive, so when preceded by a negative sign, we always obtain a negative number or equal to 0. That means that the highest point is 2 and the range is:
All real numbers, $latex f(x)\leq 2$”
In the graph, we can see this:
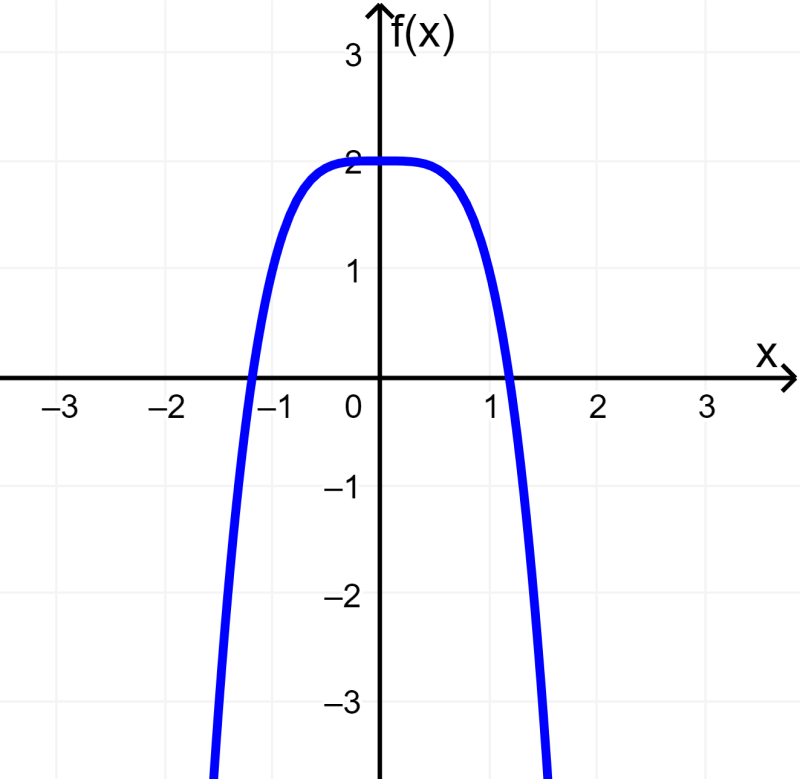
See also
Interested in learning more about functions? Take a look at these pages: