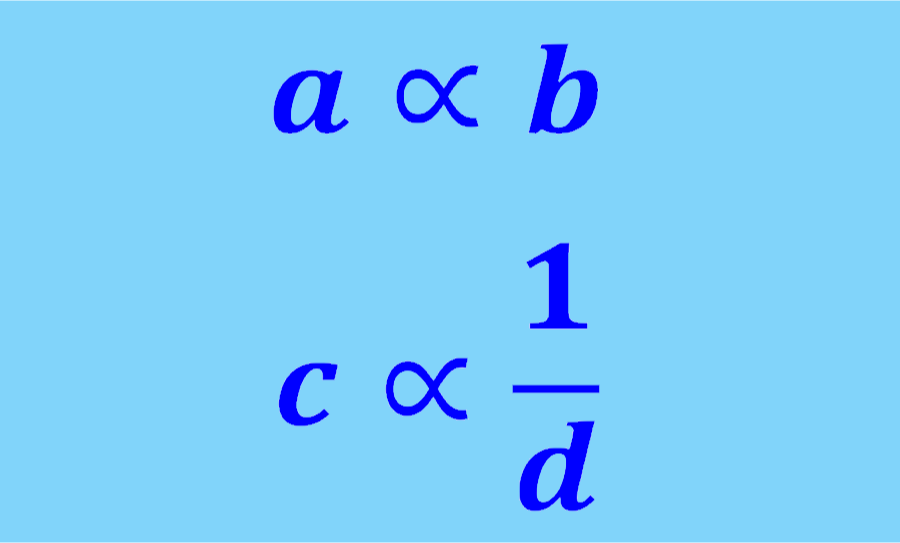Direct and inverse proportionality allows us to compare two quantities and understand how they are related. Direct proportionality refers to the case when increasing one value, produces an increase in another value. Inverse proportionality refers to the case when increasing one value, produces a decrease in another value.
In this article, we will look at direct proportionality and inverse proportionality in detail. Also, we will learn about the rule of 3, which allows us to solve proportionality problems easily.
Definitions of direct and inverse proportionality
Direct and inverse proportionality is used to show how two quantities are related to each other. The symbol used to denote proportionality is “∝”. For example, if we say that a is proportional to b, this is represented by “a∝b“. If we say that a is inversely proportional to b, this is denoted as “a∝1 / b“.
These relationships are governed by the same proportionality rules. In both cases, the value of a changes when b changes or the value of b changes when a changes. The change in both values is equated with a constant of proportionality. We will look at examples later to fully understand this.
Direct proportionality
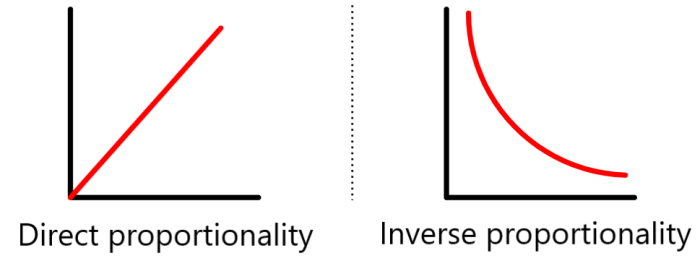
Two quantities a and b are directly proportional if they increase or decrease together, that is, the ratio of their corresponding values remains constant. This means that we have:
$latex \frac{a}{b}=k$
where k is a positive number, and the variables a and b are varying directly.
The symbol used to represent direct proportion is “∝”.
Suppose that a is directly proportional to b. This can be written as:
a∝b
EXAMPLE
Suppose we have $latex a = 3b$. This shows that a is proportional to b and the value of one variable can be found if we know the value of the other variable.
If we have that the value of b is 5, $latex b = 5$, then we have:
$latex a=3(5)=15$
Similarly, if we have that the value of a is 15, we can find the value of b:
$latex 15=3b$
$latex b=5$
Rule of 3 – Direct case
The rule of 3 is an operation that helps us solve direct and inverse proportionality problems quickly.
In order to use the rule of 3, we need three values: two that are proportional to each other and a third. With these values, we can find a fourth.
Let’s look at the case of direct proportions. We are going to place the three values a, b, c, and the unknown value x as follows and then we will apply the formula:
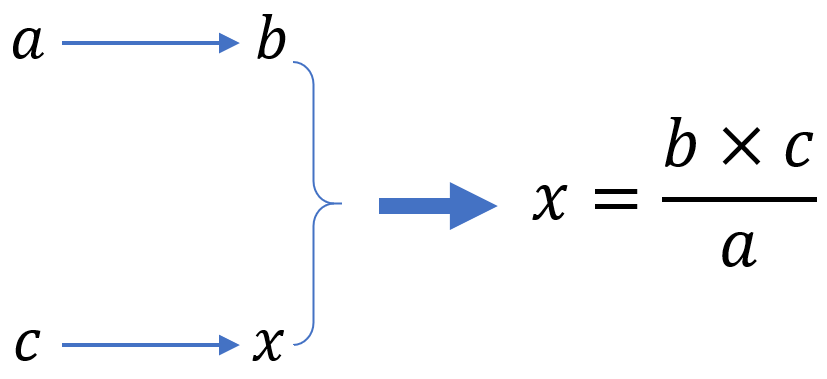
In this formula, a and b are directly proportional. Moreover, c and x have the same proportionality as a and b, but have different values.
EXAMPLE
Simon is a bricklayer and builds houses. He says it takes him 11 months to build 2 houses, and today he received an offer to build 5 houses. How many months would it take him to build the 5 houses?
Solution: Let’s form a diagram with the values we know and we will find the unknown value using the formula:
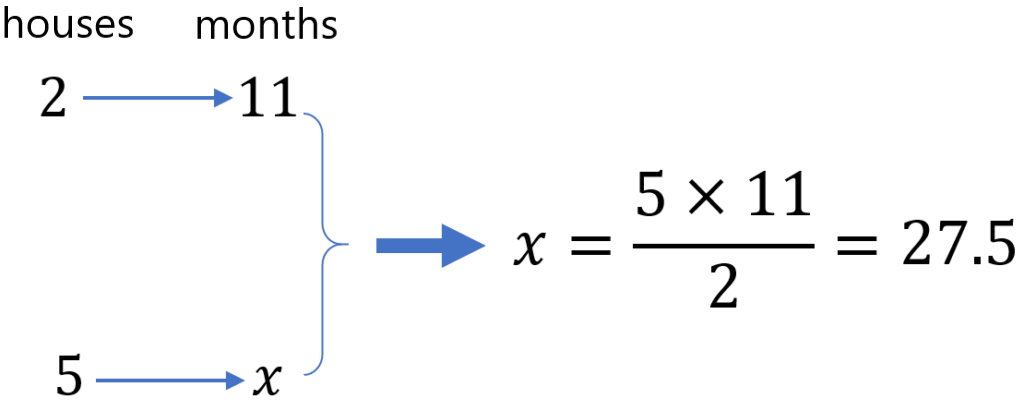
Therefore, it will take him 27.5 months to build 5 houses.
Try solving the following problem
Inverse proportionality
Two values are inversely proportional when one value increases while the other decreases. For example, a and b are inversely proportional if when a increases, b decreases, and vice versa.
This means that the product of their corresponding values must remain constant. That is, if $latex ab = k$, then a and b are inversely proportional.
The probability symbol is used in a different way. The following tells us that a is inversely proportional to b:
a∝$latex \frac{1}{b}$
EXAMPLE
Suppose we have $latex a=\frac{2}{b}$. This shows that a is inversely proportional to b and the value of one variable can be found if we know the value of the other variable.
If we have that the value of b is 4, $latex b = 4$, then we have:
$latex a=\frac{2}{4}=\frac{1}{2}$
Similarly, if we have that the value of a is 6, we can find the value of b:
$latex 6=\frac{1}{b}$
$latex b=\frac{1}{6}$
Rule of 3 – Inverse case
Now let’s see how to apply the rule of 3 in the case of inverse proportionality. We will place the 3 known values in the diagram similar to the previous case, but we will use a different formula:
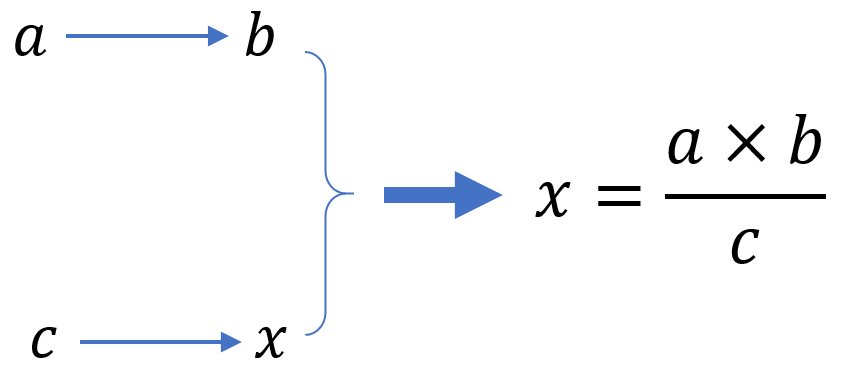
EXAMPLE
In a warehouse, truck drivers are transporting large quantities of rice. Using 4 trucks, it would take them 10 trips each to transport all the merchandise. If they use 5 trucks, how many trips would it take for each of them to transport that merchandise?
Solution: We are going to form a diagram with the values we know and use the formula to obtain the unknown value:
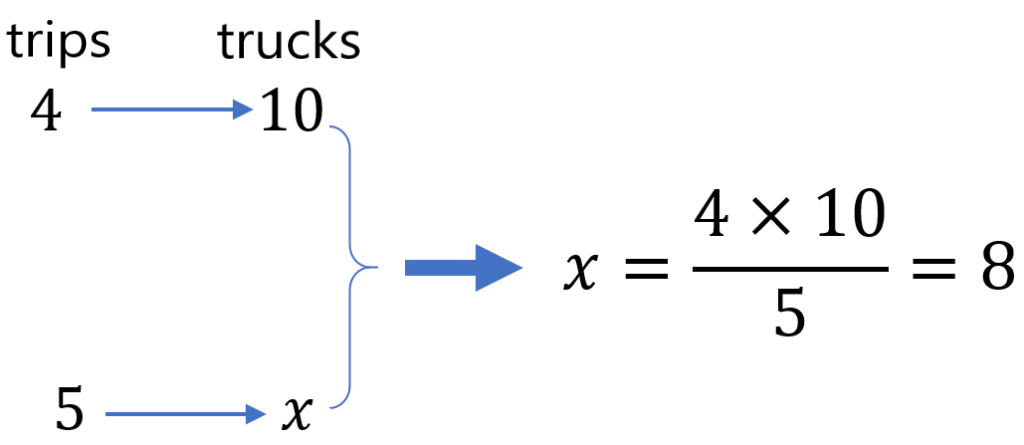
Therefore, using 5 trucks, it would take 8 trips each to transport all the merchandise.
Try solving the following problem
See also
Interested in learning more about proportionality? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
