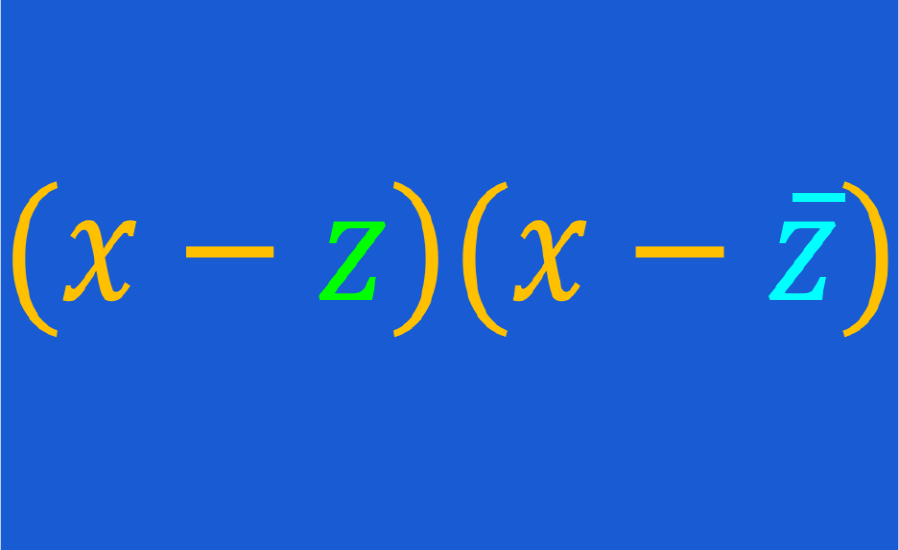The complex conjugate roots of a polynomial are those complex roots that are conjugate to each other. Remember that conjugates are two complex numbers that have the same real part and have the negative part with a different sign from each other.
Here, we will look at a review of conjugate complex numbers. Also, we will learn about the conjugate roots theorem of a polynomial. Finally, we will look at various examples of conjugate complex roots to look at the application of this theorem.
What are conjugates of complex numbers?
Complex conjugates are two complex numbers, so they have the form $latex a+bi$, where a and b are real numbers and $latex i=\sqrt{-1}$. The a is called the real part of a complex number and the bi is called the imaginary part.
Two complex numbers are conjugated to each other if they have the same real part and the imaginary parts are opposite of each other. This means that the conjugate of the number $latex a+bi$ is $latex a-bi$.
For example, if we have the complex number $latex 4+5i$, we know that its conjugate is $latex 4-5i$. Similarly, the complex conjugate of $latex 2-4i$ is $latex 2+4i$. Finding the conjugate of a complex number is very easy, we simply change the sign of the imaginary part of the number.
What are complex conjugate roots?
Complex conjugate roots can be described using the conjugate roots theorem:
Conjugate roots theorem
If the complex number $latex a+bi$ is a root of the polynomial $latex P(x)$ in a variable with real coefficients, then the complex conjugate $latex a-bi$ is also a root of that polynomial.
This theorem is very useful for finding the roots of polynomials. For example, suppose we are trying to find all the roots of a polynomial and as we solve, we find that $latex a+bi$ is a root of the polynomial.
Knowing this, we automatically know yet another root. By the conjugate roots theorem, we know that if $latex a+bi$ is a root, then $latex a-bi$ must be a root.
For example, if we find that $latex 6-3i$ is a root of a polynomial, then $latex 6+3i$ is also a root of that polynomial. This theorem saves us time and effort by not having to use an additional process to find that root.
Complex conjugate roots – Examples with answers
The complex conjugate roots theorem is used to solve the following examples. Each example has its respective solution, but it is recommended that you try to solve the exercises yourself before looking at the answer.
EXAMPLE 1
If $latex z=2+3i$ is a root of $latex p(z)={{z}^2}-4z+13$, show that $latex {{z}^{\ast}}=2-3i$ is another root.
Solution
We know that, if z is a root of a polynomial, we must have $latex p(z)=0$. Therefore, we can use $latex z=2-3i$ in the polynomial and equate with zero to check if it is a root:
$$p(2-3i)={{(2-3i)}^2}-4(2-3i)+13$$
$latex =4-12i+9{{i}^2}-8+12i+13$
$latex =9+9(-1)$
$latex =0$
We got zero after replacing $latex z=2-3i$ in the polynomial. This means that it is a root.
EXAMPLE 2
What are the roots of $latex 4{{x}^2}+10= -90$?
Solution
This equation can be solved directly by solving for the variable on one side of the equation.
We subtract 10 from both sides and then divide by 4 to solve for $latex {{x}^2}$:
$latex 4{{x}^2}+10=-90$
$latex 4{{x}^2}=-100$
$latex {{x}^2}=-25$
Now, we can take the square root of both sides of the equation. We use the sign $latex \pm$ since we have a positive root and a negative one:
$latex x=\pm\sqrt{-25}$
We know that we can rewrite $latex \sqrt{-a}$ as $latex i \sqrt{a}$. Therefore, we have:
$latex x=\pm i\sqrt{25}$
$latex =\pm 5i$
Therefore, the roots of the polynomial are the conjugates $latex x=5i$ and $latex x=-5i$.
EXAMPLE 3
What are the roots of the quadratic polynomial $latex {{x}^2}-4x+8=0$?
Solution
In this case, we can use the quadratic formula to solve:
$latex x=\frac{{-b\pm \sqrt{{{{b}^{2}}-4ac}}}}{{2a}}$
Here, we have the values $latex a=1$, $latex b=-4$ and $latex c=8$. Therefore, replacing these values, we have:
$latex x=\frac{{-(-4)\pm \sqrt{{{{{( -4)}}^{2}}-4( 1 )(8)}}}}{{2( 1)}}$
$latex =\frac{{4\pm \sqrt{{16-32}}}}{2}$
$latex =\frac{{4\pm \sqrt{{-16}}}}{2}$
$latex =\frac{4}{2}\pm \frac{\sqrt{{-16}}}{2}$
$latex =2\pm \frac{\sqrt{{-16}}}{2}$
Again, we can use the fact that $latex \sqrt{-a}=i\sqrt{a}$ to rewrite to expression:
$latex x=2\pm \frac{\sqrt{{16}}}{2}i$
$latex =2\pm \frac{4}{2}i$
$latex =2\pm 2i$
We obtained the two conjugate solutions for the quadratic equation:
$latex x=2+2i$, $latex x=2-2i$
EXAMPLE 4
Solve the equation $latex 3{{x}^2} -4x+10 =0$ and find its roots.
Solution
Similar to the previous exercise, we can use the quadratic formula with the values $latex a=3$, $latex b=-4$ and $latex c=10$. Therefore, we have:
$latex x=\frac{{-(-4)\pm \sqrt{{{{{( -4)}}^{2}}-4( 3 )(10)}}}}{{2( 3)}}$
$latex =\frac{{4\pm \sqrt{{16-120}}}}{6}$
$latex =\frac{{4\pm \sqrt{{-104}}}}{6}$
$latex =\frac{{4\pm \sqrt{{-4}}\sqrt{{26}}}}{6}$
$latex =\frac{{4\pm 2i \sqrt{{26}}}}{6}$
$latex =\frac{{2\pm i \sqrt{{26}}}}{3}$
The conjugate solutions of the quadratic equation are:
$latex =\frac{{2+ i \sqrt{{26}}}}{3}$, $latex =\frac{{2- i \sqrt{{26}}}}{3}$
Complex conjugate roots – Practice problems
Put into practice what you have learned about complex conjugate roots to solve the following problems. If you need help with these problems, you can look at the solved examples above.
See also
Interested in learning more about the complex roots of polynomials? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
