A parabola is a conic section formed when a circular cone is cut by a plane. For the parabola to be formed, the plane that intersects the cone must be parallel to one lateral side of the cone. A parabola is defined by the set of all points (x, y) that are located at the same distance from a line, called the directrix, and a fixed point (the focus) that is not on the directrix.
Here, we will learn about these conic sections. Specifically, we will learn about the equation of the parabola when the vertex is located at the origin. We will solve some exercises to apply these concepts.
PRECALCULUS
Relevant for…
Finding the equation of a parabola with vertex at the origin.
PRECALCULUS
Relevant for…
Finding the equation of a parabola with vertex at the origin.
Parabolas with vertex at the origin
From algebra, we know that a parabola has the general equation $latex y={{x}^2}$. The graph of this parabola has the vertex at (0, 0) and an axis of symmetry at $latex x=0$.
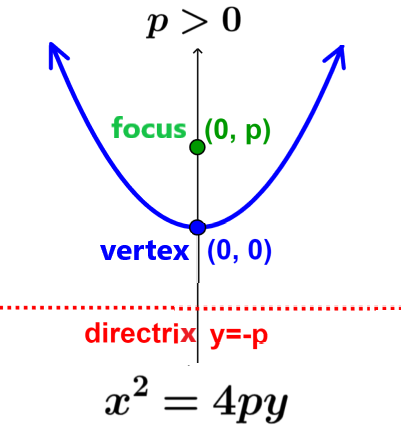
However, it is also possible to define a parabola in a different way since parabolas have the main property that each point in the parabola is equidistant from another point, called the focus, and a line called the directrix.
The focus of the parabola is located on its axis of symmetry and the vertex is located in the middle between the focus and the directrix. Also, the directrix is perpendicular to the axis of symmetry. We can observe this in the following diagram:
The most common equation to describe a parabola is in the form $latex y =a{{x}^2}$. We are going to rewrite this equation to obtain an equation of the form $latex {{x}^2}=4py$, where p is used to find the focus and directrix. Furthermore, we will also plot a parabola with horizontal orientation so that the equation will be $latex {{y}^2}=4px$.
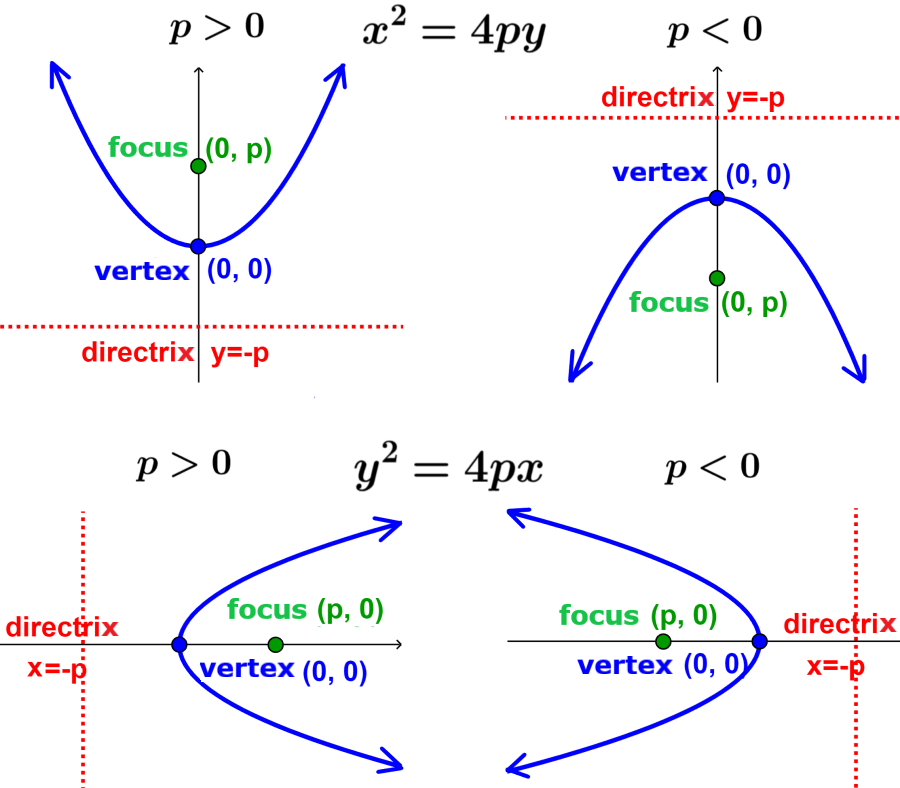
In the diagram, we can see that in all cases the vertex is (0, 0). When the parabola opens up or down, we see that x is squared. On the other hand, when the parabola opens to the left or to the right, the y is squared.
Also, when the value of p is positive, the focus is on the positive part of the axes and the parabola opens upwards or to the right. On the other hand, when the value of p is negative, the focus is located on the negative part of the axes and the parabola opens downwards or to the left.
Graphs of parabolas with vertex at the origin in standard form
Let’s find the focus and directrix of the parabola $latex {{y}^2}=-8x$. In addition, we are going to determine if the parabola opens up, down, left, or right. Here, we will graph the parabola.
To find the focus and the directrix, we have to start by finding p. We can form the equation $latex -8 = 4p$ and solve for p:
$latex -8=4p$
$latex p=-2$
We have y squared, so we know that the parabola opens to the left or to the right. Moreover, because p is negative, we know that the parabola must open to the negative side of the x-axis. Therefore, the focus is (-2, 0) and the directrix is $latex x = 2$.
To graph the parabola, we have to draw the focus and the directrix. Then we find at least two points on the parabola to get the curve correctly. In this case, because the point (-2, 4) is part of the parabola, the point (-2, -4) is also part of the parabola since it is symmetric. Therefore, we have:
Examples with answers of parabolas with vertex at the origin
The following exercises are solved by applying what has been learned about equations of parabolas in their standard form. These exercises can be used to reinforce what has been learned.
EXAMPLE 1
If the focus of the parabola is (0, 2), what is its equation?
Solution: The vertex of the parabola is (0, 0). This means that the value of p is the value of y and is positive, so the parabola will open up. Therefore, the general equation is $latex {{x}^2}=4py$.
If we substitute p by 2, we have:
$latex {{x}^2}=4(2)y$
$latex {{x}^2}=8y$
EXAMPLE 2
What is the focus and directrix of the parabola $latex y = \frac{1}{2} {{x}^2}$?
Solution: To find the focus and directrix, we have to solve for $latex {{x}^2}$ and then find p. Therefore, we have:
$latex y=\frac{1}{2}{{x}^2}$
$latex 2y={{x}^2}$
Now, we form the equation $latex 2 = 4p$ and solve for p:
$latex 2=4p$
$latex p=\frac{1}{2}$
Therefore, the focus is $latex (0, \frac{1}{2})$ and the directrix is $latex y=-\frac{1}{2}$.
EXAMPLE 3
Find the equation of the parabola with directrix $latex x = -2$.
Solution: If the directrix is negative and vertical, we know that the equation must be $latex {{y}^2} = 4px$ and that the parabola must open to the right, so p is positive: $latex p = 2$. Therefore, the equation of the parabola is:
$latex {{y}^2}=4(2)x$
$latex {{y}^2}=8x$
See also
Interested in learning more about parabolas? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
