Hyperbolas are formed by the set of all points in the Cartesian plane so that the difference of the distances between any point and the foci is equal to a constant. Hyperbolas have two lines of symmetry. The transversal axis is defined as the segment that joins the two vertices and passes through the center. The conjugate axis is the segment that connects the two covertices and is perpendicular to the transversal axis. The foci are located on the line that contains the transverse axis. The center of the hyperbola is located at the point of intersection of the transverse axis and the conjugate axis. The two asymptotes of the hyperbola also intersect at the center.
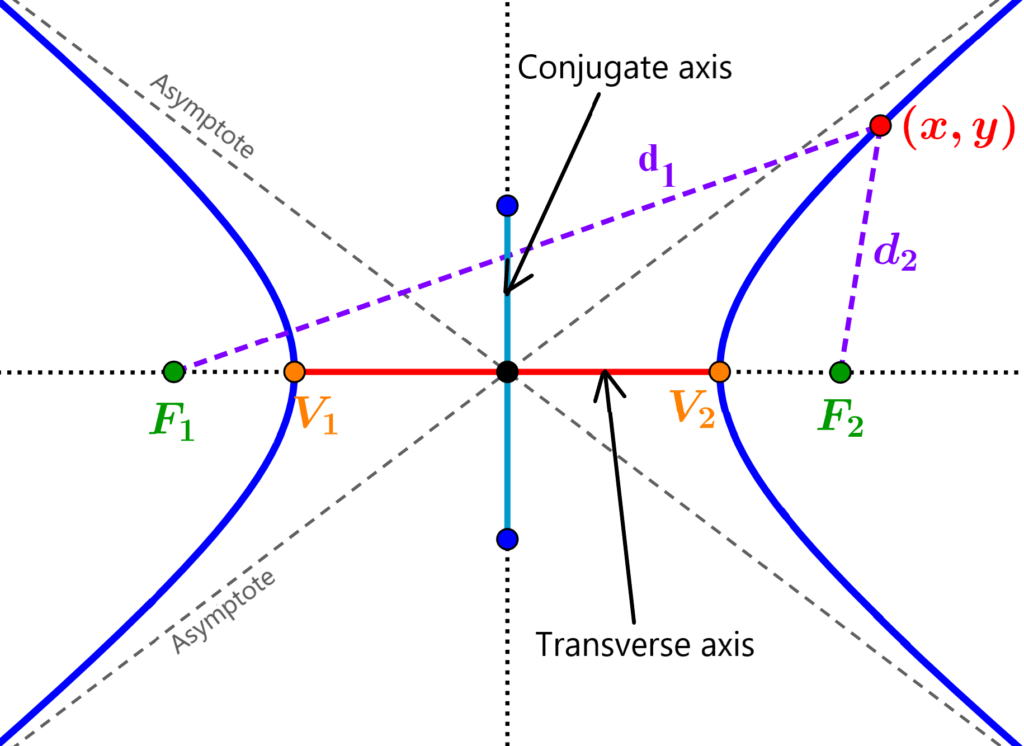
There are four variations of the equation of a hyperbola. First of all, we have two variations depending on the location of the center. The center can be located at the origin or outside the origin. Also, we have two variations depending on whether the hyperbola is oriented horizontally or vertically.
Equation of hyperbolas with center at the origin
The equation of a hyperbola that has the center at the origin has two variations that depend on its orientation. When the transverse axis (segment connecting the vertices) of the hyperbola is located on the x-axis, the hyperbola is oriented horizontally.
When the transverse axis is located on the y axis, the hyperbola is oriented vertically.
Horizontally oriented hyperbola
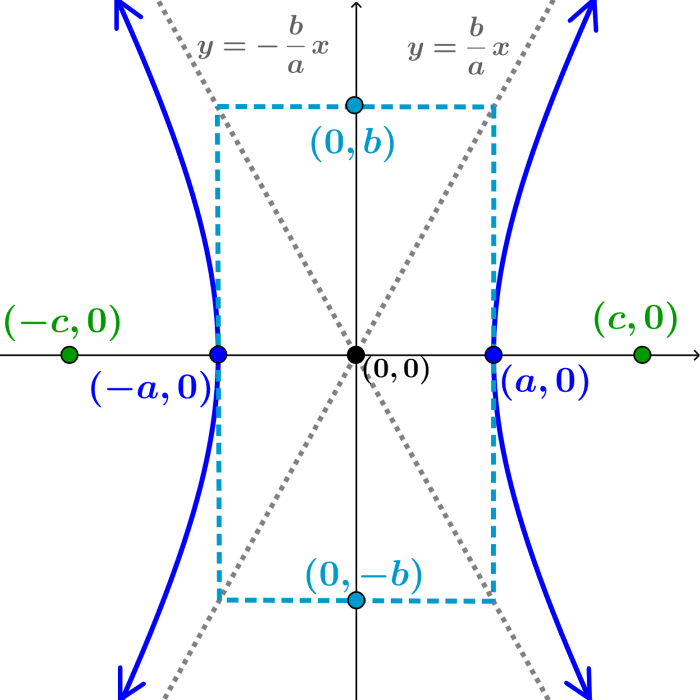
When the hyperbola is centered at the origin, (0, 0) and its transversal axis is on the x-axis, its equation in standard form is:
| $latex \frac{{{x}^2}}{{{a}^2}}-\frac{{{y}^2}}{{{b}^2}}=1$ |
where,
- The length of the transverse axis is $2a$
- The vertices have the coordinates $latex (\pm a, 0)$
- The conjugate axis (segment that joins the covertices) has a length of $latex 2b$
- The covertices have the coordinates $latex (0, \pm b)$
- The distance between the foci is $latex 2c$, where, $latex {{c}^2}={{a}^2}+{{b}^2}$
- The foci have the coordinates $latex (\pm c, 0)$
- The asymptotes have the equations $latex y=\pm \frac{b}{a}x$
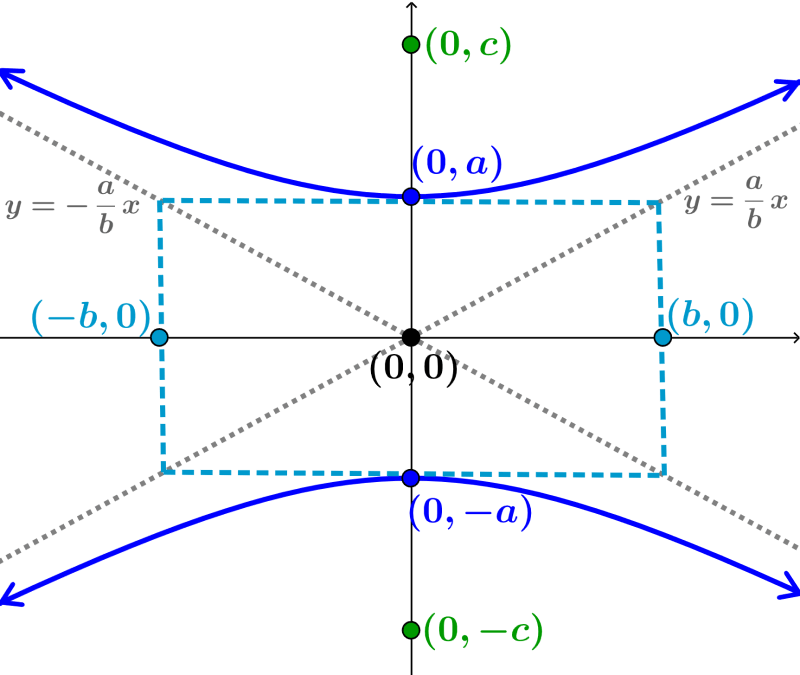
Vertically oriented hyperbola
A hyperbola that is centered at the origin, (0, 0), and that has its transversal axis on the y axis, has the general equation:
| $latex \frac{{{y}^2}}{{{a}^2}}-\frac{{{x}^2}}{{{b}^2}}=1$ |
where,
- $2a$ represents the length of the transverse axis
- The vertices are located at $latex (0, \pm a)$
- $latex 2b$ represents the length of the conjugate axis
- The covertices are located at $latex (\pm b, 0)$
- $latex 2c$ represents the distance between the foci, where, $latex {{c}^2}={{a}^2}+{{b}^2}$
- The foci are located in $latex (0, \pm c)$
- The equations of the asymptotes are $latex y=\pm \frac{a}{b}x$
Use vertices and foci to find equations of hyperbolas centered at the origin
When a hyperbola is centered at the origin, we can use the coordinates of the vertices and foci along with the following steps to find its equation:
Step 1: Determine the location of the transversal axis with respect to the x-axis or on the y axis to find its orientation.
1.1. A hyperbola is oriented horizontally when the coordinates of the vertices have the form $latex (\pm a, 0)$ and the coordinates of the foci have the form $latex (\pm c, 0)$. In these cases, we use the form $latex \frac{{{x}^2}}{{{a}^2}}-\frac{{{y}^2}}{{{b}^2}}=1$.
1.2. A hyperbola is oriented vertically when the coordinates of the vertices have the form $latex (0, \pm a)$ and the coordinates of the foci have the form $latex (0, \pm c)$. In these cases, we have the form $latex \frac{{{y}^2}}{{{a}^2}}-\frac{{{x}^2}}{{{b}^2}}=1$.
Step 2: We find the value of $latex {{b}^2}$, using the equation $latex {{b}^2}={{c}^2}-{{a}^2}$.
Step 3: We substitute the values of $latex {{a}^2}$ and $latex {{b}^2}$ into the general form from step 1.
EXAMPLE
If it is a hyperbola that has vertices at (±5, 0) and foci at (± 6, 0), what is its equation?
Solution
Looking at the coordinates of the foci and the vertices, we conclude that they are located on the x-axis. Therefore, we know that the transverse axis is on the x-axis and the equation will have the following form:
$latex \frac{{{x}^2}}{{{a}^2}}-\frac{{{y}^2}}{{{b}^2}}=1$
Using the vertices $latex (\pm 5, 0 )$, we get the value $latex a=5$ and we have $latex {{a}^2}=25$.
Using the foci $latex (\pm 6,0)$, we get the value $latex c=6$ and we have $latex {{c}^2}=36$.
Using the equation $latex {{b}^2}={{c}^2}-{{a}^2}$, we find the value of $latex {{b}^2}$:
$latex {{b}^2}={{c}^2}-{{a}^2}$
$latex {{b}^2}=36-25$
$latex {{b}^2}=11$
Using the values we found, we define the equation of the hyperbola:
$latex \frac{{{x}^2}}{25}-\frac{{{y}^2}}{11}=1$
Equation of hyperbolas with center outside the origin
We can obtain the equation of hyperbolas centered outside the origin by applying a translation to the general equation. If we translate the hyperbola h units on the x-axis and k units on the y axis, the new center will be located at $latex (h, k)$.
In this case, the equation of the hyperbola also depends on its orientation.
Horizontally oriented hyperbola with center outside the origin
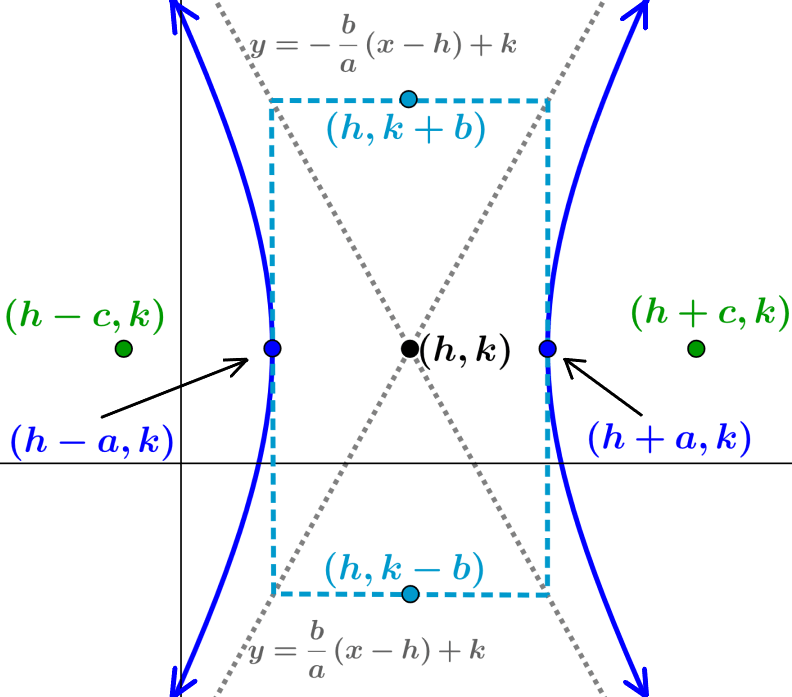
When the hyperbola has its center at the point $latex (h, k)$ and its transversal axis is parallel to the x axis, its equation is:
| $latex \frac{{{(x-h)}^2}}{{{a}^2}}-\frac{{{(y-k)}^2}}{{{b}^2}}=1$ |
where,
- h is the x component of the center and k is the y component of the center
- The transversal axis has a length of $latex 2a$
- The coordinates of the vertices are $latex (h\pm a, k)$
- The conjugate axis has a length of $latex 2b$
- The coordinates of the covertices are $latex (h, k\pm b)$
- The distance between the foci is $latex 2c$, where, $latex {{c}^2}={{a}^2}+{{b}^2}$
- The coordinates of the foci are $latex (h\pm c, 0)$
- The asymptotes have the equations $latex y=\pm \frac{b}{a}(x-h)+k$
Vertically oriented hyperbola with center outside the origin
If a hyperbola is centered at $latex (h, k)$ and its transverse axis is parallel to the y axis, its equation is:
| $latex \frac{{{(y-k)}^2}}{{{a}^2}}-\frac{{{(x-h)}^2}}{{{b}^2}}=1$ |
where,
- h is the x component of the center and k is the y component of the center
- The transversal axis measures $latex 2a$
- The vertices have the coordinates $latex (h, k\pm a)$
- The conjugate axis measures $latex 2b$
- The covertices have the coordinates $latex (h\pm b, k)$
- The distance between the foci is $latex 2c$, where, $latex {{c}^2}={{a}^2}+{{b}^2}$
- The coordinates of the foci are $latex (h, k\pm c)$
- The equations of the asymptotes are $latex y=\pm \frac{a}{b}(x-h)+k$
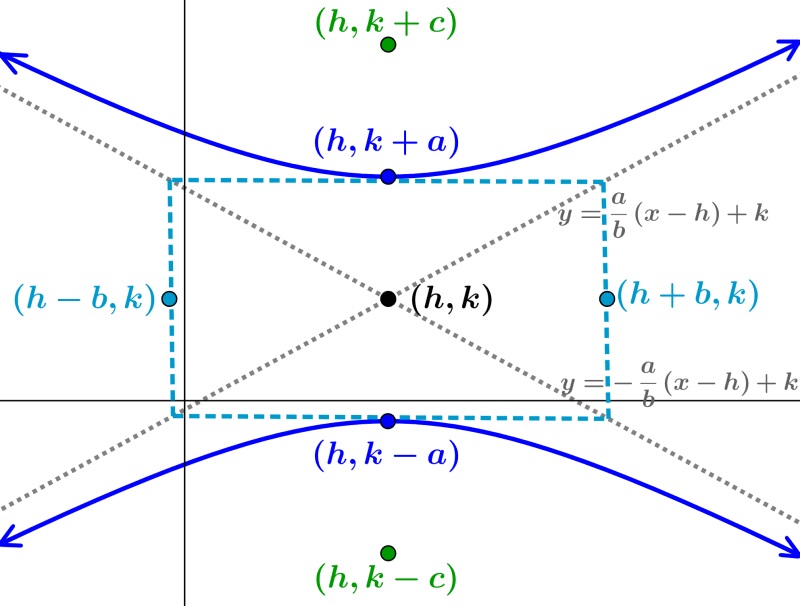
Use vertices and foci to find the equation for hyperbolas centered outside the origin
The equation of a hyperbola that is centered outside the origin can be found using the following steps:
Step 1: Determine if the transversal axis is parallel to the x-axis or parallel to the y axis to find the orientation of the hyperbola.
1.1. A hyperbola is horizontally oriented if the y coordinates of the vertices are the same as the y coordinates of the foci. In this case, we use the equation $latex \frac{{{(x-h)}^2}}{{{a}^2}}-\frac{{{(y-k)}^2}}{{{b}^2}}=1$.
1.2. A hyperbola is oriented vertically if the x coordinates of the vertices are the same as the x coordinates of the foci. In this case, we use the equation $latex \frac{{{(y-k)}^2}}{{{a}^2}}-\frac{{{(x-h)}^2}}{{{b}^2}}=1$.
Step 2: The center of the hyperbola, $latex (h, k)$, is found using the coordinates of the vertices and the midpoint formula.
Step 3: We find $latex {{a}^2}$ using the distance between the vertices, $latex 2a$.
Step 4: The value of c is found using the coordinates of the foci and the values of h and k.
Step 5: The value of $latex {{b}^2}$ is found using the equation $latex {{b}^2}={{c}^2}-{{a}^2}$.
Step 6: We use the values of $latex {{a}^2}$ and $latex {{b}^2}$ in the general equation from step 1.
EXAMPLE
What is the equation of a hyperbola that has foci at (2, 0), (2, 6) and vertices at (2, 1), (2, 5)?
Solution
We note that the x coordinates of the foci and the vertices are the same, so the transversal axis is parallel to the y axis. This means that the equation of the hyperbola has the form:
$latex \frac{{{(y-k)}^2}}{{{a}^2}}-\frac{{{(x-h)}^2}}{{{b}^2}}=1$
We find the center using the midpoint formula and the coordinates of the vertices (2, 1) and (2, 5):
$latex (h, k)=(\frac{2+2}{2}, \frac{1+5}{2})$
$latex =(2, 3)$
We find the value of $latex {{a}^2}$ using the length of the transverse axis, 2a. In turn, the length of the transverse axis is equal to the difference in the y coordinates of the vertices:
$latex 2a=|5-1|$
$latex 2a=4$
$latex a=2$
$latex {{a}^2}=4$
The value of $latex {{c}^2}$ is found using the coordinates of the foci, $latex (h, k \pm c)$. We can form the equations $latex (h, k-c)=(2, 0)$ and $latex (h, k+c)=(2, 6)$. Now, we use one of the equations to find the value of c:
$latex k+c=6$
$latex 3+c=6$
$latex c=3$
$latex {{c}^2}=9$
Using the equation $latex {{b}^2}={{c}^2}-{{a}^2}$ to find the value of $latex {{b}^2}$:
$latex {{b}^2}={{c}^2}-{{a}^2}$
$latex =9-4$
$latex =5$
Using the values of h, k, $latex {{a}^2}$ and $latex {{b}^2}$ into the general form, we have:
$latex \frac{{{(y-3)}^2}}{4}-\frac{{{(y-2)}^2}}{5}=1$
See also
Interested in learning more about equations of a hyperbola? Take a look at these pages:



