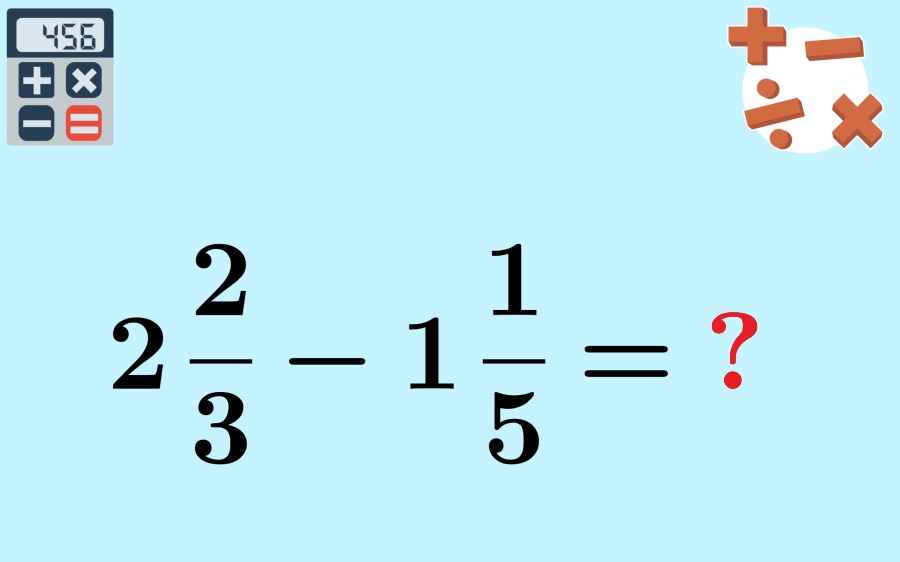Fractions with whole numbers are called mixed fractions. We can solve a subtraction of these types of fractions by converting them to improper fractions. For this, we multiply the whole number by the denominator and add the result to the numerator. Then we can use different processes depending on whether the fractions are like or unlike.
Here, we will learn how to subtract mixed fractions. We will look at the steps for like and unlike fractions. Then, we will solve some practice problems.
Steps to subtract mixed fractions
Unlike improper fractions, which only have a numerator and a denominator, mixed fractions also have whole numbers. These fractions can be like or unlike.
When the denominators of the fractions are the same, we have like fractions and when the denominators are different, we have unlike fractions.
We can solve a subtraction of mixed fractions with the following steps:
Step 1: Write the mixed fractions as improper fractions. To achieve this, we have to multiply the whole number by the denominator of the fraction and add the result to the numerator.
Step 2: Determine if the fractions are like (same denominators) or unlike (different denominators). If the fractions are like, continue to step 6.
Step 3: Find the least common denominator (LCD) of the fractions.
Step 4: Divide the LCD by the denominator of each fraction.
Step 5: Multiply the numerator and denominator by the numbers resulting from step 4. By doing this, we will obtain like fractions with a denominator equal to the LCD.
Step 6: Solve the difference of like fractions by using a single denominator and subtracting the numerators.
Step 7: Simplify the resulting fraction if possible.
Subtracting mixed fractions – Examples with answers
Each of the following examples has its respective solution using the mixed fraction subtraction solving steps seen above.
EXAMPLE 1
Solve the subtraction of fractions $latex 1\frac{1}{2}-\frac{1}{2}$.
Solution
Step 1: Converting the mixed fraction to an improper fraction, we have:
$$1\frac{1}{2}-\frac{1}{2}$$
$$=\frac{3}{2}-\frac{1}{2}$$
Step 2: We have like fractions because the denominators are the same. Therefore, we continue to step 6.
Steps 3-5: Not applicable.
Step 6: We subtract the like fractions by using a single denominator and subtracting the numerators:
$$=\frac{3}{2}-\frac{1}{2}$$
$$=\frac{3-1}{2}$$
$$=\frac{2}{2}$$
Step 7: Simplifying, we have:
$$=1$$
EXAMPLE 2
Solve the subtraction of mixed fractions $latex 2\frac{2}{3}-1\frac{1}{3}$.
Solution
Step 1: Converting both mixed fractions to improper fractions, we have:
$$2\frac{2}{3}-1\frac{1}{3}$$
$$=\frac{8}{3}-\frac{4}{3}$$
Step 2: The denominators are equal to 3, so the fractions are like. Thus, we continue to step 6.
Steps 3-5: Not applicable.
Step 6: We combine the denominators of the like fractions and subtract the numerators:
$$=\frac{8}{3}-\frac{4}{3}$$
$$=\frac{8-4}{3}$$
$$=\frac{4}{3}$$
Step 7: We can simplify by writing as a mixed fraction:
$$=1\frac{1}{3}$$
EXAMPLE 3
Solve the subtraction of mixed fractions $latex 1\frac{2}{3}-\frac{2}{5}$.
Solution
Step 1: We convert the first mixed fraction to an improper fraction:
$$1\frac{2}{3}-\frac{2}{5}$$
$$=\frac{5}{3}-\frac{2}{5}$$
Step 2: The fractions are unlike because the denominators are different. Thus, we continue to step 3.
Step 3: The denominators are 3 and 5, so the least common denominator is 15.
Step 4: Dividing 15 by 3 (first denominator), we get 5. Dividing 15 by 5 (second denominator), we get 3.
Step 5: We multiply the numerator and the denominator by the numbers obtained in step 4:
$$=\frac{5\times 5}{3\times 5}-\frac{2\times 3}{5\times 3}$$
$$=\frac{25}{15}-\frac{6}{15}$$
Step 6: We combine the denominators of the like fractions and subtract the numerators:
$$=\frac{25}{15}-\frac{6}{15}$$
$$=\frac{25-6}{15}$$
$$=\frac{19}{15}$$
Step 7: We can simplify by writing as a mixed number:
$$=1 \frac{4}{15}$$
EXAMPLE 4
Find the result of the subtraction $latex 2\frac{3}{4}-1\frac{1}{2}$.
Solution
Step 1: We convert both mixed fractions to improper fractions:
$$2\frac{3}{4}-1\frac{1}{2}$$
$$=\frac{11}{4}-\frac{3}{2}$$
Step 2: Since we have unlike fractions, we continue to step 3.
Step 3: We have denominators 4 and 2, so the least common denominator is 4.
Step 4: Dividing 4 by 4 (first denominator), we get 1. Dividing 4 by 2 (second denominator), we get 2.
Step 5: We multiply the numerator and the denominator by the numbers obtained in step 4:
$$=\frac{11\times 1}{4\times 1}-\frac{3\times 2}{2\times 2}$$
$$=\frac{11}{4}-\frac{6}{4}$$
Step 6: Combining the denominators and subtracting the numerators, we have:
$$=\frac{11-4}{4}$$
$$=\frac{5}{4}$$
Step 7: We can simplify by writing as a mixed number:
$$=1 \frac{1}{4}$$
EXAMPLE 5
Find the result of the following $latex 3\frac{2}{5}-\frac{3}{5}-1\frac{1}{5}$.
Solution
Step 1: We convert the two mixed fractions to improper fractions:
$$3\frac{2}{5}-\frac{3}{5}-1\frac{1}{5}$$
$$=\frac{17}{5}-\frac{3}{5}-\frac{6}{5}$$
Step 2: The fractions are like, so we continue to step 6.
Steps 3-5: Not applicable.
Step 6: Using a single denominator and subtracting the numerators, we have:
$$=\frac{17}{5}-\frac{3}{5}-\frac{6}{5}$$
$$=\frac{17-3-6}{5}$$
$$=\frac{8}{5}$$
Step 7: Converting to a mixed fraction, we have:
$$=1\frac{3}{5}$$
EXAMPLE 6
Find the result of $latex 3\frac{3}{4}-1\frac{2}{3}-1\frac{4}{5}$.
Solution
Step 1: We convert the three mixed fractions to improper fractions, and we have:
$$3\frac{3}{4}-1\frac{2}{3}-1\frac{4}{5}$$
$$=\frac{15}{4}-\frac{5}{3}-\frac{9}{5}$$
Step 2: We have three unlike fractions, so we continue to step 3.
Step 3: We have denominators 4, 3, and 5, so the least common denominator is 60.
Step 4: Dividing 60 by 4 (first denominator), we get 15. Dividing 60 by 3 (second denominator), we get 20. Dividing 60 by 5 (third denominator), we get 12.
Step 5: We multiply the numerators and denominators of the fractions by the numbers obtained in step 4:
$$=\frac{15\times 15}{4\times 15}-\frac{5\times 20}{3\times 20}-\frac{9\times 12}{5\times 12}$$
$$=\frac{225}{60}-\frac{100}{60}-\frac{108}{60}$$
Step 6: Subtracting the like fractions, we have:
$$=\frac{225-100-108}{60}$$
$$=\frac{17}{60}$$
Subtraction of mixed fractions – Practice problems
Use everything you have learned about subtracting mixed fractions to solve the following practice problems.
See also
Interested in learning more about subtracting fractions? You can take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
