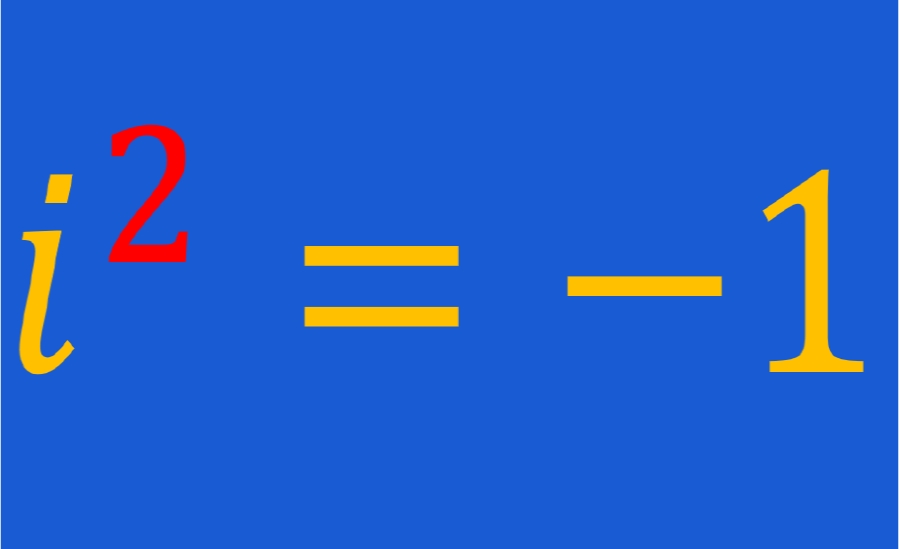
An imaginary number is a number that, when squared, has a negative result. In other words, imaginary numbers are defined as the square root of negative numbers and have no tangible value. These numbers cannot be quantized on the number line.
However, imaginary numbers are “real” in the sense that they do exist in mathematics and help us solve a large number of real-life problems.
Examples of imaginary numbers
Imaginary numbers are written using real numbers multiplied by the imaginary unit “i“. The notation “i” is the foundation of all imaginary numbers.
EXAMPLES
- 5i is an imaginary number, where 5 is the real number and “i” is the imaginary unit.
When we square this number, we get -25. This happens because the value of i² is -1. This means that $latex \sqrt{-1}=i$.
- -7i is an imaginary number, where -7 is the real number multiplied by the imaginary unit “1”.
If we square this number, we get -49 since the value of $latex {{(-7)}^2}$ is 49 and the value of i² is -1.
How to know if a number is real or imaginary?
Determining if a number is real or imaginary is very easy since generally, imaginary numbers always carry the imaginary unit “i“. Therefore, if a number is written with the imaginary unit, we know that it is an imaginary number.
For example, if we have the number 5, we know that it is a real number, but if we have the number 5i, we know that it is an imaginary number.
Similarly, if we have the number $latex -\frac{\sqrt{3}}{2}$, we know that it is a real number, and if we have the number $latex \frac{\sqrt{3}}{2}i$, we know that it is imaginary.
However, there may be situations where the imaginary numbers are not written with the imaginary unit. For example, if we have the number $latex \sqrt{-3}$, we know that this number is not real since in real numbers we cannot have square roots of negative numbers, so it is an imaginary number.
In short, we can identify imaginary numbers if they carry the imaginary unit. In case of not having the imaginary unit, we check if we have a square root (or another even root) of a negative number.
What is the origin of the imaginary numbers?
Complex numbers are known to have been used by mathematicians before they were properly defined, therefore, it is difficult to find their exact origin.
The first known reference to imaginary numbers is by Cardan in 1545. He mentioned them while investigating the roots of polynomials. During this period, the notation $latex \sqrt{-1}$ was used, but only in the sense of something convenient to categorize the properties of some polynomials. $latex \sqrt{-1}$ was considered a useful notation to put polynomials into categories, but it was not seen as a real mathematical object.
In 1777, Euler eliminated some of the complications by introducing the notation i and –i for the two square roots of -1. With Euler, the notation “i” for imaginary numbers originated. However, the numbers i and –i were called “imaginary” since their existence was not fully understood.
Wessel in 1797 and Gauss in 1799 employed a geometric interpretation of imaginary and complex numbers. This interpretation consisted in representing these numbers as points on a plane. With this, imaginary numbers became more concrete and less mysterious.
Finally, Hamilton in 1833 put complex numbers into mathematical definitions by showing that pairs of real numbers with properly defined multiplication form a number system, and the numbers that Euler defined as “i” are interpreted as one of this pair of numbers. This was the point at which the modern formulation of complex numbers began.
What is the use of imaginary numbers?
Imaginary numbers are widely used in real-life applications, such as electricity as well as quadratic equations. In quadratic planes, imaginary numbers appear in equations that do not touch the x-axis. Complex numbers are also extremely useful in advanced mathematical calculus.
Imaginary numbers have particular applications in electricity, especially in alternating current (AC) electronics. Alternating current electricity changes between positive and negative in a sine wave.
Combining alternating currents can be very difficult since they do not fit properly in the waves. Thanks to the use of imaginary numbers, the calculation of alternating currents is greatly simplified.
Imaginary numbers can also be used in signal processing, which is useful in cell phone and wireless technology, as well as radars and even brain waves. Essentially, imaginary numbers can be used in applications that use sine or cosine waves.
See also
Interested in learning more about imaginary and complex numbers? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
