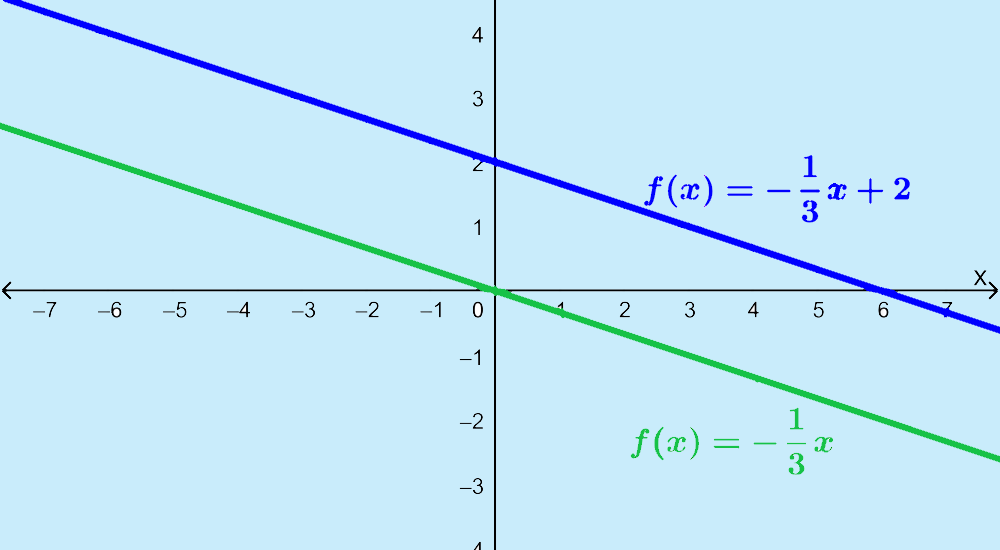
Linear equations are equations that produce graphs that are linear, that is, their graphs are straight lines. The main characteristic of these equations is that all of the variables have a maximum degree of one.
In this article, we will explore these equations in more detail. We will start by considering what linear equations are in general, and then we will look at the different forms in which they can be written. We will explore the slope-intercept form, the point-slope form, and the standard form.
Definition of linear equations
Linear equations are equations of the first order. These equations are defined by straight lines in the Cartesian plane. An equation for a straight line is called a linear equation. All the variables in linear equations have a maximum order of 1.
Example: the equation $latex y = x + 2$ is linear:
The following are examples of linear equations with 1 variable, with 2 variables, and with 3 variables:
EXAMPLES
Linear equations with one variable:
- $latex 2x+4=17$
- $latex 3x=24$
- $latex \frac{1}{2}x+2=10$
Linear equations with two variables:
- $latex x+3y=15$
- $latex y=3x-12$
- $latex \frac{1}{3}x+2y=5$
Linear equations with three variables:
- $latex x+2y-2z=10$
- $latex 2a+4b-c=8$
- $latex \frac{1}{2}x+2y=3z-4$
Forms of linear equations
There are varios different forms in which we can write linear equations, but generally, equations have constants (such as 2 or c) and must contain only simple variables (such as “x” or “y“).
EXAMPLES
These are linear equations:
- $latex y=2x-4$
- $latex y-5=2(x-1)$
- $latex y+3x-5=0$
- $latex 3x=24$
- $latex \frac{y}{3}=12$
However, keep in mind that linear equations should not contain variables (such as “x” or “y“) with exponents, square roots, cube roots, etc.
EXAMPLES
These are not linear equations:
- $latex {{y}^{2}}+3=9$
- $latex {{y}^{2}}+3=9$
- $latex \frac{{{{x}^{2}}}}{4}=20$
Slope-intercept form of a linear equation
The slope-intercept form is the most common in linear equations. This form is represented as follows:
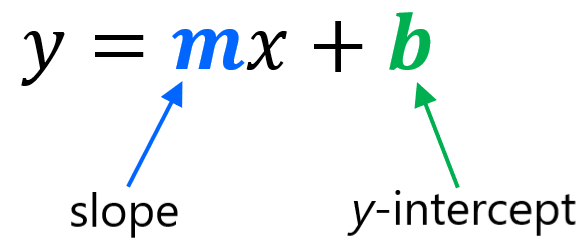
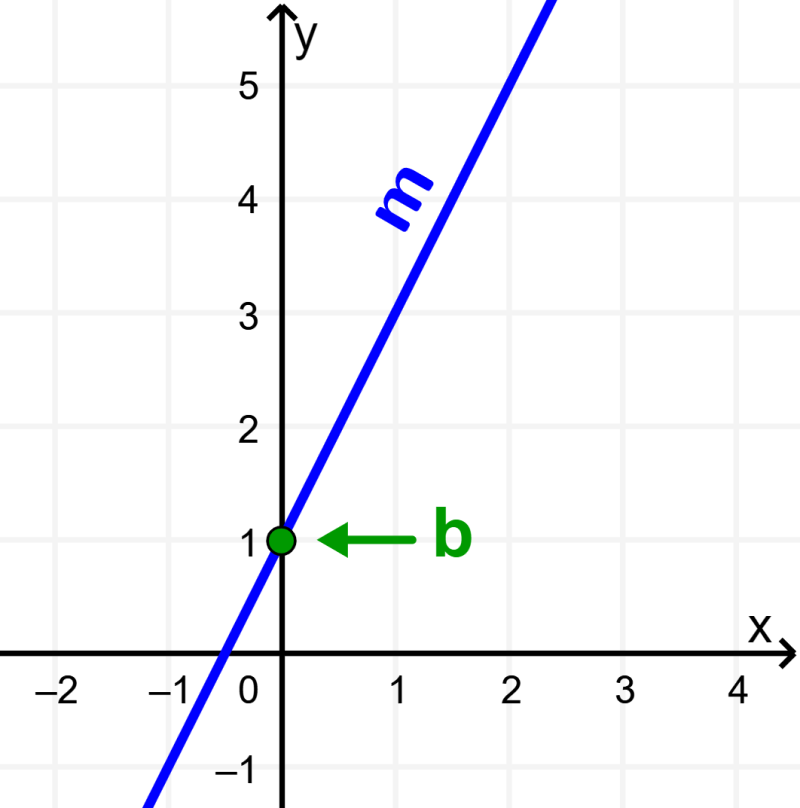
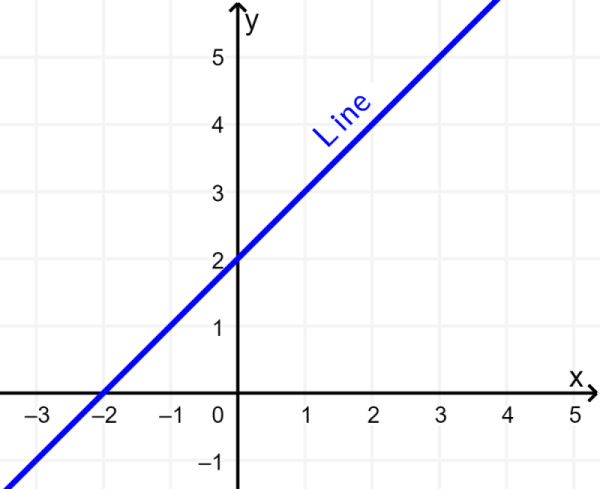
The line we have above represents the equation y = 2x + 1. In this equation:
- the slope is $latex m = 2$.
- the y-intercept is 1.
Slope
The slope of the line is equal to the rate of change of y over the rate of change of x. The slope can be evaluated as follows:
$latex m=\frac{{\left( {{{y}_{2}}-{{y}_{1}}} \right)}}{{\left( {{{x}_{2}}-{{x}_{1}}} \right)}}$
Basically, the slope indicates the inclination of the line in the plane.
Point-intercept form of a linear equation
Another common form for linear equations is the point-slope form. In this form, the equation of the linear equation is formed considering the points in the Cartesian plane:
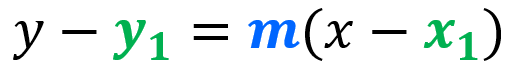
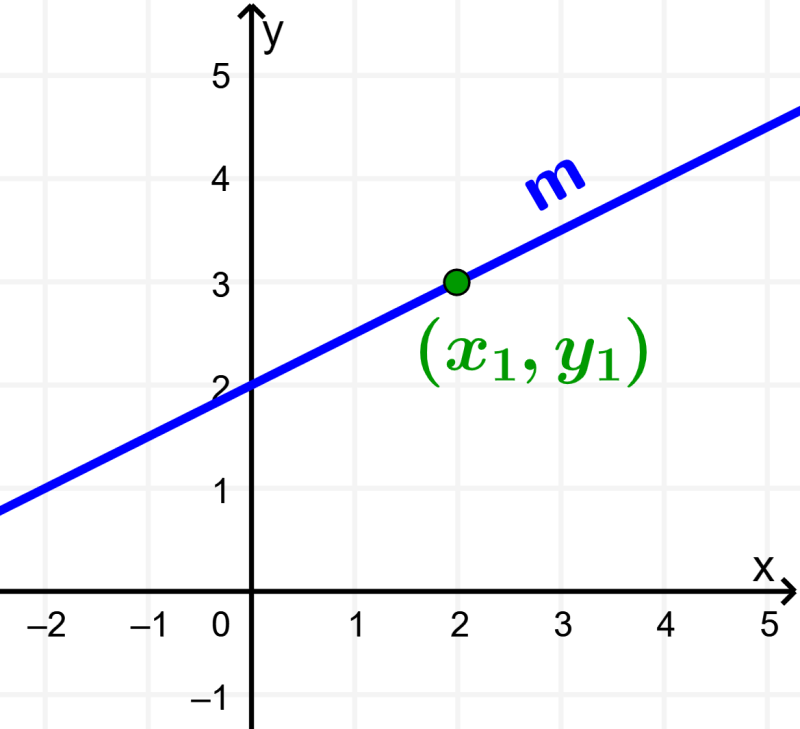
An example of this form is the equation $latex y-3=\frac{1}{2}\left( {x-2} \right)$, where:
- $latex {{y}_{1}}=3$
- $latex {{x}_{1}}=2$
- $latex m=\frac{1}{2}$
Standard form of a linear equation
The standard form of a linear equation with two variables is represented as
$latex Ax + By + C = 0$
where A≠0, B≠0 and x and y are the variables.
EXAMPLES
The equation $latex 2x+4y+5=0$ is in the standard form and we have:
- A=2
- B=4
- C=5
Frequently asked questions
What are linear equations?
Linear equations are the equations used to represent straight lines. The equations for lines in the Cartesian plane are linear equations.
What are the three main forms of linear equations?
The three main forms of linear equations are point-slope form, slope-intercept form, and standard form.
How do we express the standard form of linear equations?
The standard form of linear equations is given by $latex Ax + By + C = 0$.
What is the slope-intercept form of linear equations?
The slope-intercept form is given by $latex y = mx + b$. Where m is the slope and b is the y-intercept.
What is the difference between linear equations and non-linear equations?
A linear equation corresponds to straight lines.
A non-linear equation does not form a straight line. It could be a curve, oscillations or others.
See also
Interested in learning more about equations and systems of equations? Take a look at these pages:
Jefferson Huera Guzman
Jefferson is the lead author and administrator of Neurochispas.com. The interactive Mathematics and Physics content that I have created has helped many students.
